Einstein’s big insight that explained Newton’s law of gravity

One of the most revolutionary scientific laws was first put forth by Isaac Newton way back in the 17th century: the law of universal gravitation. Very simply, it hypothesized that between any and all masses in the Universe, there was an attractive force acting on both of them: the force of gravity. But what were the properties of this force? According to Newton, it had to:

• be completely universal, between all objects with mass,
• be proportional to the mass of each object, multiplied together,
• be instantaneous, and traverse the great distances that separated these objects at infinite, unlimited speed,
• and it also needed to get weaker with increased distance, where it fell off in proportion to the square of the distance separating these objects.

This resulted in what physicists call an inverse square force law: where if the distance separating any two objects is given by r, then the force between those two objects is proportional to ~1/r².

Newton was able to point out many compelling properties of this inverse square law, including that it led to closed, elliptical orbits, allowed all of Kepler’s laws of planetary motion to be derived from it, and that it led to spheroidal planets where objects always fell “down” toward their centers. But one of the big existential questions remained: why was it an inverse square law? Why was the strength of this force proportional to ~1/r², instead of any other possibility? Why was that exponent in the equation “2” rather than any other number?

For hundreds of years, we never knew. Then Einstein came along, and showed it couldn’t be any other way. Here’s why.

Unlike the picture that Newton had of instantaneous forces along the line-of-sight connecting any two masses, Einstein conceived gravity as a warped spacetime fabric, where the individual particles moved through that curved space according to the predictions of general relativity. In Einstein’s picture, gravity is not instantaneous at all, but instead must propagate at a limited speed: the speed of gravity, which is identical to the speed of light.

When Einstein came along, his big problem with Newtonian gravity was that it didn’t make sense in the context of one of his big breakthroughs from 1905: the notion that there was no such thing as “absolute space” or “absolute time,” but only a unified combination of the two, spacetime. If you were to plant two flags at two different locations, and asked yourself the questions of:

• when were these flags each planted,
• and how far apart these two flags are,

you would think — at least, if you were thinking like Newton — that you could just measure both of them conventionally, with a clock or other timekeeping device to determine when each one was planted and with a measuring device, such as a ruler or a tape measure. It shouldn’t matter, in a Newtonian world, where you were located or how fast you were moving, because those signals would be universal, and the same to all observers.

But, as Einstein so convincingly showed in 1905, this is not so. Physical signals can only travel at a finite speed — limited by the speed of light — and both the “when” of the arrival time of signals that correspond to the moment of the planting of the flag, as well as the “how far apart” distance separating the two flags, are dependent on both where an observer is located as well as how fast (and in what direction) that observer is moving.

One revolutionary aspect of relativistic motion, put forth by Einstein but previously built up by Lorentz, FitzGerald, and others, is that rapidly moving objects appear to contract in space and dilate in time. The faster you move relative to someone at rest, the greater your lengths appear to be contracted, while the more time appears to dilate for the outside world. This picture, of relativistic mechanics, replaced the old Newtonian view of classical mechanics, but also carries tremendous implications for theories that aren’t relativistically invariant, like Newtonian gravity.

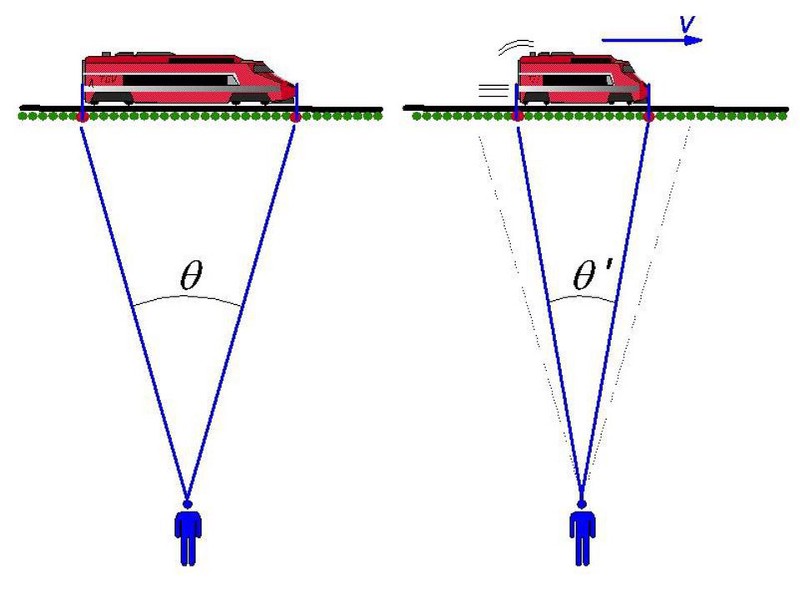
We commonly think of these two phenomena as related to one another: length contraction and time dilation. And they are related, precisely because neither space nor time is the thing that’s invariant and absolute, but rather a quantity known as “the spacetime interval” (sometimes called the Einstein interval), which is a combination of an object’s experience as it moves through both space and time together. In Einstein’s universe, the faster you move through space relative to someone who’s stationary, the slower you move through time, and conversely, the slower you move through space, the more rapidly you move through time.

Something that moves at the maximum speed allowable — the speed of light — is especially interesting, because it moves through space at the maximum possible rate. It’s as though time becomes effectively “frozen” for any object that moves at the speed of light, even though, to an outside observer, its speed and motion is still finite: at 299,792,458 m/s. This, too, is something that all observers can agree upon in Einstein’s universe: that the speed of light appears to be constant to everyone. Whether you’re at rest or whether you’re in motion, light always moves at this speed. Your experience of the passage of time or of the separation distance between objects (or even the physical size of objects) might be different dependent on your motion or your location, but the speed of light is not.

A “light clock” will appear to run differently for observers moving at different relative speeds, but this is due to the constancy of the speed of light. Einstein’s law of special relativity governs how these time and distance transformations take place between different observers. However, each individual observer will see time pass at the same rate as long as they remain in their own reference frame: one second-per-second, even though when they bring their clocks together after the experiment, they’ll find that they no longer agree.

This leads us to pose a very interesting question, or “thought-experiment” as Einstein was fond of calling it. We know that the Sun gravitationally attracts the Earth, and that the Earth orbits the Sun due to the Sun’s gravitational influence. We also know that the Earth receives sunlight because the Sun generates that light, and that light then traverses the space between the Sun and Earth.

We also know that the light we see, right now, does not correspond to the light being emitted right now, at this very instant, from the surface of the Sun. Instead, the Sun, from our perspective here on Earth, is located about 150 million km (93 million miles) from the Earth, and so it takes time — about 8 minutes and 20 seconds — for that light to travel through that space, and to journey from the Sun to the Earth, where we can receive and absorb it.

If the Sun were to somehow cease shining, as though it instantaneously went dark, we wouldn’t know about it instantaneously. It would take time — that same 8 minutes and 20 seconds — for the light that was already emitted and that had already begun its journey from the Sun to the Earth (including the light that was already en route) to finish arriving. Only after the last of that light arrived would the Sun appear to go dark to an observer here on planet Earth.

When humans observe the Sun, we are only observing the light that was generated within it and released from the Sun’s photosphere approximately 8 minutes and 20 seconds ago. It takes roughly that amount of time for light to propagate across the 150 million km (93 million miles) separating the Sun from the Earth before we can “see” it. If the Sun suddenly went dark, we wouldn’t observe that darkness for another 8 minutes and 20 seconds.

Well, what if we could somehow imagine the same thing, but instead imagining what would happen to the Sun’s light if it suddenly turned “off” somehow, what would happen to the Sun’s gravitational effects? What would happen if you could somehow imagine “disappearing” the Sun from spacetime itself, and removing that enormous amount of mass — all two nonillion (2 × 10³⁰) kilograms of it — from its present location in space?

How would this removal of the Sun itself affect the Earth, and when would Earth feel those effects?

If we were considering a Newtonian picture of the Universe, the answer would be “instantaneously,” as the very moment the Sun were winked out of existence, the gravitational force between the Sun and Earth — as well as the Sun and every object — would cease to exist. Planets from Mercury out through Neptune would immediately fly off in a straight line, no longer bound by the Sun’s gravity. But Einstein realized this could not be so.

Because there was no way for a signal to traverse these distances instantaneously, and because there was no “action at a distance” in general, the gravitational signal induced by the curved space caused by the Sun’s presence could only propagate at a finite speed — the speed of light — and so it must take that same amount of time, roughly 8 minutes and 20 seconds, for the Earth to cease feeling the gravitational effects of the Sun.

An animated look at how spacetime responds as a mass moves through it helps showcase exactly how, qualitatively, it isn’t merely a sheet of fabric. Instead, all of 3D space itself gets curved by the presence and properties of the matter and energy within the Universe. Space doesn’t “change shape” instantaneously, everywhere, but is rather limited by the speed at which gravity can propagate through it: at the speed of light. The theory of general relativity is relativistically invariant, as are quantum field theories, which means that even though different observers don’t agree on what they measure, all of their measurements are consistent when transformed correctly.

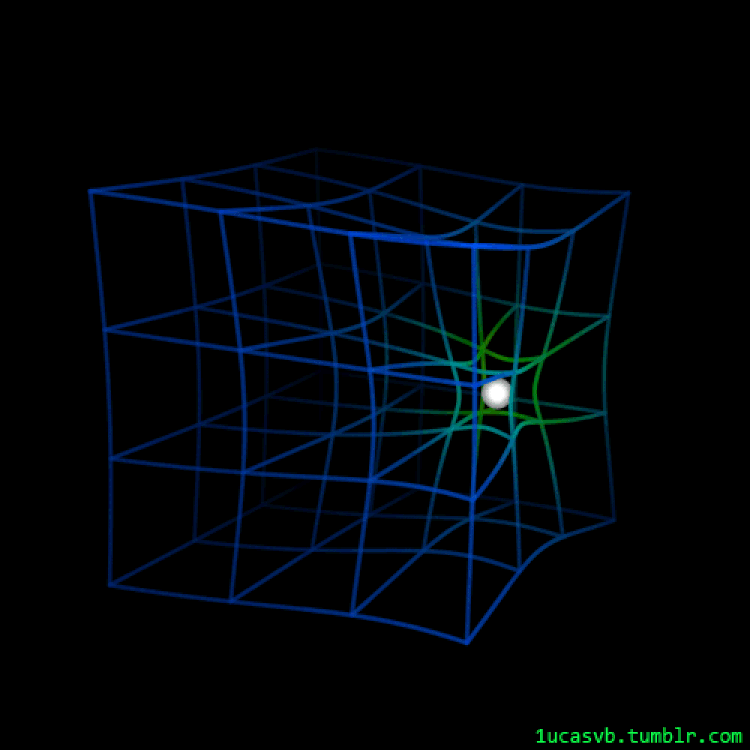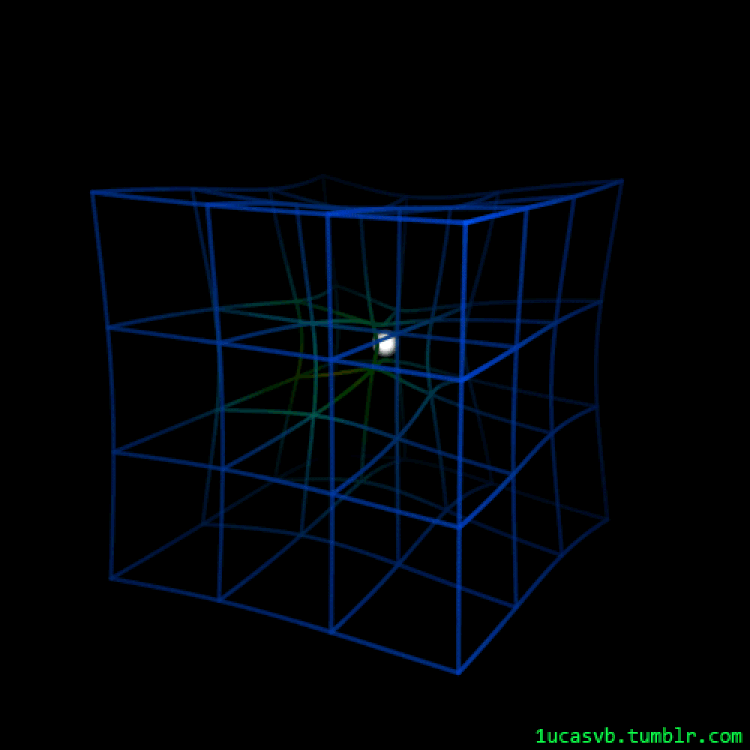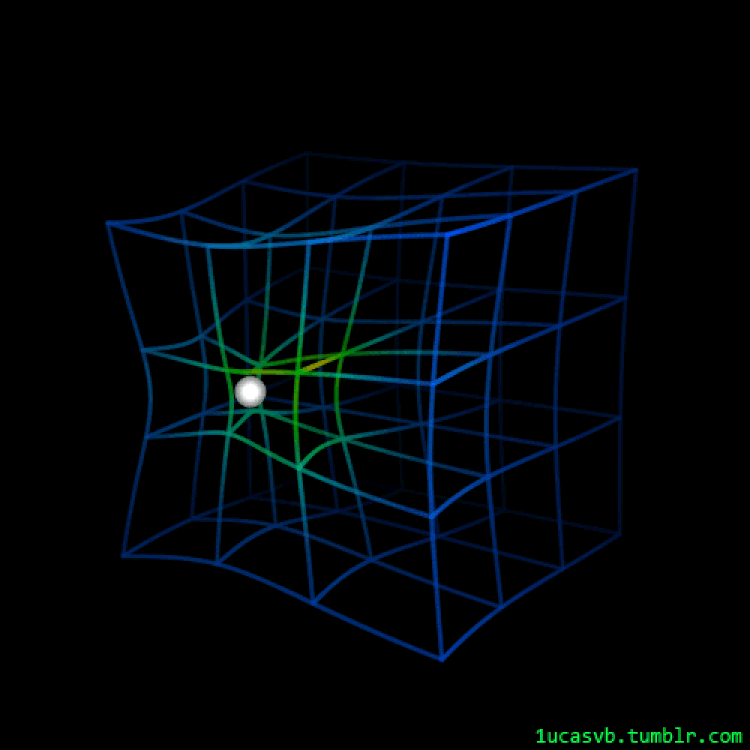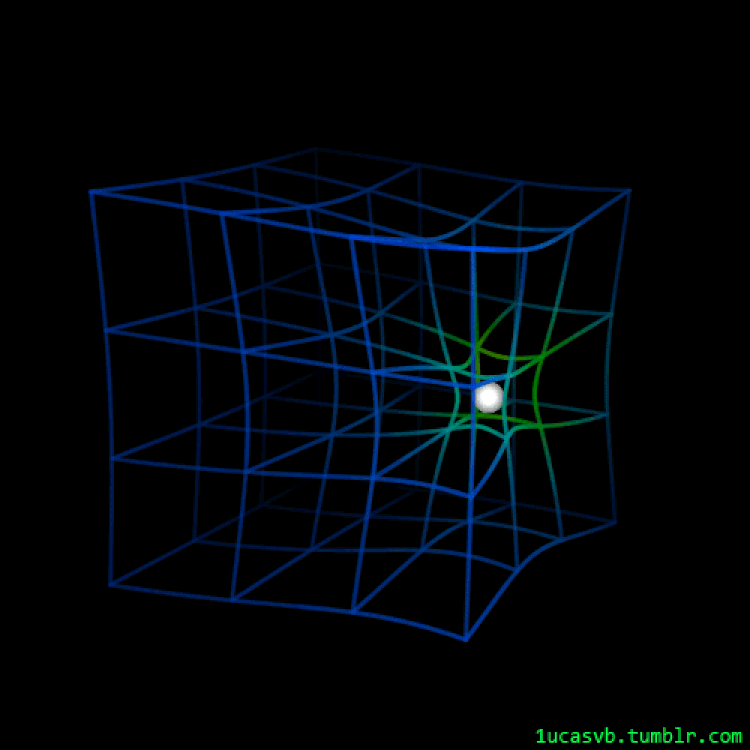
The answer to this question, all on its own, was enough to convince Einstein that the gravitational force between any two separate masses must obey a ~1/r² law, as any other answer would be unphysical.

The explanation is straightforward if you think about it. First, think about the Sun: a large, hot, light-emitting sphere of material. As light emanates from the Sun’s photosphere (its outermost layers), it doesn’t just propagate through space; it also spreads out as it departs from the source. The Sun is a sphere, and the light from it propagates spherically outward: in all three dimensions.

As this light spreads out in a sphere, we have to understand that it’s not filling the sphere with light, which would be a three-dimensional volume, but rather that it moves outward in a spherical shell: over a two-dimensional area, like the surface of a globe. As it propagates farther away from the Sun, the light you receive over any given amount of area decreases, as the surface area of a sphere is given by 4πr². A planet that’s twice as far from the Sun as another receives only one-fourth of the light per unit area; a planet that’s three times as distant receives only one-ninth of the light per unit area.

The way that light spreads out as a function of distance means that the farther away from a power source you are, the energy that you intercept drops off as one over the distance squared. This also illustrates, if you view a certain specific angular area (illustrated by the squares) from the perspective of the original source, how larger objects at greater distances will appear to take up the same angular size in the sky. Each time you double your distance between a source and observer, the brightness you observe gets quartered. In general, photons (and all light) propagate spherically outward away from the emitting source.

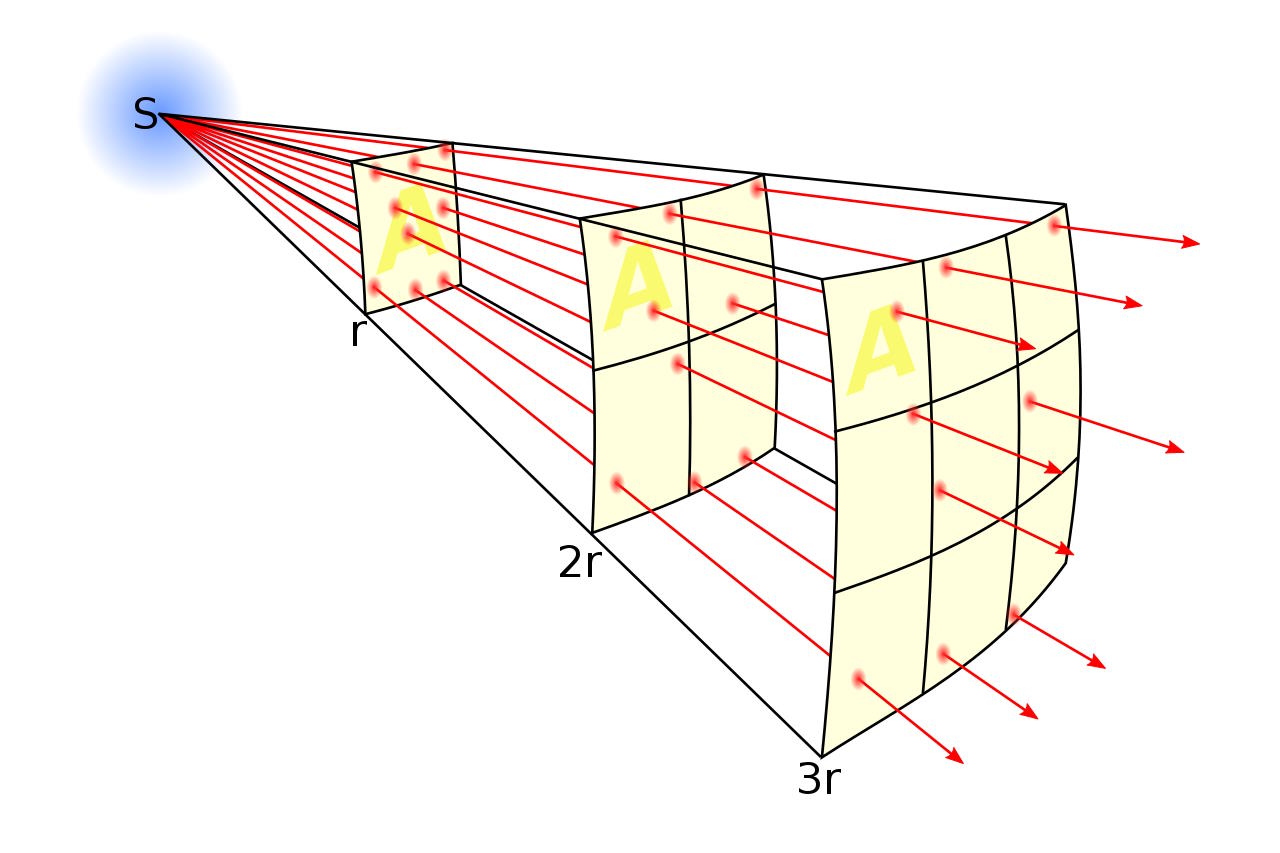
As a result, the brightness that an object appears to shine at falls off three-dimensionally: as ~1/r².

We can imagine a scenario where things didn’t propagate through three-dimensional space, but rather where they propagated through a different number of dimensions. Take, for example, the scenario of dropping a stone into a very still pond of water. Yes, there will be a large initial “splash” in the middle of the pond, where the stone lands, but after the stone strikes the water’s surface, a wave will propagate outward in the shape of a circle, moving across the surface of the water and spreading out not in three dimensions, but only in two.

As a result, if you were to measure the height of the crest of the wave at several points along the water, as you moved away from the impact location of the stone, you’d find that:

• when you’re a certain initial distance away, you measure a certain initial height,
• when you’re twice that initial distance away, the height is one-half of the initial height,
• when you’re three times the initial distance away, the wave’s crest is one-third of the initial height,
• when you’re four times the initial distance away, the wave’s crest is one-fourth of the initial height,

and so on. Because the wave can only propagate along the surface of the water — a two-dimensional surface — it only spreads out proportionally to ~1/r, since the circumference of a circle is given by C = 2πr.

When a disturbance is created in a pond, such as by dropping a stone into an otherwise still body of water, it will generate ripples that propagate circularly outward. Because the waves are confined to the surface of the water, their amplitude will only decrease as ~1/r, as the waves spread out in a circle, confined to a two-dimensional surface.

Einstein’s general relativity is a four-dimensional theory: where one dimension is a time dimension and the other three are spatial dimensions, describing the length, width, and depth (or x, y, and z-directions) we experience. Just as light emitted by the Sun must spread out in three dimensions, but at any instant, that light is confined to the surface of a sphere, so too must the gravitational effects exerted by the Sun: it affects space three-dimensionally, but gets weaker the same way that the surface area of a sphere grows: according to the formula for the area of a sphere’s surface, or 4πr².

That leads to a force law where gravity gets weaker in precisely the same way: as though it’s proportional to ~1/r². The reason gravity follows a ~1/r² force law is because we live in a Universe with three spatial dimensions, and as gravity propagates through all three dimensions away from whatever source generated it, it spreads out in all three dimensions. This makes sense, if you think about it.

• Things that propagate in one-dimension, like a compression wave through a slinky, don’t spread out, and so the wave doesn’t weaken, but rather stays constant: proportional to ~1/r⁰.
• Things that propagate in two-dimensions, like ripples arising from a stone dropped into a pond, spread out like circles, so the height of those ripples fall off proportionally to ~1/r.
• And things that propagate in three-dimensions, like light or gravity traveling through space, spread out like spheres, so their intensity falls off proportionally to ~1/r².

This illustration shows the results of numerical simulations of the gravitational waves emitted by the inspiral and merger of two black holes. The colored contours around each black hole represent the amplitude of the gravitational radiation; the blue lines represent the orbits of the black holes and the green arrows represent their spins. Even though the two objects orbit one another in the plane, gravitational waves propagate outward in three-dimensions, and the gravitational force falls off as ~1/r².

You might then think to ask the question, “What if there are additional spatial dimensions out there beyond the three that we know of?” You might have had the thought that any extra dimensions would provide an extra direction — or degree of freedom — for a physical phenomenon to propagate through. Believe it or not, this thought is correct!

• If the Universe had four spatial dimensions instead of three, both light’s intensity and the strength of the gravitational force would spread out in four dimensions now, and their intensity or strength would fall off proportionally to ~1/r³.
• If the Universe had nine spatial dimensions (as in superstring theory), the gravitational force would fall off proportionally to ~1/r⁸.
• If, instead, it had ten spatial dimensions (M-theory), it would fall off ~1/r⁹, while in bosonic string theory, with 25 spatial dimensions, it would fall off proportionally to ~1/r²⁴.

The fact that we can test the behavior of the gravitational force on a wide variety of scales — from cosmic scales to galactic ones down to Solar System scales, all the way down to terrestrial and even microscopic laboratory scales — has confirmed that, everywhere we’ve been able to probe, our Universe only behaves as though there are three spatial dimensions: no more and no fewer.

This image, of an optically levitated microsphere in a vacuum, provides a laboratory for testing gravitation and the nature of the inverse-square force law down to ~micron scales. Despite the variety of enormously precise experiments, no deviations, which could indicate the presence of extra dimensions, have ever been found. The ~1/r² nature of the gravitational force holds down to the smallest scales we’ve ever probed.

It remains a possibility that somewhere way down there, perhaps on subatomic scales, there truly are extra spatial dimensions, and thus, extra degrees of freedom in precisely this fashion. If there are extra dimensions, experiments that probe:

• the gravitational force,
• the way that light’s intensity changes,
• or the strength of the electromagnetic force,

should be able to reveal their presence by observing a departure from the conventional, three-dimensional predictions that have proven successful time and time again on all scales.

It was through a series of successful realizations that Einstein, at long last, was able to explain the inverse square (i.e., ~1/r²) force law of gravity. If space and time weren’t absolute and gravitation didn’t propagate instantaneously, then gravity had to travel at a finite speed and propagate through space in order to interact with objects in its vicinity. And since signals that travel through space spread out within that space, then the number of dimensions within our Universe would determine how the gravitational force behaved with distance, where a three-dimensional Universe implied exactly a ~1/r² force law.

This showed the impossibility of a “fractional exponent” to the gravitational force, as suggested by Einstein’s contemporaries Simon Newcomb and Asaph Hall, and relegated the existence of any “extra” dimensions to tiny, microscopic scales where they would not ruin laboratory or terrestrial experiments that validated the ~1/r² nature of gravity. Newton might have been the first to figure out the law of gravity, but it wasn’t until Einstein that we truly understood why it couldn’t be any other way.