Ask Ethan: Why are planets always round?

- In our Solar System, all of the planets, many moons and smaller objects, and the Sun are all round.
- Above a size of approximately ~400 kilometers in radius, practically all rocky bodies are round; above ~200 kilometers in radius, most icy bodies are, too.
- There are no irregular objects out of hydrostatic equilibrium above a certain size, and physics can explain why.
For more than 2,000 years, humanity has known that our planet, Earth, is round in shape. Just as the Moon and Sun appear round, so does not only Earth, but every planet in our Solar System. Even non-planets get in on the “round” action as well. Earth’s moon, Jupiter’s four largest moons, four of Saturn’s five largest, Uranus’s five largest and Neptune’s largest moons are all round, as well as the asteroid Ceres and numerous Kuiper belt and Oort cloud objects. Some smaller objects as little as ~200 km in radius are round, while Neptune’s Proteus and Saturn’s Iapetus, significantly larger, are not. Why is this? Why are other shapes not possible for the largest objects of all? That’s the question of Sgt. Randy Pennington, who wrote in:
“[Someone] asked me, ‘okay, so we’ve gone to space and traveled throughout the Solar System, and every planet that we’ve measured is round. But why?’ And I knew the planets were round, but I don’t know why. What would happen if a planet were shaped like a cube, or a pyramid, and why aren’t there any? But I know someone who’ll know… so why, Ethan, why are all the planets always round?”
It’s true: Every planet is round, and some are even rounder than others. Moreover, the stars are also always round, many moons and even some asteroids and Kuiper belt objects are round. Here’s the science of what’s going on.

The first thing to recognize is that normal matter can clump together in any amount. Individual atoms and even subatomic particles, like atomic nuclei or free electrons, exist in great abundances in stellar systems, as well as in interstellar space. Atoms also link up to form molecules, which can exist freely or as parts of other systems, and molecules themselves can clump together in amounts both great and small.
While there are nuclear and electromagnetic forces at play, both of which can easily overwhelm any other forces, when you get large amounts of mass together, it’s actually the weakest force of all that wins: gravity. If you get enough normal matter together in one place — regardless of the type, phase, origin, or nature of matter that you have — it will contract until it’s a single, gravitationally bound object.
When these objects are small, they tend to form minuscule, dustball-like structures. These grain-like particles aren’t actually held together via gravity, but rather via electrostatic forces. Simply bringing them close to the Sun, where they’re exposed to things like solar radiation and the solar wind, is enough to destroy them. If you want something more robust, you have to look to larger masses, enabling the force of gravity to become more dominant.

Take the above-pictured asteroid, for example: Itokawa. Itokawa is large enough to be its own gravitationally bound structure, weighing in at about ~30 million tons. It’s only a few hundred meters across on a side, but that’s enough to illustrate, at least at this scale, what gravitation can and cannot do. When you’ve accumulated more than a “grain” of matter but not more than a few million tons, here’s what you wind up with.
- A “rubble pile” body. Instead of being one solid object, you get what looks like a collection of many different grains and pebbles, all held together through their mutual gravitation.
- An object that isn’t differentiated. If you have a lot of mass together, you get a differentiation of your layers, where the densest materials sink to the center, forming a core, while the less-dense materials like a mantle or crust “float” atop them. Itokawa, and other objects of comparable masses and sizes, can’t do that.
- A composition that shows the merger of different bodies. This one isn’t necessary, but it does happen frequently, and Itokawa is a spectacular example of it: the two portions of the “peanut” that composes Itokawa have dramatically different densities, indicating that these were once two separate objects that have now, gravitationally, merged together.
All told, these objects can hold themselves together gravitationally, but aren’t round.

Why don’t these small objects become round? It’s because the forces between atoms and molecules — governed by electrons and the electromagnetic force — are stronger than the force of gravity at this scale. Gravitation is always attractive, and pulls every particle of matter toward the center of mass of the objects of which they’re part. But there are also forces between atoms and molecules that determine their shape and configuration.
Ice crystals form in lattices; silicate rocks can form amorphously; dust particles can get compacted into soils or even solid shapes; etc. When a gravitational force is applied to a large body or collection of bodies, it exerts a pressure: a force over an area. If the pressure is great enough, it will override whatever initial conditions or shapes an object possesses to start with, and compel it to reshape itself into a more energetically stable configuration.
In the case of self-gravitating bodies, overcoming whatever random initial shape and configuration you start with is the first obstacle you face, and just how much mass is required depends on what your object is made out of. You can form a cube, a pyramid, or whatever potato-esque shape nature can dream up, but if you’re too massive, and the force of gravity is too large, you won’t maintain it, and will instead be pulled into a round shape.
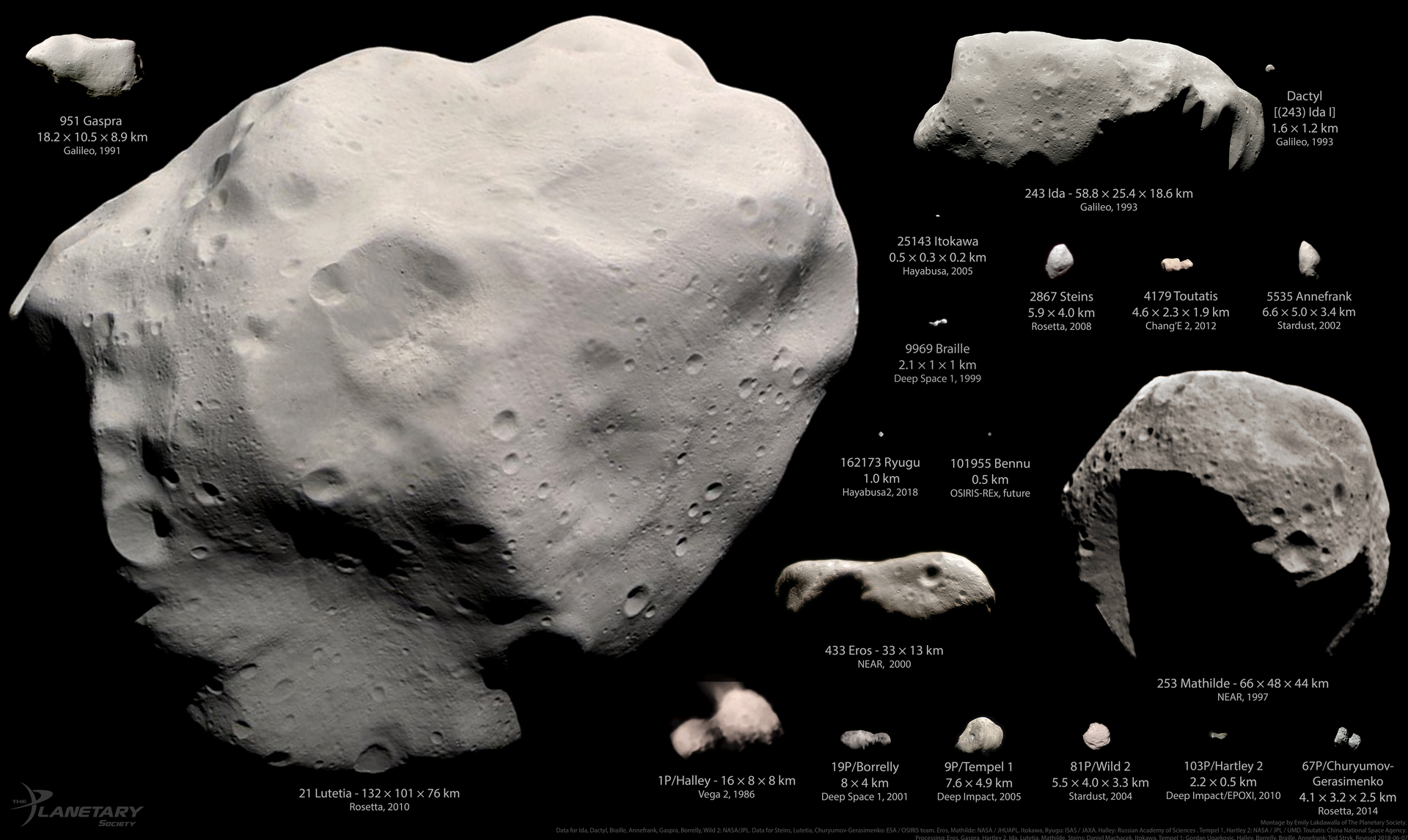
If you’re below about 1018 kilograms (a quadrillion tons or so), you’ll be below about 100 kilometers in radius, and that is always too small, or low in mass, to pull yourself into a round shape. Itokawa falls short of this threshold by a factor of many millions, as do most of the known asteroids.
However, if you can accumulate enough material to rise above this mass and size threshold, you’ve got a chance at rough “roundness.”
Saturn’s moon Mimas, for example, is slightly under 200 kilometers in radius, but is undoubtedly rounded. In fact, it’s the smallest astronomical body presently known that’s in a round shape owing to self-gravitation, and is the innermost large moon of Saturn, completing an orbit around the ringed planet in under 24 hours. Mimas is very low in density, only barely denser than water-ice, suggesting that it’s made up largely of volatiles: low-density ices that are easy to deform under the force of gravity.
Were Mimas composed largely of rocks or even metals, it would have to be larger and more massive to self-gravitate into a sphere: as large as 400 or 500 kilometers in radius, in the most extreme cases.

Round, however, is only part of the story. You can still have large features that lead your object to depart from the shape that self-gravitation would otherwise lead to on a world that becomes rounded. Mimas, in fact, demonstrates this, with its Death Star-like appearance owing to its enormous crater: so large it’s almost a third of Mimas’s diameter. The crater walls are over 5 km high and the crater floor is more than 10 km deep; in fact the surface on the opposite side of Mimas from this crater is highly disrupted. The impact that created this crater must have nearly destroyed Mimas entirely, and its gravitation is insufficient to pull it back into a more spherical shape.
This example illustrates an important distinction: the difference between being “round” and being in “hydrostatic equilibrium.” Self-gravitation can pull you into a round shape easily if you’re over 200 kilometers in radius and icy or over 400 kilometers in radius and rocky. But being in hydrostatic equilibrium is a more difficult bar to clear: you have to have your shape primarily determined by a combination of self-gravitation and rotation: the same shape a self-gravitating drop of spinning liquid water would take on.

The smallest body verified to be in hydrostatic equilibrium is the largest asteroid: the dwarf planet Ceres, with a radius of about 470 kilometers. On the other hand, the largest body known not to be in hydrostatic equilibrium is Saturn’s bizarre moon Iapetus, with a radius of around 735 km, whose planet-spanning equatorial ridge would never occur if gravity and rotation alone determined its shape.
For a solid body like a rocky planet or moon, the big question is whether your gravity can make you behave in a plastic fashion. In physics and materials science, plastic doesn’t mean “made out of the by-products of oil,” but rather describes how certain materials deform. When you subject a material to stresses arising from tension, compression, bending, or torsion, those materials will normally elongate, compress, buckle, twist, or otherwise deform.
If your material deforms plastically, those distortions and deformations can become permanent. If you have enough mass together in one place, gravitation will be sufficient to pull you back into hydrostatic equilibrium, so that your overall shape is once again determined by your rotation and gravity alone. If not, you can still be round, but not in hydrostatic equilibrium.

For icy objects, you can be round at about 200 kilometers, but you won’t be in hydrostatic equilibrium until you’re about 400 kilometers in radius. For rocky objects, you won’t be round unless your radius is about 400 kilometers, but you might not reach hydrostatic equilibrium unless your radius is greater: up to 750 kilometers may be needed.
Objects that live in that in-between region could either be in hydrostatic equilibrium or not, and we’re not certain about the status of many of the known ones. Rock-and-ice Hygeia, with a radius of only 215 km, might be in hydrostatic equilibrium. Saturn’s moon Enceladus, at 252 kilometers, is close, but the asteroids Pallas and Vesta, at 256 and 263 km, severely depart from even being round. Pluto’s large moon Charon, with a radius of 606 km, might not have quite achieved hydrostatic equilibrium. The largest two Uranian moons, Titania and Oberon are probably in hydrostatic equilibrium; the next three, Umbriel, Ariel, and Miranda, may or may not be.
However, once you get up to about 800 kilometers in radius, everything known above that size is not only round, it’s in hydrostatic equilibrium as well.
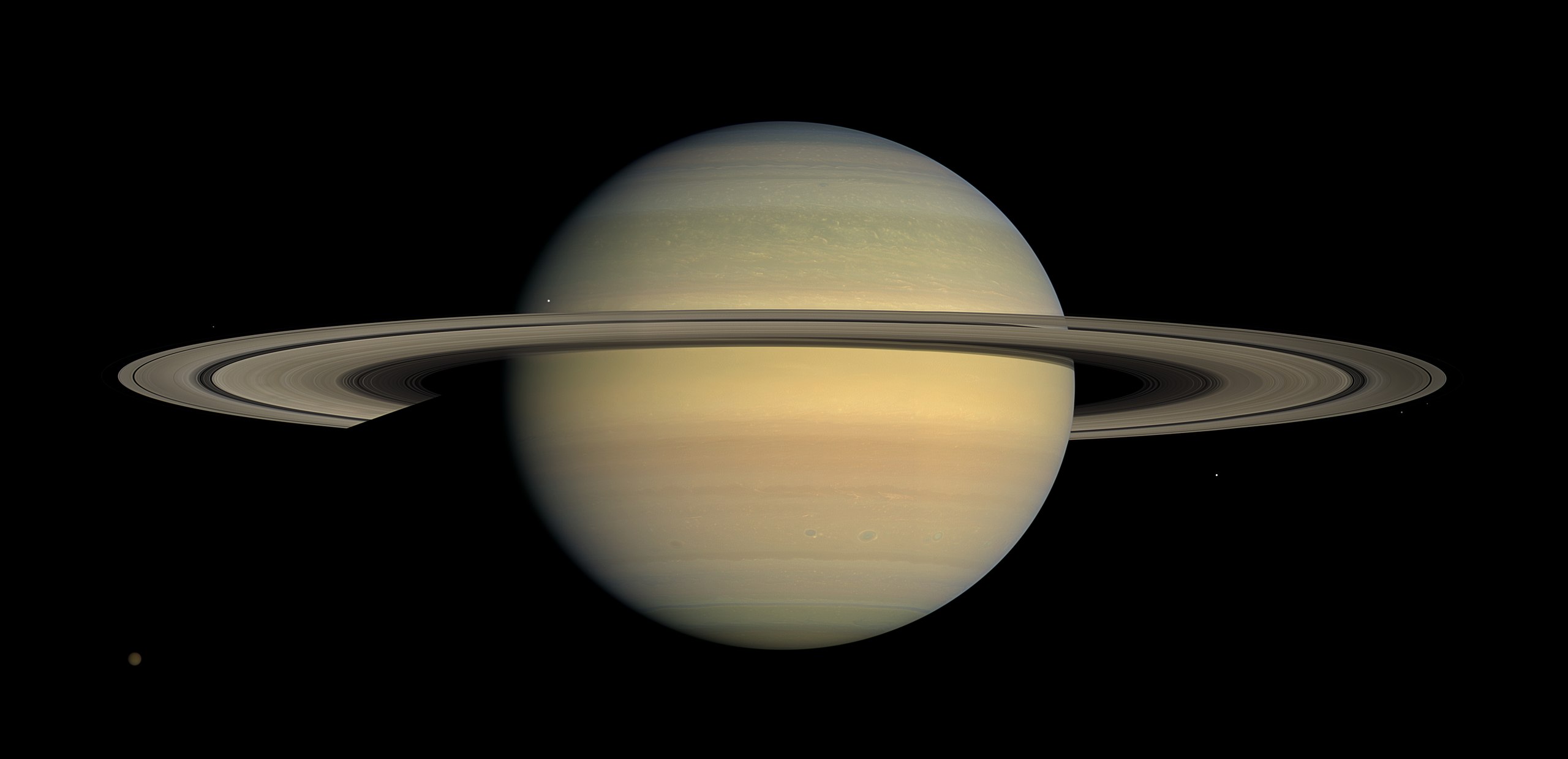
The dwarf planets Haumea, Eris, and Pluto (along with Makemake, at only 715 km in radius) are all in hydrostatic equilibrium. Neptune’s Triton, Earth’s Moon, Saturn’s Titan, and the four Galilean moons of Jupiter are all in hydrostatic equilibrium as well. So are all eight of the planets, and so is the Sun. In fact, we’re pretty confident that this is a universal rule: if you’re more than about 800 kilometers in radius, regardless of your composition, you’re going to be in hydrostatic equilibrium.
But here’s a fun fact: Many objects — including many planets and stars — rotate so quickly that it’s very clear that they aren’t round, but rather take on a squashed shape known as an oblate spheroid. Earth, due to its 24 hour rotation, isn’t quite a perfect sphere, but has a larger equatorial radius (6378 km) than a polar radius (6356 km). Saturn’s rotation is even faster, completing a rotation in just 10.7 hours, and its equatorial radius (60,268 km) is almost one full “Earth” larger than its polar radius (54,364 km).
The Moon and Mercury, however, are both incredibly slow rotators. They’re only ~2 km larger in radius in the equatorial direction than the polar one, making them very spherical rocky planets. But do you know which body is the most perfect sphere in the Solar System? The Sun. With an average radius of 696,000 kilometers, its equatorial radius is only ~5 km larger than its polar radius, making it a perfect sphere to 99.9993% precision.
Although there are many factors at play in determining the shape of an object, there are really only three main categories that bodies fall into.
- If you’re too low in mass and/or too small for your composition, you’ll simply take on whatever shape you happened to have by the luck-of-the draw in forming; practically all objects below ~200 kilometers in radius have this property.
- If you’re more massive, that initial shape will get reconfigured into a round one, a threshold that you cross between ~200 and 800 km in radius, depending on your composition. However, if a major distortive event happens, like an impact, a deposition, or a change to your orbital properties, you will likely keep an imprinted “memory” of that event.
- Finally, above ~800 kilometers in radius, you’ll be in hydrostatic equilibrium: massive enough so that gravity and rotation primarily determine your shape, with only small imperfections superimposed atop that.
In terms of mass, 0.1% of Earth’s mass will do it; bring that much together and you’ll always be in hydrostatic equilibrium. Roundness, on its own, isn’t quite enough to make you a planet, but all planets have more than enough mass to pull themselves into a round shape. The irresistible force of gravity is enough to ensure it couldn’t be any other way.
Send in your Ask Ethan questions to startswithabang at gmail dot com!





