It’s important that weight and mass are not the same

If you ask someone how much they weigh, they’re likely to give you a number that they obtained from reading it off of a scale. If that person uses the metric system, they’re likely to give you a measurement in kilograms: 93 kilograms, for example. If they use the English system of measurement, however, they’re likely to give you your answer in pounds, where the equivalent of 93 kilograms is 205 pounds. Conventionally, here on the surface of the Earth, we can convert between the two using only a minimal amount of effort: 1 kilogram is 2.205 pounds, and vice versa, 2.205 pounds converts to 1 kilogram. Going back-and-forth requires only multiplication or division, which seems easy enough.

But “kilograms” and “pounds” aren’t just what you weigh in different systems of measurement units; they’re fundamentally different quantities from one another. A kilogram is an example of mass, not of weight, while a pound is an example of a weight, not of a mass. It’s only here on the surface of the Earth, where we’re at rest relative to the rotating Earth, that these two concepts can rightfully be used interchangeably. It wasn’t until Isaac Newton came along that the difference between weight and mass was first generally appreciated, and even physics teachers (and physics textbooks) get confused about how these two concepts are not identical. Here’s why this simple-seeming fact, that weight and mass are different, is truly profound.

According to legend, the first experiment to show that all objects fell at the same rate, irrespective of mass, was performed by Galileo Galilei atop the Leaning Tower of Pisa. Any two objects dropped in a gravitational field, in the absence of (or neglecting) air resistance, will accelerate down to the ground at the same rate, and will traverse an amount of distance that’s proportional to the time it’s in free-fall squared. The ball does not “separate” from the tower, either, which some (incorrectly) expected would have been the case if the Earth rotates.

The earliest experiments we performed that gave us an insight into gravity and motion were done right here on Earth: by dropping objects in free-fall and rolling them down ramps. If you release an object from rest and allow it to fall, it falls straight down, accelerating at a constant rate. It gains speed directly proportional to the amount of time that it’s been falling, and the distance it covers is proportional to the amount of time squared that the object has been falling. For objects rolled down a ramp, the proportionality is exactly the same, but the overall acceleration — and hence, the gains in speed and changes in distance — are lower compared to the objects in free-fall.

This phenomenon, however, appears to not depend on mass or weight. A light object will fall just as quickly as a heavy object, especially if air resistance isn’t a factor. A lead pebble and a lead cannonball will fall and accelerate at the same rate, while the same lead pebble and cannonball will roll down a ramp in identical amounts of time. However, the larger cannonball has both more mass and more weight than the smaller pebble. You can test this out by using a scale: a device that either puts masses on one end of a balance until it cancels out your own mass, or a device that directly measures the force exerted through your feet (or whatever contacts the device) onto the scale itself.

The idea behind the “scales of justice” is that the weight of the strongest arguments will tip the scales in favor of the more just side. Unlike a conventional scale, this two-sided balance will allow you to determine the correct mass for an object under all environmental conditions except for perfect weightlessness.

These two types of scales, interestingly enough, make fundamentally different measurements. There’s a simple device you can use to test this out for yourself: an elevator. If you balance the two sides of a scale — something like the famous “scales of justice” that place two sets of masses on either side of a balance point — then that scale will remain balanced. This remains true whether the elevator:

• is at rest on the surface of the Earth,
• accelerates upward,
• accelerates downward,
• or is at rest on any other planet.

The same amount of mass that balances the scales on Earth will also balance the scales under any other acceleration you can imagine. This is because the downward force of gravity, acting on the mass on one side of the scale, balances the downward force of gravity acting on the (equivalent) mass on the other side of the scale.

But if you have a scale that works by measuring the force it uses to push up against your feet (and keep you from falling through the scale), you’ll find that those same scenarios, where the elevator:

• is at rest on the surface of the Earth,
• accelerates upward,
• accelerates downward,
• or is at rest on any other planet,

yields different answers for each situation. Only when you’re at rest on the surface of the Earth will the scale give the same reading you saw using the other type of scale.

This scale, from more than 100 years ago, balanced out the downward force of gravity exerted by the objects atop the scale with an internal spring. The amount that the spring compresses is dependent on the downward force from the weights above it. If either the mass or the acceleration of the above weights change, so will the reading of the scale.

Why does your weight change in the second set of examples, where you use a force-based scale, but not in the first set of examples, where you use a set of counterweights for your scale?

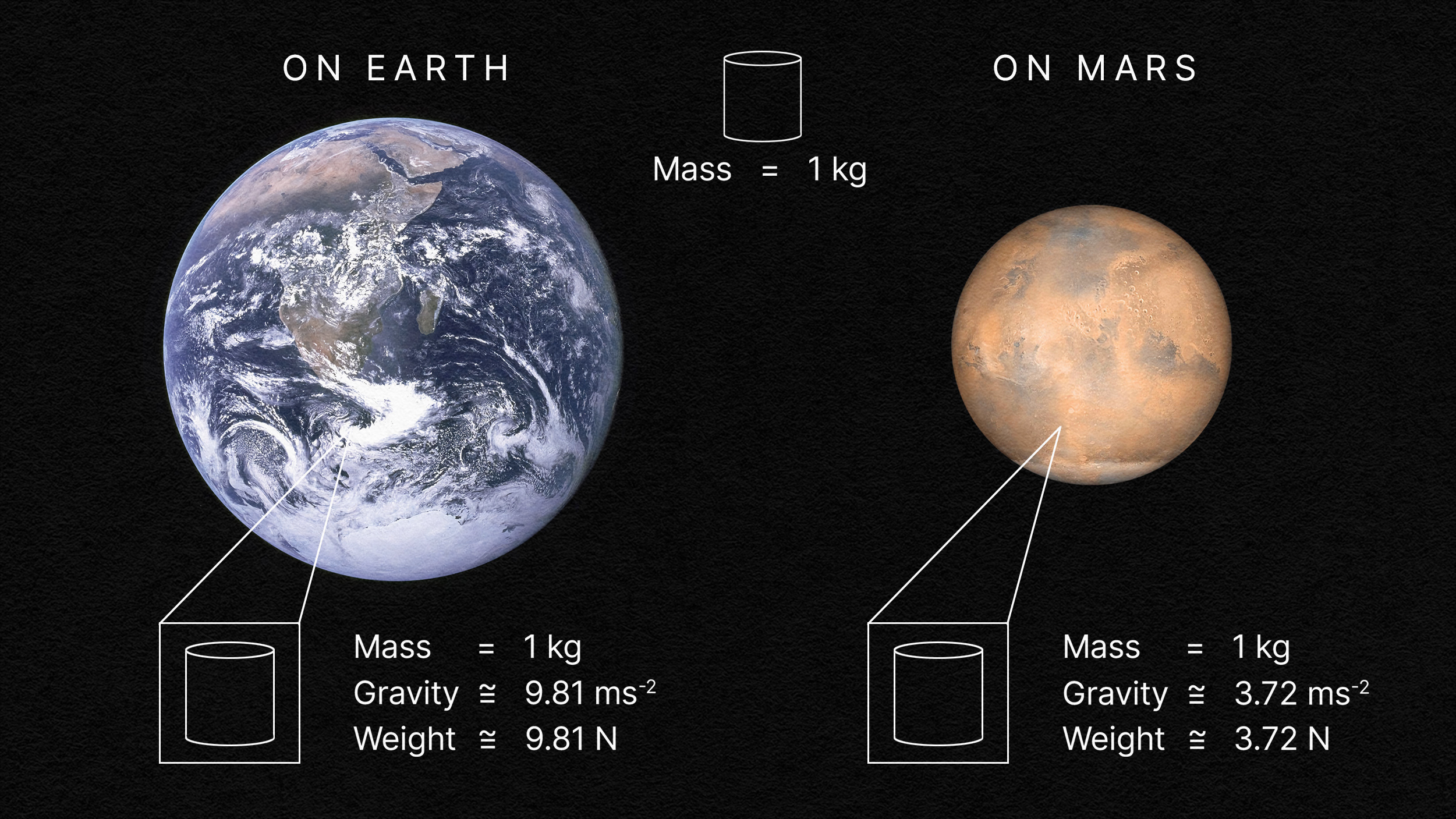
Because in the second set of examples, you’re measuring weight directly: through the force the scale must exert on you. Weight itself is just one example of a type of force, where force obeys Newton’s most famous law: F = ma. If you are at rest, then gravity is trying to accelerate you downward with a specific force: where the acceleration is due to Earth’s gravity at its surface. We call this acceleration g, where g points toward the center of the Earth with a value of about 9.8 meters-per-second-per-second. The scale, in order to keep you from falling through the scale itself, must push back with an equal-and-opposite force: a force that balances your weight. This leads to the conventional definition of weight on the surface of the Earth, your mass multiplied by g, or 9.8 m/s².

If you stand on that same scale, however, and you happen to be in an elevator that’s accelerating you upward at the moment, such as right after you’ve pressed the button for the top floor and the door closes, you don’t just have the acceleration of Earth’s gravity to contend with. You also, on top of that, have the upward acceleration of the elevator. In order to keep you accelerating upward along with the elevator, the scale that you’re on doesn’t just need to oppose your weight, but also to apply the additional force that will accelerate you upward. Now, the scale doesn’t just read “mg” for your weight, but “ma,” where a is equal to g plus your extra upward acceleration.

When you stand on a scale at rest on Earth’s surface, it will give you a reading of your weight that equals your mass multiplied by the acceleration at Earth’s surface. If you’re in an elevator that’s either accelerating upward or downward, however, your weight on this type of scale will appear to change: increasing while you accelerate upward and decreasing while you accelerate downward.

When you accelerate downward, the opposite is true; the scale reads less than your weight at rest on Earth, because your acceleration is equal to g minus your extra downward acceleration. And on a different planet, where the acceleration due to gravity is different, the scale will indeed read “mg” just as it does on Earth, but the g will be specific to the surface of that planet, which may well be very different to what it is on Earth. (g on Mars is about 38% of what it is on Earth; whereas g on Jupiter, if the surface were solid rather than gaseous, would be about 254% of what it is on Earth.) In all of these scenarios, your weight is different from what it is when you’re stationary on Earth’s surface. However, in each one, your mass remains unchanged.

Why does this type of scale, which measures the force your feet make with the scale beneath them, give such varying readings, whereas the “other” type of scale — a balance scale — gives a consistent reading across all of these conditions?

Because on the balance scale, it’s the mass on the opposite end of the scale from you that’s balancing the force your mass creates. If the acceleration on you is due to Earth’s gravity, so is the acceleration on that nearby mass. If it’s due to Earth’s gravity plus or minus an additional acceleration, so is the acceleration on the nearby mass. If it’s due to the gravity on a different planet, so is the acceleration on the nearby mass. In other words, as the force on your body changes, even if it’s because the acceleration on your body changes, the force on this opposing mass changes in the same exact fashion.

This type of scale will only balance if the amount of weight on one side of the scale is equivalent to the amount of weight on the opposite side of the scale. You can be certain of getting 1 kilogram (or 9.8 Newtons) worth of walnuts by balancing this scale as shown, and this will be the case regardless of whether you perform this experiment at rest on Earth, in an accelerated elevator, or even on the surface of a distant world.

We can sum up the difference succinctly: your mass is an inherent quality of the atoms that make up your body, but your weight is dependent on how those atoms accelerate under the influence of all the factors and forces acting on it.

There are plenty of physics textbooks (and physics teachers) that ignore this difference, and simply state that your weight, W, always obeys the equation W = mg. This is incorrect; it is only true when you are at rest on the surface of the Earth. Even under these stipulations, it’s only approximately true; over the entirety of the Earth’s surface, g varies from a minimum of about 9.78 m/s² atop the high plateaus found at Earth’s equator up to a maximum of around 9.83 m/s² at sea level at Earth’s ice-covered north pole. The fact that Earth is not quite spherical, but rather bulges at the equator and is compressed at the poles, means that the acceleration due to gravity at Earth’s surface is not a true constant, but is location-dependent.

Still, it wasn’t thinking about weight and mass on Earth that led Newton to his understanding of gravitation. Instead, it was by thinking about what happens here on Earth’s surface and comparing it with something that happens far away from Earth, but that’s clearly still dominated by the phenomenon of Earth’s gravity: the Moon.

Animation showing the umbral phase of the November 19, 2021 partial lunar eclipse. At 9:03 AM UT, maximum eclipse is reached, where only 0.9% of the Moon remains illuminated by direct sunlight. The umbral phase lasts over 3.5 hours: the longest this century for a partial eclipse. Reconstructing the size of Earth’s shadow relative to the physical size of the Moon is the oldest method for measuring both the size of the Moon as well as the distance to it: a method first leveraged by Aristarchus back in the 3rd Century BCE.

The distance to the Moon had been known for nearly 2000 years before Newton, dating back to the Ancient Greek scientist Aristarchus. Aristarchus correctly reasoned that during a lunar eclipse, the Sun’s rays struck the Earth and created a shadow, and that when the Moon passed through that shadow, the portion of Earth’s shadow that fell on the Moon would encode useful information about the relative sizes of the Earth and Moon, which could then be used to determine the distance to the Moon. This very same method can be used today, millennia later, to measure the Moon’s distance from Earth, although lunar laser ranging experiments can make those measurements far more accurately.

What Newton recognized were two simple facts. First, relative to the size of the Earth, whose radius is just a little bit under 6400 kilometers, the Moon is about 60 times farther away, or around 380,000 kilometers distance. And second, that the amount of time it took the Moon to complete a full 360° revolution around the Earth was approximately 27.3 days: the length of a lunar month when you subtract for Earth’s motion around the Sun, also known as a sidereal month.

When you consider how much distance the Moon travels to make a revolution around the Earth in the time it takes a sidereal month to elapse, Newton immediately realized that the gravitational acceleration of the Moon around couldn’t be equivalent to the gravitational acceleration of objects at Earth’s surface. It had to be far, far less.

This diagram shows the Earth and Moon, as well as the distance between them, to scale. The gravitational force between the Earth and the Moon is proportional to the distance between them squared, which means if the Moon were only half as far away, the gravitational force and the acceleration of the Moon would be quadrupled, but if it were twice as far away, the gravitational force (and hence, the acceleration of the Moon) would be quartered. The Moon is located approximately 60 times farther away from Earth’s center than Earth’s surface is.

In fact, the Moon’s acceleration due to gravity had to be thousands of times less than it would have been relative to Earth’s surface; it had to be around a factor of 3700 times weaker. Instead of accelerating at 9.8 m/s² toward the center of the Earth, the Moon had to accelerate at a mere 0.0026 m/s² in order to maintain its speed and position relative to our planet.

Where does this factor of ~3700 come from? From the fact — swiftly deduced by Newton — that the gravitational acceleration that one object experiences from another was proportional to the inverse of the distance squared between them, and that the Moon being 61 times as far away from Earth’s center as the Earth’s surface was meant that the gravitational acceleration of the Moon due to the Earth would only be 1/61² (or 1/3721) as great as an object accelerating under Earth’s gravity as compared to at its surface.

In other words, if you weren’t at Earth’s surface, but rather were located at the Moon’s distance from Earth, but still had a platform that was anchored to the Earth that you could stand on, you would measure your weight to be just 0.027% of what you measure it to be under conventional circumstances: from here on Earth’s surface. Your weight is dependent not just on your mass, but also on your acceleration.

Apollo 10, known as the ‘dress rehearsal’ for the Moon landing, was actually equipped with all the apparatuses that would have allowed them to land on the lunar surface themselves. They came closer to the Moon than any previous crewed mission, and paved the way for the actual moon landing which took place with Apollo 11 in July of 1969. The entire endeavor required only Newtonian physics, and astronauts in orbit around the Moon experienced themselves as completely weightless.

However, astronauts who journey far away from Earth — whether into low-Earth orbit, into lunar orbit, or even onto the surface of the Moon itself — never experience that “reduced weight” that you would expect from simply applying Newton’s equations. Instead, the following experiences occur.

• An astronaut in low-Earth orbit, such as aboard the International Space Station, experiences themselves as being weightless. Their mass remains unchanged, and their distance from the Earth’s center is only a few hundred kilometers greater than their typical experience on Earth’s surface, but they experience their weight as being zero. They wouldn’t register even a fraction-of-a-pound of force on a scale.
• An astronaut in lunar orbit, which is what the crew of Apollo 8 experienced for the first time as they became the first humans to orbit the Moon, would also experience themselves as being weightless. Again, even though their masses are unchanged, and even though their distances from Earth are much greater than the low-Earth orbiting astronauts, they still register their weight as being zero.
• And an astronaut on the surface of the Moon would not be weightless; their weight would be equal to mg, but this time, g is the gravitational acceleration on the surface of the Moon, not of the Earth, which equates to their weight being about 16% of what it is on Earth’s surface.

Your weight isn’t some objective measure; it’s not even your mass multiplied by your acceleration. Instead, your weight is something you experience because there is a force accelerating you relative to your environment, like the force of the ground (or your seat) that keeps you from falling through the Earth under the influence of its gravity.

The equivalence principle holds that there should be no difference between a gravitational acceleration and an acceleration due to any other force in the Universe. Since one is dependent on the gravitational constant and the other is not, testing the equivalence principle is a way to constrain time variations in the gravitational constant. To date, the most precise test of this principle was accomplished by the MICROSCOPE satellite: demonstrating gravitational and inertial mass equivalence to 1 part in 10^15.

We often talk about “watching our weight” or “trying to lose weight,” but if that was truly your goal, you could simply go to a higher elevation, move to a different planet, or even get into an elevator and wait for the door to close after you hit the “down” button. None of these would give you your desired result, because you’re not interested in changing or controlling or managing your weight; what you’re interested in is your mass, which is a physical property intrinsic to you. Your weight is simply a side effect of having the mass that you do while simultaneously being located on the surface of the Earth, and otherwise at rest with respect to that surface.

If you’re reading this from inside a closed room, it may surprise you to learn that your weight — the very weight you feel in your bones right now — is insufficient to tell you that you are at rest here on Earth. You could just as easily be in a spacecraft or rocket that was in the process of accelerating upward through space; you would feel your weight identically to how you feel it right here and now. There is no fundamental difference between gravity and any other acceleration, although that would be a revelation that wouldn’t come along until Einstein first made it. However, to learn about weight, and specifically the difference between weight and mass, we only needed the insight of Newton. As Matt Strassler recently put it in his fascinating new book,

“Today’s brains aren’t superior to those of several centuries or even several millennia ago. What was conceptually difficult for the human mind back then remains difficult now. The most essential difference between our ancestors and ourselves is merely this: they lived before Newton, and we live after.”