How the Sun *really* shines

You never would’ve guessed that nuclear physics could be this easy.

“Mr. Burns: Smithers, hand me that ice-cream scoop.
Smithers: Ice-cream scoop?
Mr. Burns: Damn it, Smithers! This isn’t rocket science, it’s brain surgery!”
-The Simpsons

The Sun is the one object that’s out-of-this-world that everyone on Earth is familiar with. With a mass that’s some 300,000 times our entire planet’s worth, it’s the most powerful source of heat, light, and radiation in the Solar System by far.

The amount of energy it emits is literally astronomical. Here are some fun facts about the Sun:

• It emits 4 × 10^26 Watts of power, or as much energy as ten quadrillion high-powered power plants would emit running full-bore at once.
• It’s been shining for 4.5 billion years, emitting energy at a nearly constant rate the entire time. (Changing under 20% over that entire time frame.)
• The energy emitted comes from Einstein’s famous E=mc^2, as matter gets turned into energy in the Sun’s core.
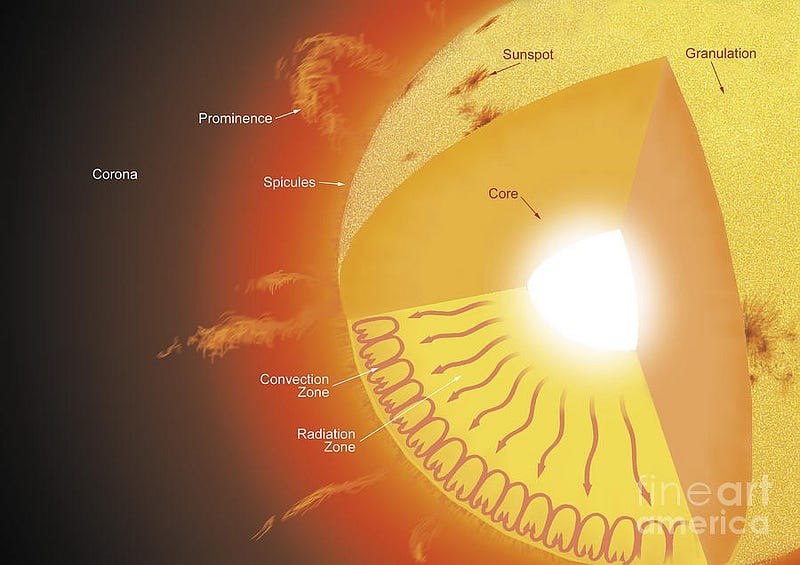
• And finally, that core energy needs to propagate to the Sun’s surface, a journey that requires it to pass through 700,000 kilometers of plasma.

That last step is a lot of fun! Because photons collide with ionized, charged particles very easily, it takes somewhere around 170,000 years for a photon created in the Sun’s core to make it to the surface.

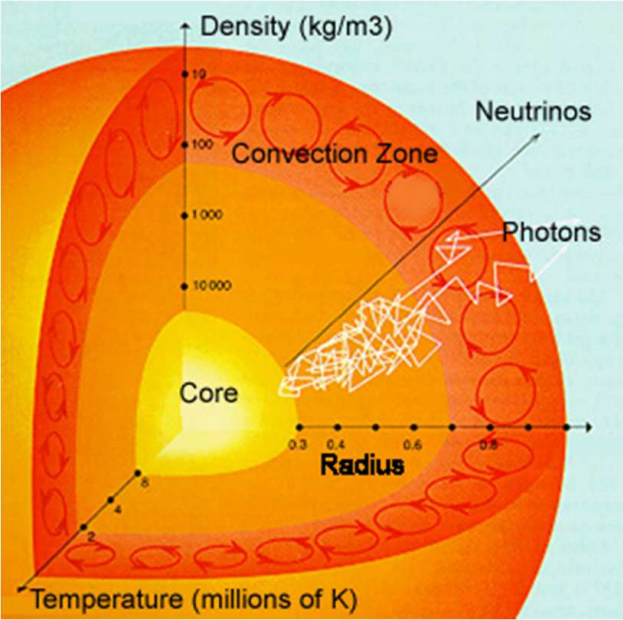
Only then can it leave the Sun and light up the Solar System, our planets, and the Universe beyond. We’ve talked about why the Sun shines (and how we know it works) before, but we never talked about how that all-important step — how its mass gets converted into energy — in detail before.

At a macro level, it’s pretty simple, at least as far as nuclear physics goes.

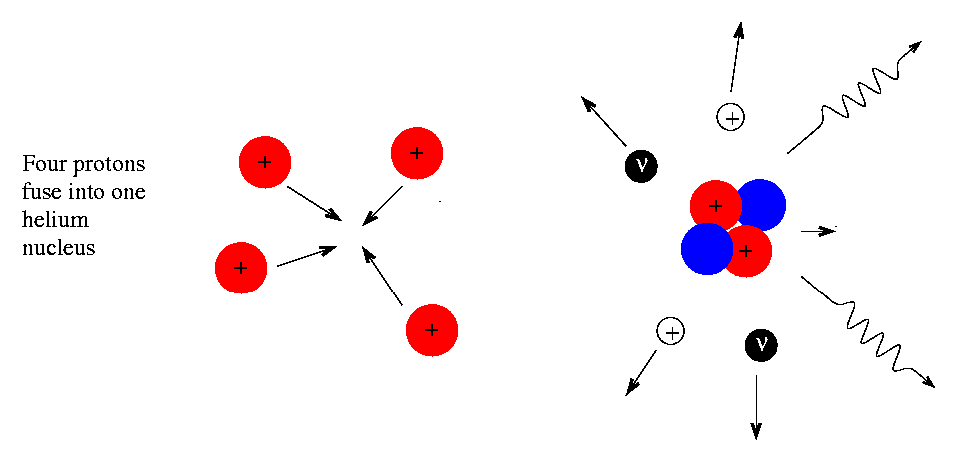
The way nuclear fusion works in the Sun — and in all except the absolute most massive stars — is by fusing humble protons (hydrogen nuclei) into helium-4 (nuclei with two protons and two neutrons), releasing energy in the process.

This might puzzle you slightly, as you may remember that neutrons are ever so slightly heavier than protons.

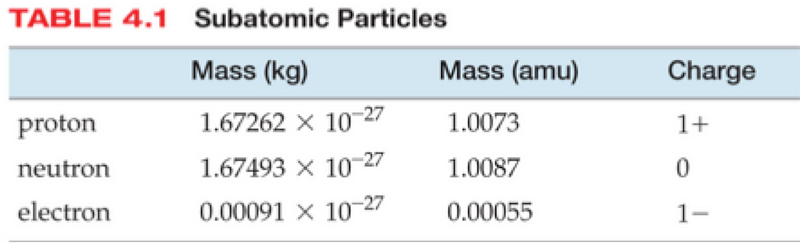
Nuclear fusion only releases energy when the mass of the products — of the helium-4 nucleus, in this case — is less than the mass of the reactants. Well, even though helium-4 is made up of two protons and two neutrons, these nuclei are bound together, which means that their combined mass of the whole is lighter than the individual parts.

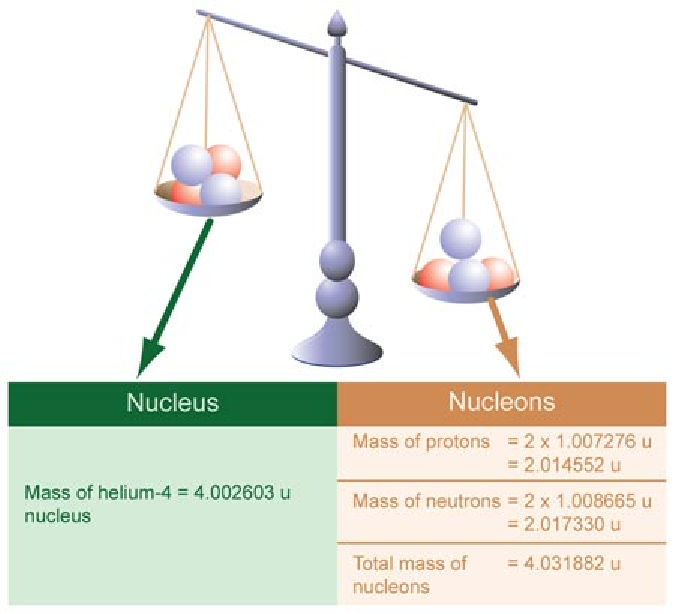
In fact, not only is helium-4 lighter than two protons and two neutrons individually, it’s lighter than four individual protons! It isn’t by all that much — just 0.7% — but with enough reactions, it adds up quickly. In our Sun, for example, somewhere around a whopping 4 × 10^38 protons fuse into helium-4 every second in our Sun; that’s how many it takes to account for the Sun’s energy output.

But it’s not like you can just turn four protons into helium-4; in point of fact, you never get more than two particles colliding at the same time. So how, then, do you build up to helium-4? It might not proceed how you expect!

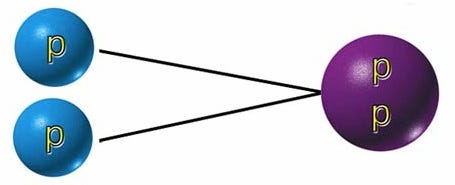
Most of the time, when two protons collide together, they simply do just that: collide, and bounce off one another. But under just the right conditions, with high enough temperatures and densities, they can fuse together to form a state of helium you’ve probably never heard of: a diproton, made up of two protons and no neutrons.

The overwhelming majority of the time, the diproton — an incredibly unstable configuration — simply decays back into two protons.

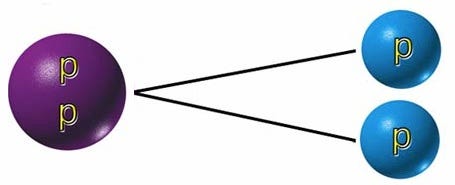
But every rare once-in-a-while, less than 0.01% of the time, this diproton will undergo beta-plus decay, where it emits a positron (the electron’s antiparticle), a neutrino, and where the proton transmutes into a neutron.

To someone who was only viewing the initial reactants and the final products, the diproton lifetime is so small that they’d only see something like the diagram below.

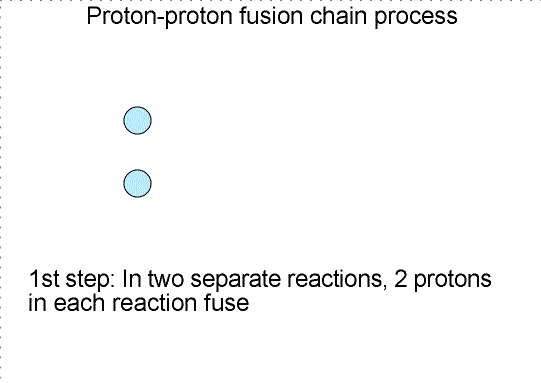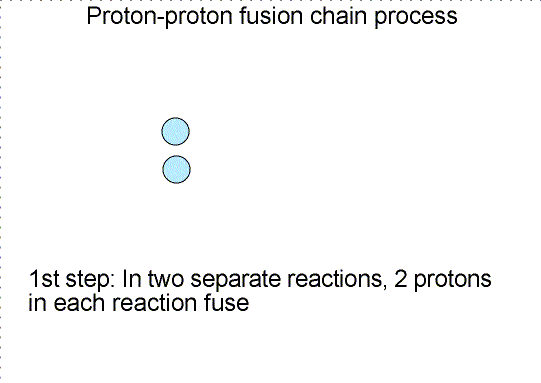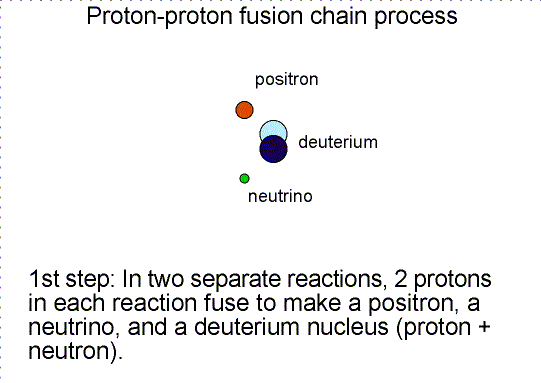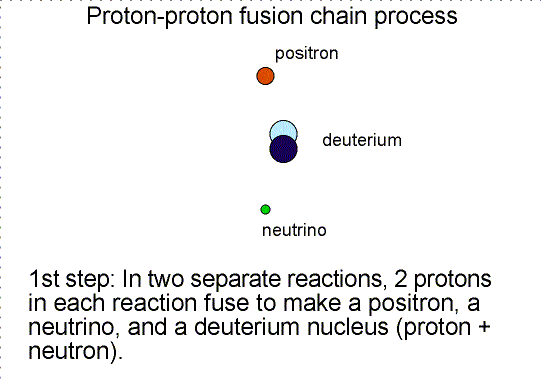
So you wind up with deuterium — a heavy isotope of hydrogen — a positron, which will immediately annihilate with an electron, producing gamma-ray energy, and a neutrino, which will escape at a speed indistinguishable from the speed of light.

And making deuterium is hard! In fact, it’s so difficult that even at a temperature of 15,000,000 K — which is what we achieve in our Sun’s core — those protons have a mean kinetic energy of 1.3 keV apiece. The distribution of these energies is Poisson, meaning that there is a small probability of having protons with extremely high energies, and speeds rivaling the speed of light. With 10^57 protons (of which maybe a few times 10^55 are in the core), I get the highest kinetic energy a proton is likely to have is about 170 MeV. This is almost (but not quite) enough energy to overcome the Coulomb barrier between protons.

But we don’t need to overcome the Coulomb barrier completely, because the Universe has another way out of this mess: quantum mechanics!

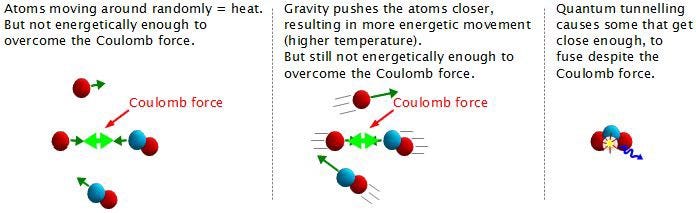
So these protons can quantum tunnel into a diproton state, a small (but important) fraction of which will decay into deuterium, and once you make deuterium, it’s smooth sailing to the next step. While deuterium is only a slightly energetically favorable state compared to two protons, it’s far easier to take the next step: to helium-3!

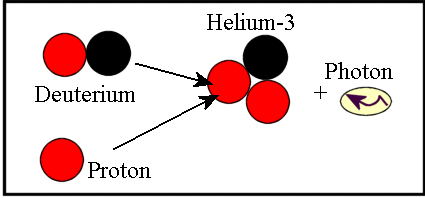
Combining two protons to make deuterium releases a total energy of about 2 MeV, or about 0.1% of the mass of the initial protons. But if you add a proton to deuterium, you can make helium-3 — a much more stable nucleus, with two protons and one neutron — and that’s a reaction that releases 5.5 MeV of energy, and one that proceeds far more quickly and spontaneously.

While it takes billions of years for two protons in the core to fuse together into deuterium, it takes only about a second for deuterium — once it’s created — to fuse with a proton and become helium-3!

Sure, it’s possible to have two deuterium nuclei fuse together, but that’s so rare (and protons are so common in the core) that it’s safe to say 100% of the deuterium that forms fuses with a proton to become helium-3.

This is interesting because we normally think of fusion in the Sun as “hydrogen fusing into helium,” but in reality, this step in the reaction is the only lasting one that involves multiple hydrogen atoms going in and a helium atom coming out! After that — after helium-3 is made — there are four possible ways to get to helium-4, which is the most energetically favorable state at the energies achieved in the Sun’s core.

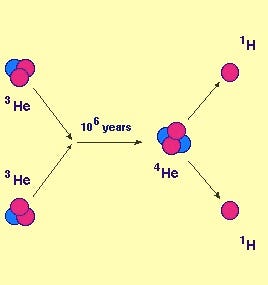
The first and most common way is to have two helium-3 nuclei fuse together, producing a helium-4 nucleus and spitting out two protons. Of all the helium-4 nuclei made in the Sun, some 86% of them are made by this path. This is the reaction that dominates at temperature below 14 million Kelvin, by the way, and the Sun is a hotter, more massive star than 95% of stars in the Universe.

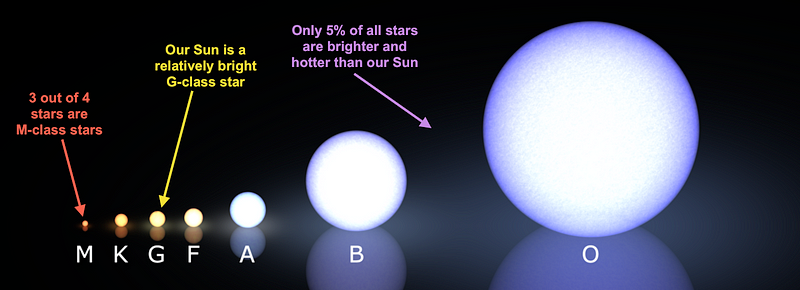
In other words, this is by far the most common path to helium-4 in stars in the Universe: two protons quantum mechanically make a diproton that occasionally decays into deuterium, deuterium fuses with a proton to make helium-3, and then after about a million years, two helium-3 nuclei fuse together to make helium-4, spitting two protons back out in the process.

But at higher energies and temperatures — including in the innermost 1% of the Sun’s core — another reaction dominates.

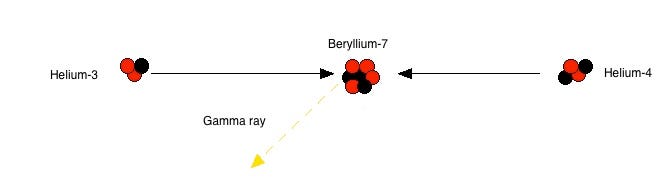
Instead of two helium-3 nuclei merging together, helium-3 can merge with a pre-existing helium-4, producing beryllium-7. Now, eventually, that beryllium-7 will find a proton; because it’s unstable, however, it might decay into lithium-7 first. In our Sun, typically the decay to lithium happens first, and then adding a proton creates beryllium-8, which immediately decays to two helium-4 nuclei: this is responsible for about 14% of the Sun’s helium-4.

But in even more massive stars, proton fusion with beryllium-7 happens before that decay to lithium, creating boron-8, which decays first to beryllium-8 and then into two helium-4 nuclei. This isn’t important in Sun-like stars — accounting for just 0.1% of our helium-4 — but in the massive O-and-B-class stars, this may be the most important fusion reaction for producing helium-4 of all.

And — as a footnote — helium-3 can in theory fuse directly with a proton, producing helium-4 and a positron (and a neutrino) straightaway. Although it’s so rare in our Sun that less than one-in-a-million helium-4 nuclei are produced this way, it may yet dominate** in the most massive O-stars!

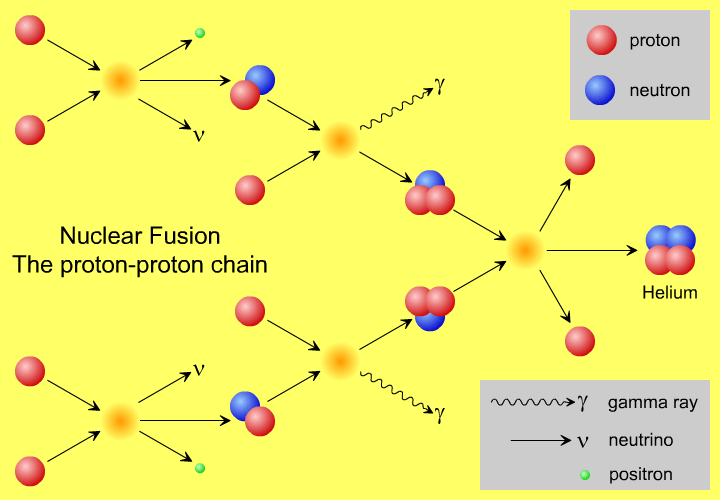
So, to recap, the vast majority of nuclear reactions in the Sun, listing only the heaviest final product in each reaction are:

• two protons fusing together to produce deuterium (about 40%),
• deuterium and a proton fusing, producing helium-3 (about 40%),
• two helium-3 nuclei fusing to produce helium-4 (about 17%),
• helium-3 and helium-4 fusing to produce beryllium-7, which then fuses with a proton to produce two helium-4 nuclei (about 3%).

So it might surprise you to learn that hydrogen-fusing-into-helium makes up less than half of all nuclear reactions in our Sun, and that at no point do free neutrons come into the mix!

There are strange, unearthly phenomena along the way: the diproton that usually just decays back to the original protons that made it, positrons spontaneously emitted from unstable nuclei, and in a small (but important) percentage of these reactions, a rare mass-8 nucleus, something you’ll never find naturally occurring here on Earth!

But that’s the nuclear physics of where the Sun gets its energy from, and what reactions make it happen along the way!

** — And that’s just considering the proton-proton chain; in more massive stars, the CNO-cycle comes into play, a way of making helium-4 with the aid of pre-existing carbon, nitrogen and oxygen, something that happens in all but the very first generation of massive stars!

Have a comment? Weigh in at the Starts With A Bang forum on Scienceblogs!