Ask Ethan: Why does nature obey laws at all?

Throughout the entire Universe, everywhere we look, we see an endless variety of structures that have formed at all different stages of cosmic evolution. With a tremendous number of planets, stars, galaxies, clusters of galaxies, and components of the great cosmic web, no two objects that we find are ever identical. And yet, the fundamental laws that they obey — from the quantum to the cosmic — never appear to change. All across the Universe, gravity works the same way, atoms exhibit the same quantum transitions, and the fundamental constants all remain unchanged throughout time and space.

But why is it that way? Is there anything forbidding it from being different? That’s this week’s inquiry from our Patreon supporter Jeff Bonwick, who wants to know:

“Why does nature obey laws? It’s a relatively recent concept because most of what was observable to our ancestors was macroscopic — thunderstorms, earthquakes, volcanoes — and seemed entirely capricious, the whim of the gods. We now understand that all physical phenomena follow a handful of simple equations, no exceptions, ever… which is quite amazing. But why?”

Physics, although it’s very good at answering questions of “how” things are, is lousy at taking on questions of purpose, such as “why” things are. Here are the best statements we can make about it.

Through the vacuum of space, all light, regardless of wavelength or energy, travels at the same speed: the speed of light in a vacuum. When we observe light from a distant star, we are observing light that has already completed that journey from the source to the observer.

In many ways, it’s the most remarkable fact of all about the Universe: that the constituents, the laws, and the constants of nature, on a fundamental level, do not change throughout space and time. Yes, the structures that they bind together to form change; the conditions under which they exist and interplay with one another change; the various phenomena that emerge from their interactions change. The various complex systems that come into existence are chaotic enough that, in all the Universe, no two are ever truly identical.

But the fundamental constituents (i.e., the particles/quanta), the laws that they obey (i.e., the interactions between them), and the constants that govern their relationships (i.e., the “amount” of any property we examine) are all truly constant.

If this weren’t the case, reality as we know it would be impossible. The fact that reality is consistent from moment-to-moment and location-to-location is the only thing that enables the Universe to be comprehensible in any meaningful fashion. To illustrate this, let’s look at what would happen if any of these three entities — the constituents, laws, or constants — weren’t universally fixed.

On the right, the gauge bosons, which mediate the three fundamental quantum forces of our Universe, are illustrated. There is only one photon to mediate the electromagnetic force, there are three bosons mediating the weak force, and eight mediating the strong force. This suggests that the Standard Model is a combination of three groups: U(1), SU(2), and SU(3), whose interactions and particles combine to make up everything known in existence. Despite the success of this picture, many puzzles still remain.

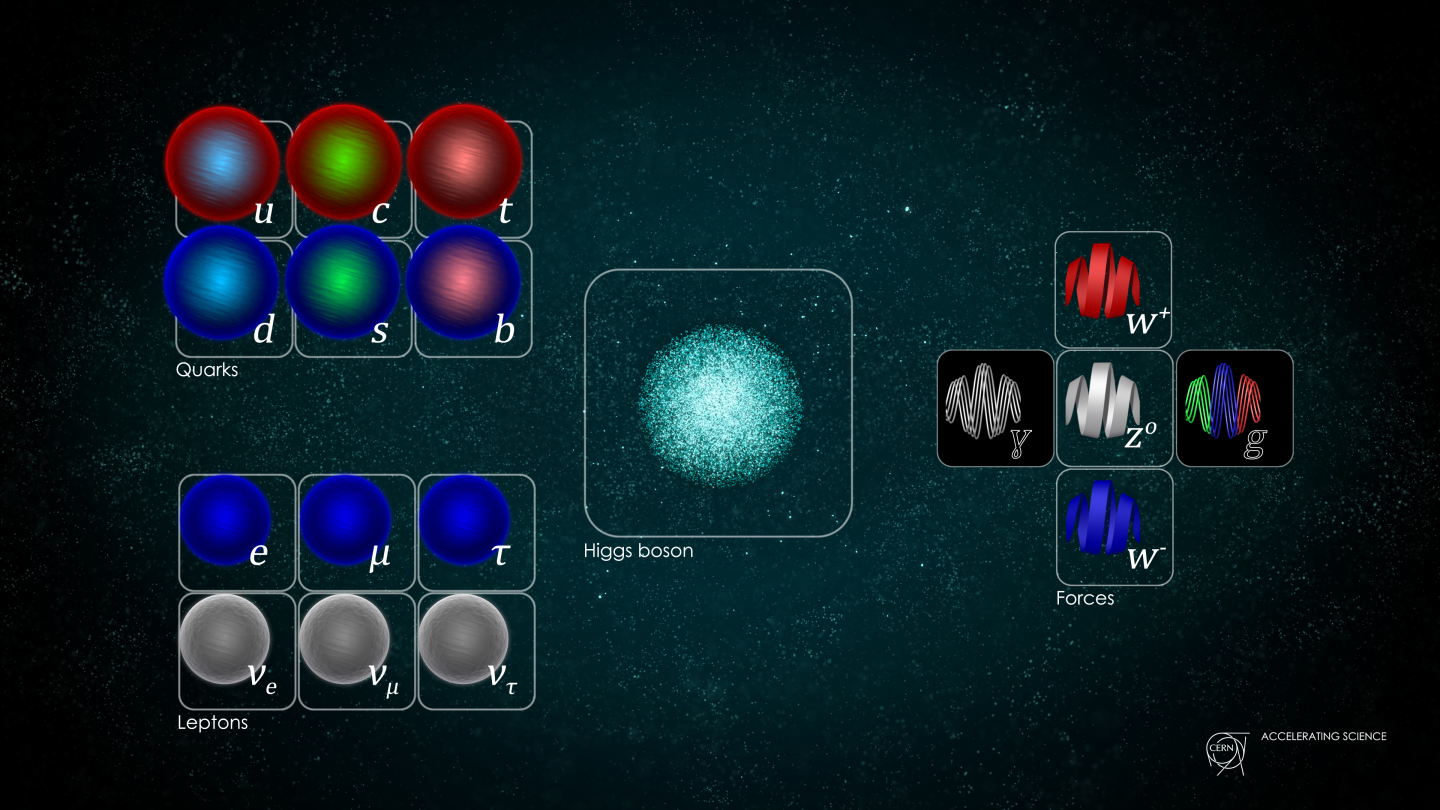
What if the particle content of the Universe weren’t constant?

Imagine that any one of the particles we have and know today, including every particle within the Standard Model, wasn’t a constant. That doesn’t mean “Imagine that one of these particles was unstable,” but rather “Imagine that one of these particles ceased to exist and that either no new particle came to replace it or that one or more novel particles that don’t presently exist came to exist in its stead.”

What would be the consequence of that?

The answer, like it or not, is that everything that exists in the Universe, as we know it, would fundamentally cease to exist, and would be replaced with something new.

If one of the quarks ceased to be, even the elusive and short-lived top quark, then protons and neutrons would take on different fundamental properties. The reason is subtle, but easy to understand. Inside each and every bound hadron — particles made up of quarks — lives a sea of subatomic particles. Yes, particles like protons and neutrons are made up of three (valence) quarks, all bound together by gluons. But there’s a particle-antiparticle “sea” inside each such hadron, where this sea is composed of all the quarks-and-antiquarks popping in and out of existence: precisely what gives these particles the properties they have.

A proton isn’t just three quarks and gluons, but a sea of dense particles and antiparticles inside. The more precisely we look at a proton and the greater the energies that we perform deep inelastic scattering experiments at, the more substructure we find inside the proton itself. There appears to be no limit to the density of particles inside, but whether a proton is fundamentally stable or not is an unanswered question.

If any one of these quarks, or anything that couples to them for that matter, ceased to exist or were replaced by something else, the fundamental properties associated with each such composite particle would no longer remain the same.

Their masses would change, their magnetic moments would change, the structure of the bound nuclei they formed would change, and as a result, the properties of individual atoms and the way that they bind together would all fundamentally change.

If this had occurred anywhere within the Universe, we’d be able to detect it. The bound structures that formed all across the Universe, including individual atoms, would no longer exhibit:

• the same quantum energy levels,
• the same emission and absorption lines,
• the same fine-structure and hyperfine-structure transitions,
• or the same molecular bonds.

Yet this is precisely what we don’t see. Everywhere we look, the spectra of atoms and molecules, in their own rest frame, are identical throughout space and time. The spin-flip transition of hydrogen is always the same. Going back to the earliest signals from the Universe, and in all directions and locations that we can observe, there’s no evidence of any sort of change of this type.

The atomic transition from the 6S orbital in a cesium-133 atom, Delta_f1, is the transition that defines the meter, second, and the speed of light. Slight changes in the observed frequency of this light will occur based on motion and the properties of spatial curvature between any two locations. Spin-orbit interactions, as well as various quantum rules and the application of an external magnetic field, can cause additional splitting at narrow intervals in these energy levels: examples of fine and hyperfine structure.

What if the interactions between particles weren’t constant?

Here in our modern Universe, we’ve got four fundamental forces: gravitation, electromagnetism, plus the strong and weak nuclear forces. If any of these forces weren’t constant, it’s easy to imagine how haywire the Universe would get.

If the gravitational force weren’t constant, there would be no way to reliably predict the motion of objects on Earth, the orbits of celestial bodies within our Solar System, the flight paths of airplanes, rockets, and spaceships, or cosmic properties like gravitational lensing or the expansion of the Universe.

If the electromagnetic force weren’t constant, things would go bonkers on atomic scales. Electrons in orbit around atomic nuclei would see their orbitals and energy levels change, and the binding properties between electrons in different atoms would be up-for-grabs. In other words, every molecule in the Universe, if the electromagnetic force changed, would alter its properties in a fundamental fashion. If this happened where entities like human beings exist, we’d be immediately thrust into an unsustainable configuration. If it occurred on Earth, life would immediately come to an end.

The traditional model of an atom, now more than 100 years old, is of a positively charged nucleus orbited by negatively charged electrons. Although the outdated Bohr model is where this picture comes from, the size of the atom itself is determined by the charge-to-mass ratio of the electron. If the electron were heavier or lighter, atoms would be smaller or larger, as well as more difficult or more easy to ionize, respectively.

And if the strong or weak nuclear forces changed, there would be consequences so catastrophic that we wouldn’t live long enough to know that they’d occurred. Many atomic nuclei that are now stable would decay, shifting into a more stable configuration and causing a tremendous release of energy in the process. Bound atoms would all become ionized, creating — perhaps ironically — a “plasma Universe” wherever this transition occurred.

You might start to wonder about the fact that the electromagnetic and weak forces were once unified into the electroweak force, and you might start to ask about that transition, wondering what effects it had on the Universe.

These are good questions! It turns out that the bound states that we know and (that most of us) love today, which enable the creation of hadrons, atomic nuclei, atoms, molecules, and more, were not possible during electroweak unification. Particles (other than maybe the neutrinos) did not have a rest mass yet, as the Higgs symmetry was restored. The kinetic energy inherent to each particle during the hot, dense, energetic conditions present at these epochs — in the hot Big Bang and in particle colliders both — must decrease so that this symmetry “breaks” again for such bound states to exist. Everything we interact with, today, could only come into existence because of the current form of the Standard Model.

Variations in the fine-structure constant across a wide variety of quasar systems, sorted by redshift. This latest work from 2020 leverages four separate systems at high redshift, but sees no net evidence for a time-variation or a statistically significant spatial variation in the constant itself.

What if the fundamental constants themselves weren’t constant?

This is a big one, and one where people are actively exploring various scenarios as to how the fundamental constants might not, in fact, be constant. There’s a big catch, however: whenever you endeavor to change a constant, including:

• the mass of a particle,
• the coupling strength in any interaction,
• the speed of light,
• Planck’s constant,
• the gravitational constant,
• or the strength of the energy of the quantum vacuum, i.e., the zero-point energy of the Universe,

you have to make sure the scheme you’re proposing isn’t going to conflict with observations, measurements, and experimental results that we already have in hand. Quantum transitions occur with the same specific energy, wavelength, and frequency all throughout the cosmos and all throughout our cosmic history. Atomic clocks have constrained variations in the “ticking” of these clocks to something like 1 part in a quintillion (10¹⁸). And the long-term orbital stability of the Solar System over the past 4.5 billion years is sufficient to constrain changes in gravitation to an extraordinary degree, among others.

The running of the three fundamental coupling constants (electromagnetic, weak, and strong) with energy, in the Standard Model (left) and with a new set of supersymmetric particles (right) included. The fact that the three lines almost meet is a suggestion that they might meet if new particles or interactions are found beyond the Standard Model, but the running of these constants is perfectly within expectations of the Standard Model alone. The fact that the coupling constants may all meet at a point in supersymmetric (SUSY) scenarios may not mean very much for reality.

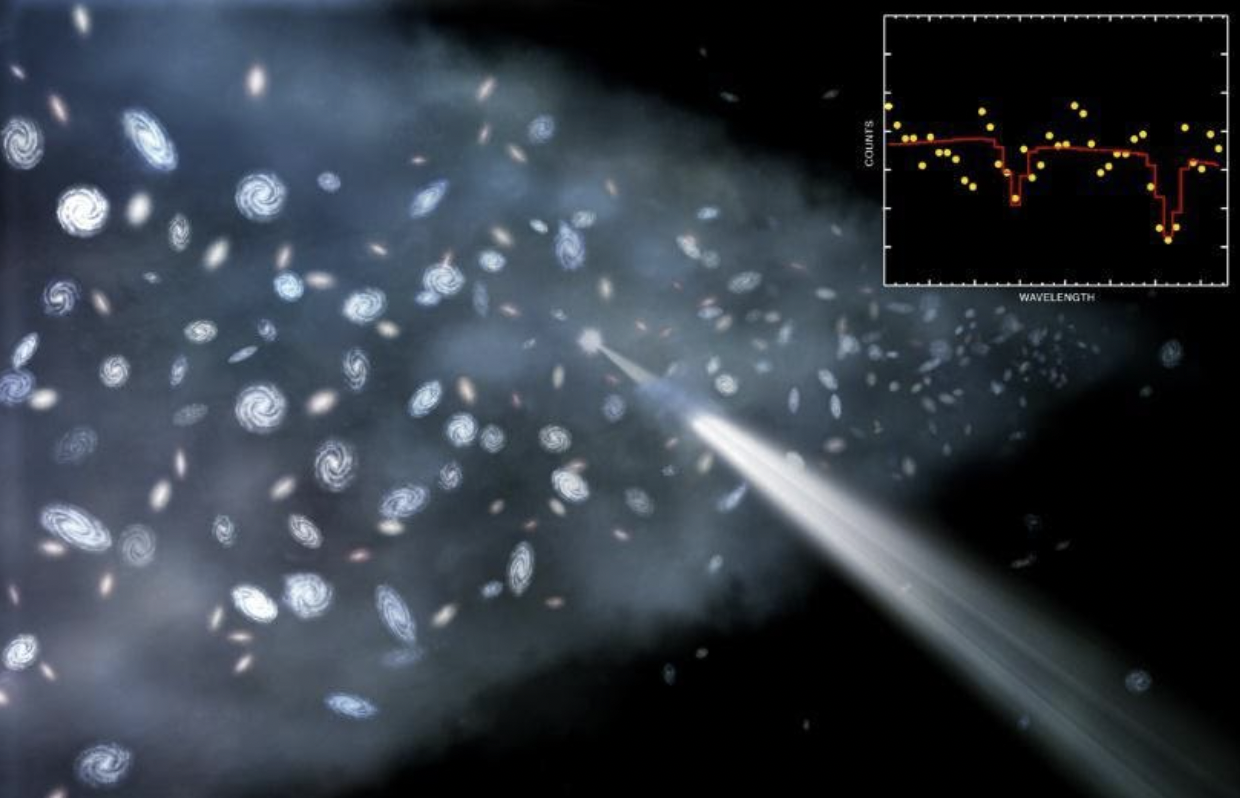
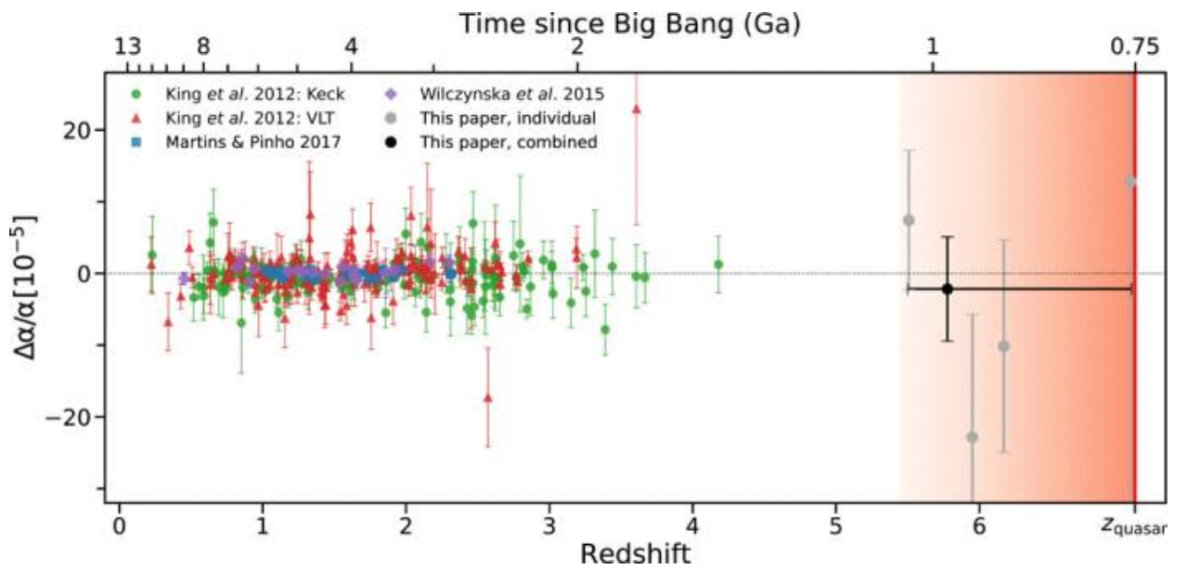
But there is a type of variation that really does occur: the interaction strength of three of the fundamental forces — electromagnetism and the strong and weak nuclear forces — is dependent on the energy scale at which it occurs. For example, the fine-structure constant, which dictates the strength of the electromagnetic interaction, is a fraction that’s about 1/137 today in our low-energy Universe. But at the conditions attained in very high-energy particle colliders, that interaction is stronger: about 1/128 instead. This is understood in terms of quantum field theory, and mandated by them, but it isn’t intuitive; it’s simply a consequence of what “new pathways” for interactions become more probable at high energies.

One thing we can be relatively certain about, however, is that the zero-point energy of the Universe hasn’t changed, at least within our light-cone, since the start of the hot Big Bang. Such a change would be the most catastrophic scenario of all, as the quantum vacuum would have to “tunnel” into a lower-energy state. In doing so, it would fundamentally change all of the constants and interactions in our Universe, destroying every composite particle in the Universe entirely. Wherever it occurred, it would generate a “bubble of destruction” that propagated outward at the speed of light, destroying everything it encountered as it overtook it. Thankfully, we have not detected this occurrence, and our continued existence within this Universe remains possible.

This simplified animation shows how light redshifts and how distances between unbound objects change over time in the expanding Universe. Note that the objects start off closer than the amount of time it takes light to travel between them, the light redshifts due to the expansion of space, and the two galaxies wind up much farther apart than the light-travel path taken by the photon exchanged between them.

You might think that there are deeper reasons why such variations in the laws of physics, either through space or over time, are ruled out. After all, we have some fundamental symmetries and conservation laws in the Universe, and the existence of one arises as a consequence of the other: that’s what Noether’s theorem proved more than 100 years ago.

But that’s only an “if-then” sort of theorem. You no longer need to conserve (or keep constant) the quantities and entities that your perfect symmetries imply if you’re willing to violate the underlying symmetry. Even a slight, tiny violation can give you the wiggle-room you need to defy these conserved quantities.

• You can violate spatial translation invariance (i.e., you can have things be different from place-to-place), and then momentum is no longer necessarily conserved.
• You can violate rotational invariance (things can be different in different directions), and then angular momentum is no longer conserved.
• You can violate time translation invariance (things can be different from moment-to-moment), and then energy is no longer conserved.

Although all of these conservation laws appear to be good for any particle properties we’ve been able to measure in the lab, we’re certain that the last one is not obeyed on a cosmic scale. In the expanding Universe, because cosmic distances vary from moment-to-moment between gravitationally unbound objects, even something as fundamental as energy is not strictly conserved.

Prior to its collapse, one of the scientific tasks that the Arecibo telescope excelled at was measuring isotopes of the same element with the same nuclear charges but different nuclear masses in the Universe. By measuring the spectral properties of a hydroxyl ion found in space, they showed that the fine-structure constant, α, as well as the proton-to-electron mass ratio, had not varied at all over the past ~3 billion years.

There have been, in fact, searches for — and assertions that — the time-evolution or spatial differences from location-to-location in fundamental constants. The fine-structure constant has been claimed to vary at the level of ~a few parts in 1,000,000, both over time and across the great cosmic distances. Unfortunately, this has not been robustly reproducible: the uncertainties in each measurement that claims to detect such a signal are comparable to the size of the overall effect that’s been claimed. Yet time and time again, with each new claim, the robust, independent confirmation has never appeared.

One of the properties of theoretical physics, and it’s up to you whether it’s a flaw or a feature, is that you can never completely rule out an idea that modifies your laws: you can only constrain it. Such an effect may exist, but if it does, it’s below our observational and experimental detection thresholds, or it’s occurred somewhere in our Universe that’s presently outside of our light-cone, and therefore cannot (yet) be seen by us. This is part of why you must never treat physics — and I say this as a theoretical physicist — as a purely theoretical science. Our knowledge of the Universe is informed by experiment and observation. Every time we push those frontiers into previously unexplored territory, we come to understand our reality a little bit better.

Send in your Ask Ethan questions to startswithabang at gmail dot com!