Throwback Thursday: What is the Sun made of?

It’s the greatest source of energy in the Universe, and yet we had no idea until less than 100 years ago.

“The sun is a miasma
Of incandescent plasma
The sun’s not simply made out of gas
No, no, no
The sun is a quagmire
It’s not made of fire
Forget what you’ve been told in the past” –They Might Be Giants

It’s so ingrained in us that the Sun is a nuclear furnace powered by hydrogen atoms fusing into heavier elements that it’s difficult to remember that, just 100 years ago, we didn’t even know what the Sun was made out of, much less what powered it!

From the laws of gravity, we’ve known for centuries that it had to be around 300,000 times the mass of Earth, and from measurements of the energy received here on Earth, we knew how much energy it releases: 4 × 10^26 Watts, or about 10^16 times as much as the most powerful power plants on our planet.

But what wasn’t known was where it got its energy from. No less a figure than Lord Kelvin set out to tackle that question.

From the recent work of Darwin, it was evident that the Earth needed at least hundreds of millions of years for evolution to produce the diversity of life we see today, and from contemporaneous geologists, the Earth had apparently been around for at least a couple of billion years. But what type of power source could be that energetic for that long a period of time? Lord Kelvin — the famed scientist who discovered the existence of absolute zero — considered three possibilities:

1. ) That the Sun was burning some type of fuel.
2. ) That the Sun was feeding on material from within the Solar System.
3. ) That the Sun generated its energy from its own gravity.

As it turned out, each one was insufficient.

1.) That the Sun was burning some type of fuel. The first possibility, that the Sun burned some type of fuel source, made a lot of sense.

The most “combustible” type of fuel is either hydrogen, a hydrocarbon, or TNT, all of which can combine — with oxygen — to release a tremendous amount of energy. Indeed, if the Sun were made entirely out of one of these fuels, there would be enough material for the Sun to produce that incredible amount of power — 4 × 10^26 Watts — for tens of thousands of years only. Unfortunately, even though that’s quite long when compared to, say, a human lifetime, it’s not nearly long enough to account for the long history of life, Earth, or our Solar System. Kelvin, therefore, ruled this option out.

2.) That the Sun was feeding on material from within the Solar System. The second possibility was a little more intriguing. While it wouldn’t be possible to sustain the Sun’s power output from whatever atoms were presently in there, it could be possible in principle to continuously add some type of fuel to the Sun to keep it burning. It was well-­known that comets and asteroids abound in our Solar System, and so long as there was enough new (unburned) fuel being added to the Sun at a roughly steady rate, its lifetime could be extended by large amounts.

However, you couldn’t add an arbitrary amount of mass, because at some point, the increasing mass of the Sun would slightly change the orbits of the planets, which had been observed to incredible precision since the 16th century and the time of Tycho Brahe. A simple calculation showed that even just adding that small amount of mass to the Sun — less than a thousandth of a percent over the past few centuries — would have a measurable effect, and that the steady, observed elliptical orbits ruled this option out. So, Kelvin reasoned, that left only the third option.

3.) That the Sun generated its energy from its own gravity. The energy released could have been powered by the Sun’s gravitational contraction over time. In our common experience, a ball raised to a certain height on Earth and then released will pick up speed and kinetic energy as it falls, and that gets converted to heat (and deformation) when it collides with the Earth’s surface and comes to rest. Well, that same type of initial energy — gravitational potential energy — causes molecular clouds of gas to heat up as they contract and become denser.

Moreover, because these objects are now much smaller (and more spherical) than they were back when they were diffuse gas clouds, it will take a long time for them to radiate all of that heat energy away through their surface. Kelvin was the foremost expert in the world on how the mechanics of how this would happen, and the Kelvin­-Helmholtz mechanism is named after his work on this subject. For an object such as the Sun, Kelvin calculated, it’s lifetime for emitting as much energy as it does would be on the order of tens of millions of years: somewhere between 20 and 100 million years to be more precise.

Unfortunately, that had to be wrong, too! There are stars that get their energy from gravitational contraction, but those are white dwarf stars, not stars like the Sun. Kelvin’s age of the Sun (and stars) were simply far too small to account for what we observed, and so it would take generations — and the discovery of a new set of forces, the nuclear forces — to settle the issue.

In the meantime, we still didn’t even know what the Sun was made out of. The conventional wisdom at the time, believe it or not, was that the Sun was made out of pretty much the same elements that the Earth is! Although that might seem a bit absurd to you, consider the following piece of evidence.

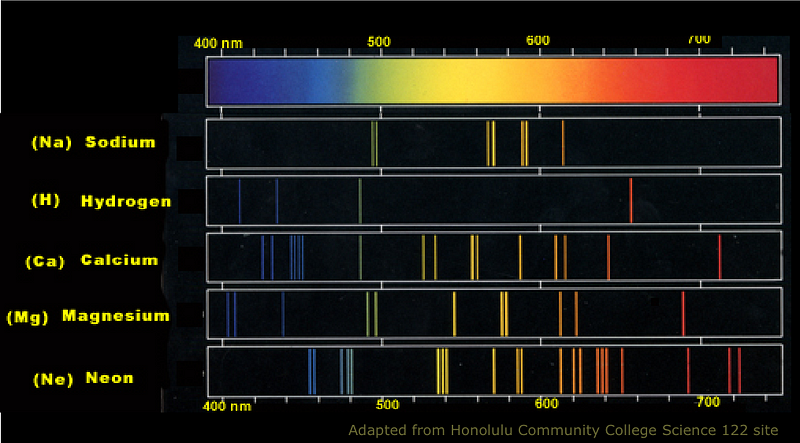
Every element in the periodic table — which was well-understood back then — has a characteristic spectrum to it. When those atoms are heated up, the transitions back down to lower-energy states cause emission lines, and when a background, multi-spectral light is shone on them, they absorb energy at those very same wavelengths. So if we observed the Sun at all these individual wavelengths, we could figure out what elements were present in its outermost layers by its absorption features.

That technique is known as spectroscopy, where the light from an object is broken up into its individual wavelengths for further study. When we do this to the Sun, here’s what we find.

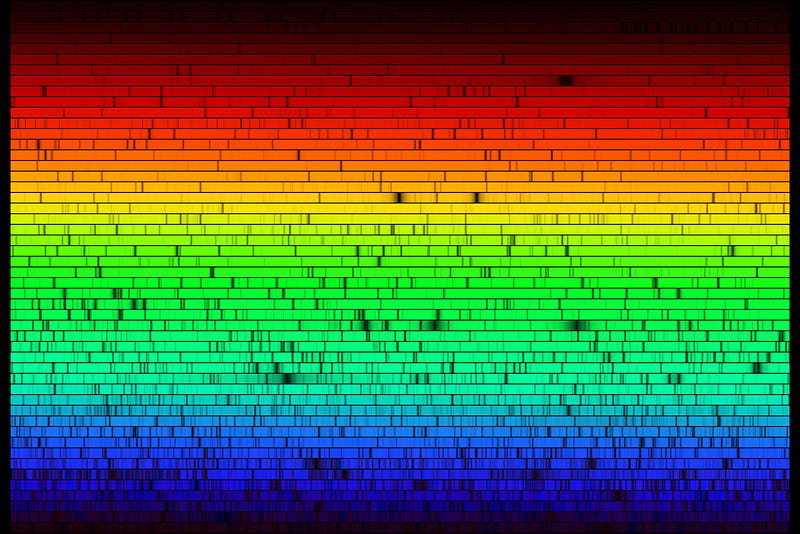
Basically, there are the same elements that we find on Earth. But what is it, exactly, that causes those lines to appear with the relative strengths that they appear. For example, you may notice that some of these absorption lines are very narrow, while some of them are very, very deep and strong. Take a closer look at the strongest absorption line in the visible spectrum, which occurs at a wavelength of 6563 Ångströms.

What determines the strength of these lines, as well as the relative weakness of the lines surrounding it? It turns out that there are two factors, one of which is obvious: the more of an element you have, the stronger the absorption line is going to be. That particular wavelength — 6563 Å — corresponds to a well-known Hydrogen line.

But there is a second factor that must be understood in order to get the strength of these lines right: the level of ionization of the atoms present.

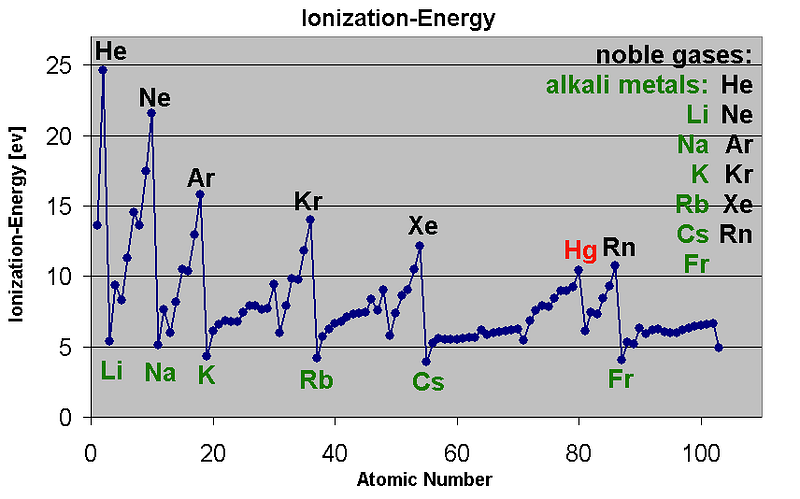
Different atoms lose an electron (or multiple electrons) at different energies. So not only do different elements each have a characteristic spectrum associated with them, they can exist in a number of different ionized states (missing one electron, or two, or three, etc.) that each have their own, unique spectrum!

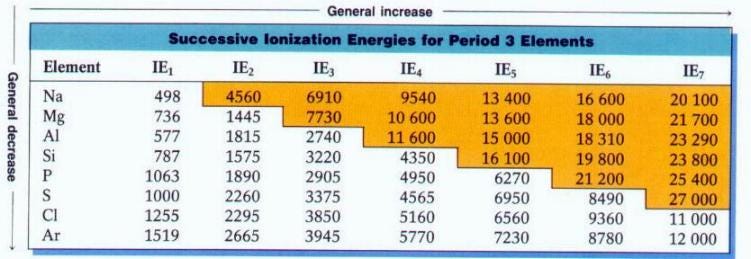
Because energy is the only thing that determines the ionization state(s) of atoms, this means that different temperatures will result in different relative levels of ionization, and therefore, different relative levels of absorption.

So when we’re looking at stars — like the Sun — we know that they come in a huge variety of different types, as a look through any telescope or binoculars will immediately show you, if it isn’t clear to your naked eye.

These stars, very notably, come in strikingly different colors, which tells us that — at least at their surfaces — they exist at vastly different temperatures from one another. Because hot objects all emit the same type of (blackbody) radiation, when we see stars of different colors, we’re really detecting a temperature difference between them: blue stars are hotter and red stars are cooler.

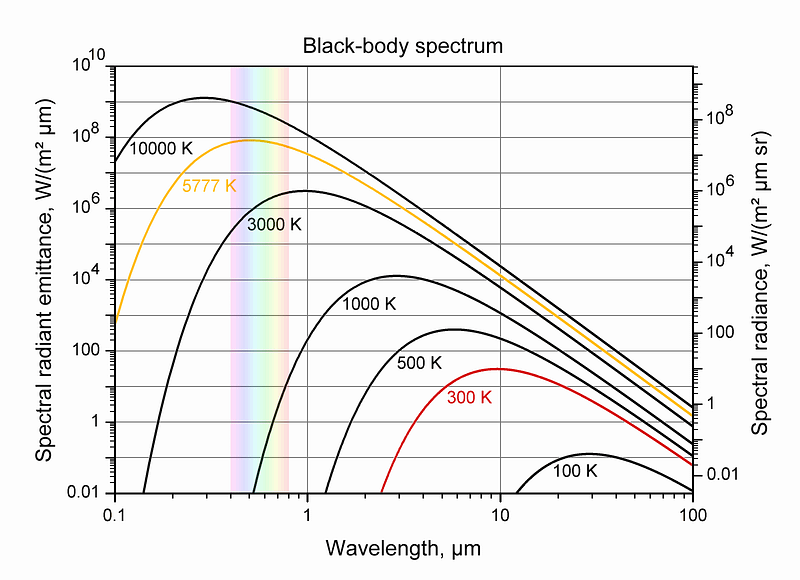
After all, this is — as Annie Jump Cannon figured out — why we classify stars the way we do in modern times, with the hottest, bluest stars (O-type stars) at one end and the coolest, reddest stars (M-type stars) at the other.

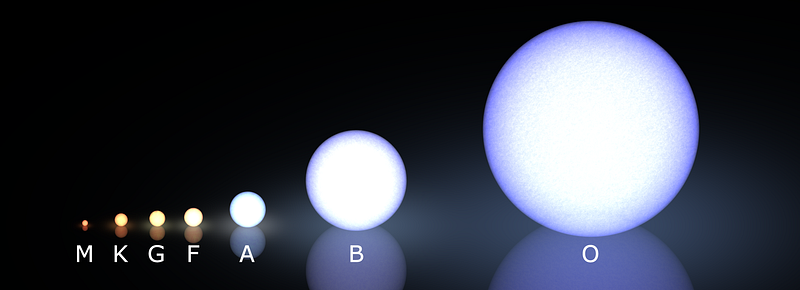
But this was not how we always classified stars. There’s a hint in the naming scheme, because if you had always classified stars by temperature, you might expect the order to go something like “ABCDEFG” instead of “OBAFGKM,” right?

Well, there’s a story here. Back before this modern classification scheme, we instead looked at the relative strengths of absorption lines in a star, and classified them by what spectral lines did or did not show up. And the pattern is far from obvious.

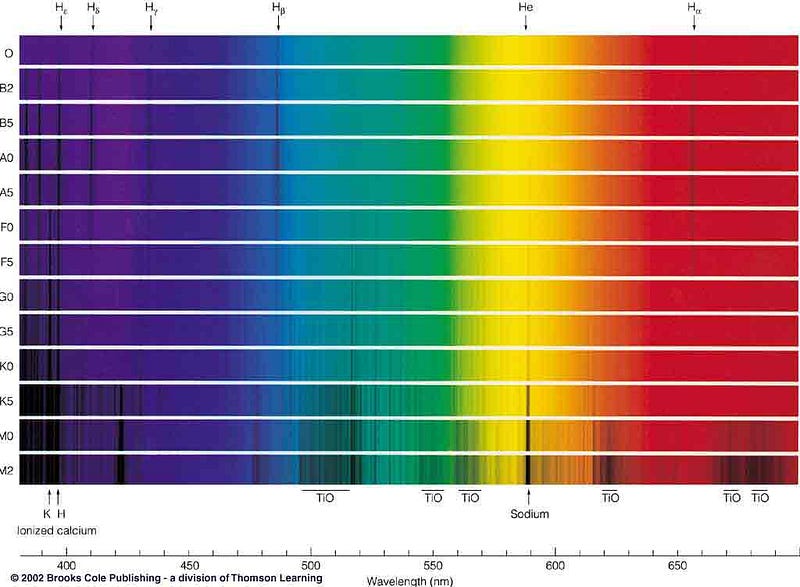
Different lines appear and disappear at certain temperatures, as atoms in their ground state are unable to make certain atomic transitions, while completely ionized atoms have no absorption lines! So when you measure an absorption line in a star, you need to understand what its temperature is (and hence its ionization properties are) in order to rightfully conclude what the relative abundances of the elements are within it.

And if we go back to the Sun’s spectrum, with the knowledge of what the different atoms are, their atomic spectra, and their ionization energies/properties, what do we learn from that?

That, in fact, the elements that are found on the Sun are pretty much the same as the elements found on Earth, with two major exceptions: Helium and Hydrogen were both vastly more abundant than they are on Earth. Helium was many thousands of times richer on the Sun than it is here on Earth, and Hydrogen was about one million times more abundant on the Sun, making it the most common element there by far.

It was only this combined understanding — of how color-and-temperature were related, how ionization was impacted by temperature, and how the strength of absorption lines were a function of ionization — that enabled us to figure out the relative abundances of the elements in a star.

Know who the scientist was who put this all together? I’ll give you a hint: it was a 25-year-old woman who was never fully given the credit she deserved.

Meet Cecilia Payne (later Cecilia Payne-Gaposchkin), who did this work for her Ph.D. thesis way back in 1925! (Astronomer Otto Struve called it “undoubtedly the most brilliant Ph.D. thesis ever written in astronomy.”) Just the second woman to earn her Ph.D. in astronomy through Harvard College Observatory (where she had to move to earn one; her original alma mater, Cambridge, didn’t award Ph.D.s to women until 1948), she wound up having a remarkable astronomy career, becoming the first female chair of a department at Harvard, the first female tenured professor at Harvard, and an inspiration to generations of astronomers, both male and female.

Historically, Henry Norris Russell (the “Russell” of Hertzsprung-Russell fame) was often given the credit for the discovery that the Sun is primarily composed of hydrogen, as he dissuaded Payne from publishing her conclusion — calling it “impossible” — and stated it himself four years later.

Let that be the case no longer! This was Cecilia Payne’s brilliant discovery and she deserves full credit for making it. The strength of the absorption lines combined with the temperature of the stars and the known ionization properties of atoms leave you with the inescapable conclusion: the Sun is a mass of primarily Hydrogen! Years later, we figured out that it was the nuclear fusion of these hydrogen nuclei into helium that powered the Sun and most of the stars, but it was all made possible thanks to Cecilia Payne, and her amazing insights into the workings and the composition of stars.

Leave your comments at the Starts With A Bang forum at Scienceblogs!