Ask Ethan: Could gravitational waves collapse into a black hole?
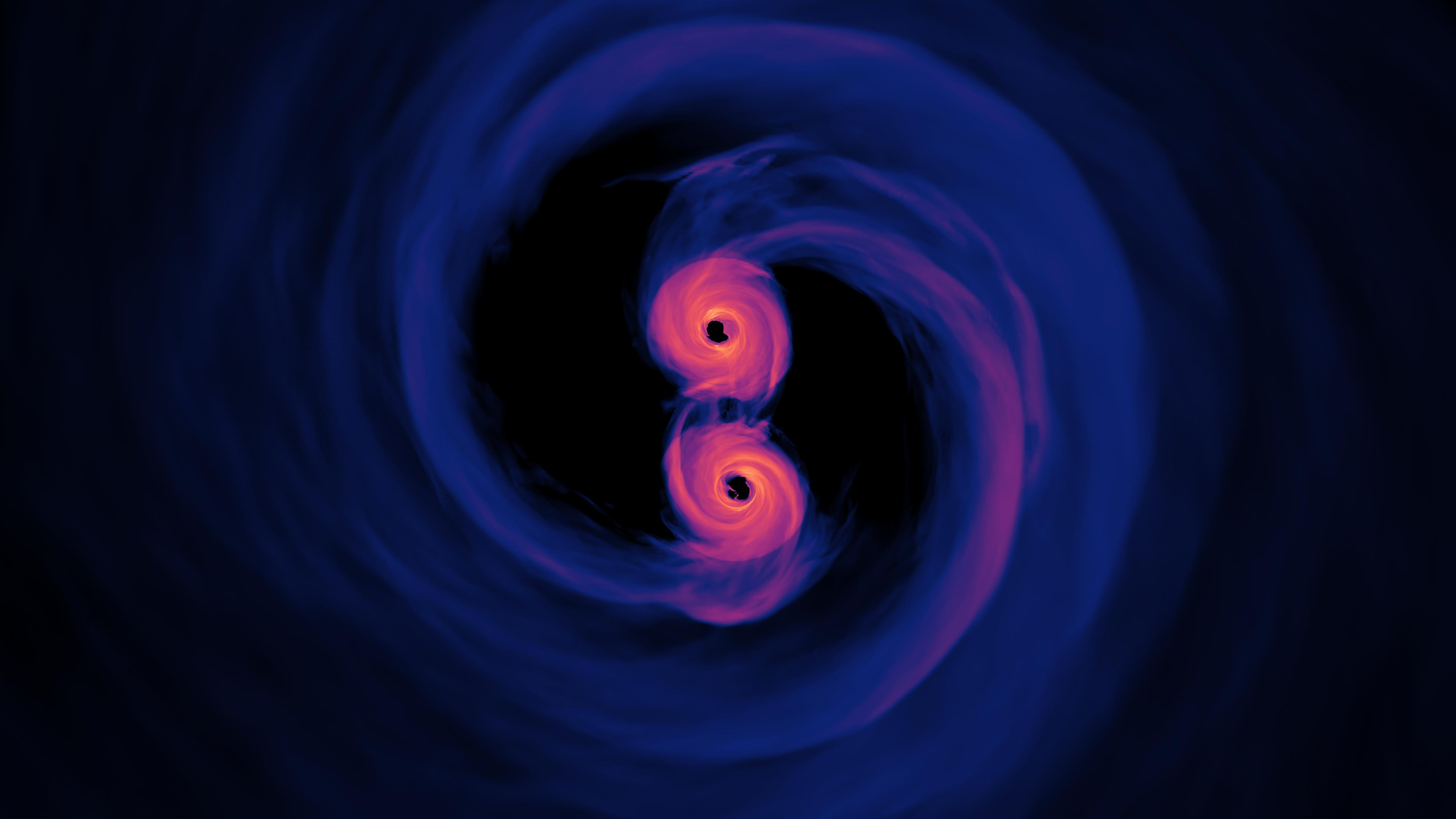
- Anytime a mass moves or accelerates through a region of spacetime where the curvature changes, that process causes the spontaneous emission of a special type of radiation: gravitational waves.
- For the most energetic events of all, the power emitted in gravitational radiation can be tremendous: briefly emitting more energy than all the stars in the Universe combined.
- With such great energies, and with gravitational waves emerging from such a tiny region of space, could those waves carry so much energy that they’ll create a black hole? The science is fascinating.
Back when Einstein’s general relativity first came out, there was a fascinating consequence that was recognized almost immediately: that masses didn’t just move through curved space, but as they did so, they would be compelled to emit gravitational radiation. Orbits between gravitationally bound masses would no longer be eternally stable, but would slowly decay over time, as the energy carried away by this radiation — gravitational waves — would have to be “stolen” from the moving masses themselves. Over time, all objects that were bound in a co-orbiting gravitational dance would eventually inspiral and merge.
We’ve now witnessed this occurring directly for several different classes of objects:
- black hole-black hole systems,
- black hole-neutron star systems,
- and neutron star-neutron star systems,
with gravitational waves detected directly for each one. In fact, the first black hole-black hole merger ever seen emitted so much energy in gravitational waves that for a few tens of milliseconds, they emitted more energy than all the stars within the observable Universe combined. With so much energy there, one can’t help but wonder about what could be possible, which inspires this week’s Ask Ethan question from Patreon supporter Chad Marler:
“Is it hypothetically possible that a gravitational wave could carry so much energy, that it collapses in on itself into a black hole?”
Just because you can imagine a scenario like this in your head doesn’t mean it’s physically plausible given the actual way objects behave in our Universe. Let’s dive into gravitational wave physics to find out.
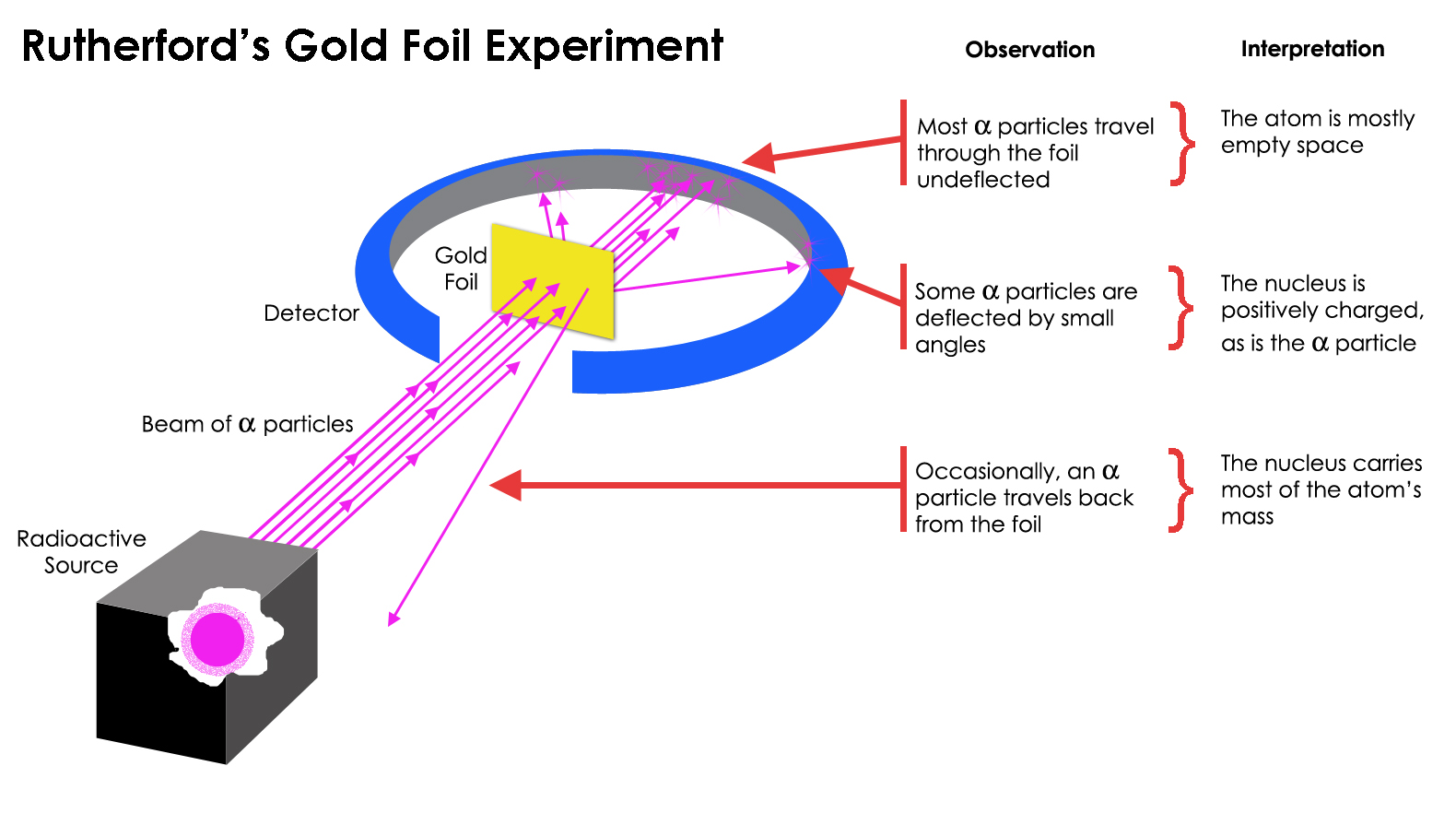
The Rutherford problem
The “inspiral” problem didn’t begin with gravitational waves, but rather came about even before general relativity existed: when physicist Ernest Rutherford showed that atoms were made up of tiny, massive, positively charged atomic nuclei that were orbited by much less massive, negatively charged electrons. Prior to his famous gold foil experiment, it was thought that atoms were large and diffuse, where the positive charge was like a “pudding” and the electrons were like fruit embedded within that pudding. However, as illustrated above, Rutherford took a:
- very thin foil of gold, hammered until it was only a few hundred atoms thick,
- created a sheet of foil in the way of a radioactive source,
- and measured what happened to the particles emitted by that radioactive source.
The expectation was that these fast-moving radioactive particles would all pass through the gold foil, the same way you’d expect a cannonball to pass through a tissue. But while this was true for most of the particles, many of them ricocheted in random directions, with some of them headed straight back toward the emitting source itself. This demonstrated the existence of a dense, massive nucleus at the core of an atom, shocking the experimenters. As Rutherford later stated,
“It was quite the most incredible event that has ever happened to me in my life. It was almost as incredible as if you fired a 15-inch shell at a piece of tissue paper and it came back and hit you.”
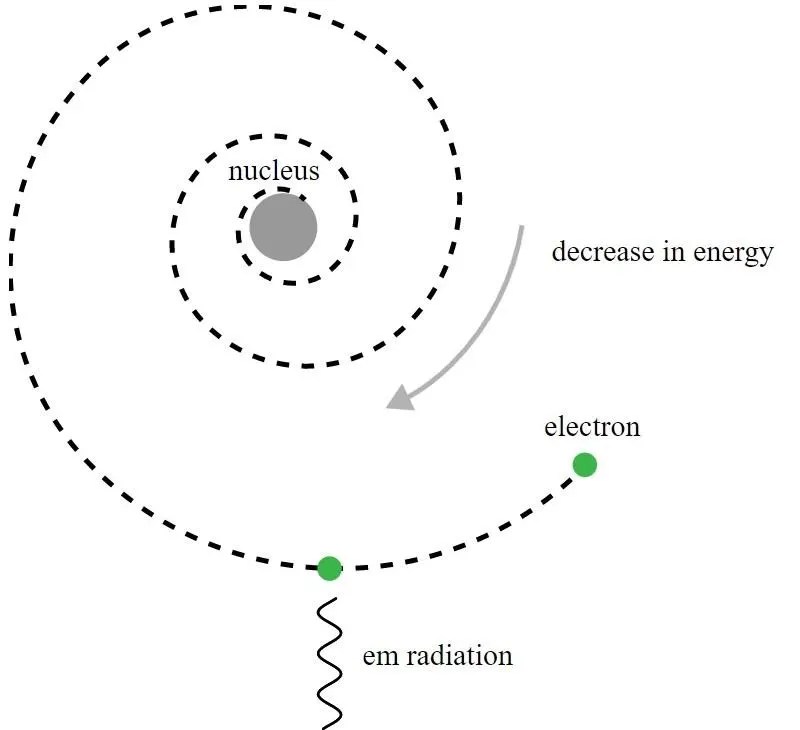
This 1909 discovery would net Rutherford a Nobel Prize, but Rutherford was rather dismayed at his discovery’s implications. If dense, massive atomic nuclei were orbited by electrons — and if that was the structure of the atom — then the laws of electromagnetism presented an enormous problem. All charged particles create electric fields around them, and if charged particles either move or spin, they also create magnetic fields. If a negatively charged particle (an electron) orbits around a positively charged particle (an atomic nucleus), then both electric and magnetic fields within the system change, and these changing fields are exactly what you need to generate electromagnetic radiation: light.
But light carries energy, and so the emission of energy would cause the electron and the nucleus to inspiral, where they’d orbit each other more tightly. Then, tighter orbits would lead to greater speeds, which would cause these electric and magnetic fields to change more quickly than before, leading to an even greater emission of radiation. In short order — on timescales of less than a second — all atoms should see their electrons inspiral into their nuclei, leading to a pathology. It would take the revolution of quantum physics to make sense of what was actually happening instead, but the conceptual puzzle raised by Rutherford would rear its head again in a new way when general relativity arrived on the scene.
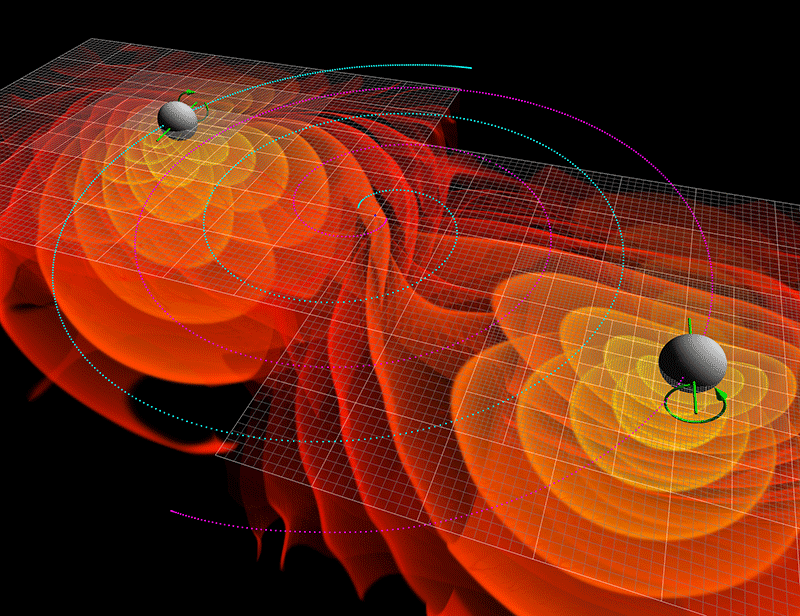
Radiation in general relativity
Although there aren’t positive and negative charges in gravity, only “positive mass” masses and “positive energy” radiation, the problem of orbiting masses in general relativity has the same pathology that Rutherford first identified. If you have two masses in a gravitationally bound orbit around one another, then one mass moves-and-accelerates through a changing gravitational field (or a region of changing spacetime curvature) just like electric charges moved through changing electromagnetic fields in the Rutherford problem, and therefore radiation will be emitted just like before. Only this time, it should be a fundamentally gravitational type of radiation, gravitational waves, rather than light, which is an electromagnetic wave.
A “normal” system, like the Earth orbiting the Sun, will survive for an extraordinarily long time before enough gravitational waves are emitted to cause an inspiral-and-merger: something like 1026 years for our planet, or much, much longer than the lifetime of the Sun itself. But for more massive systems at much closer distances, like binary neutron star systems or binary black hole systems, inspirals and mergers can take place in much shorter periods of time: timescales that are less than ~1010 years, or younger than the age of the Universe. If gravitational waves were real, the way Einstein’s theory predicted, then dense, orbiting masses at very close distances to each other should inspiral and merge in a detectable way.
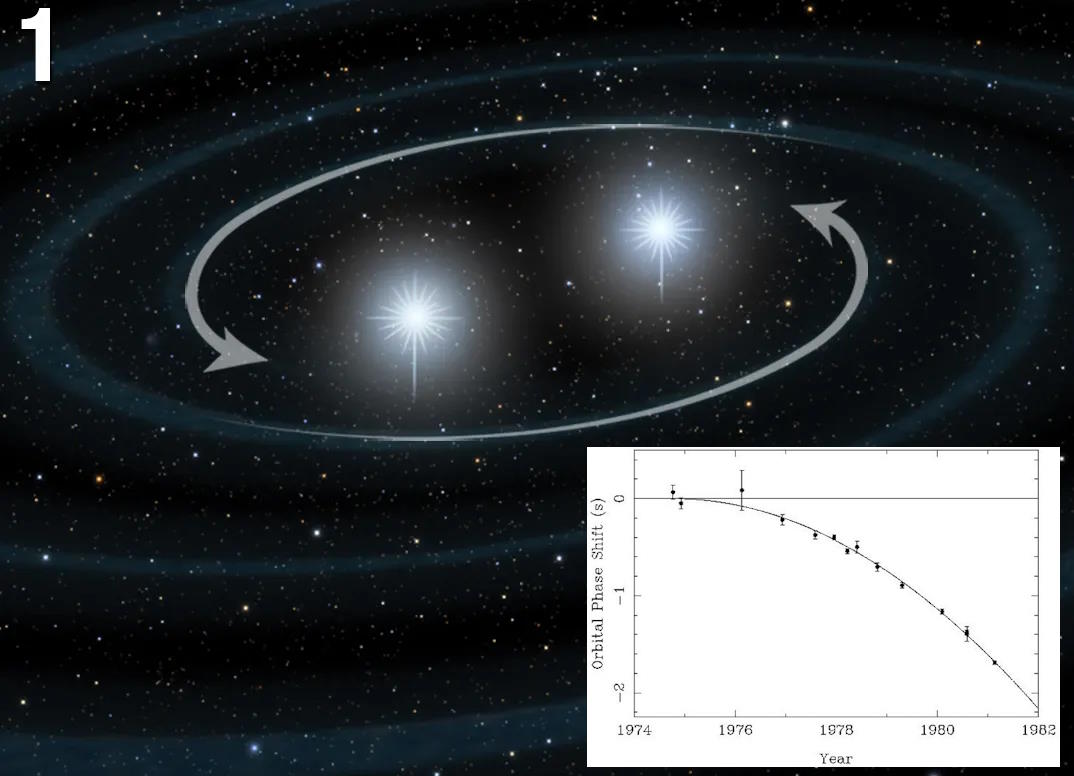
The first sign of gravitational waves: binary pulsars
Most people recall that the first direct detection of gravitational waves was announced in 2016: just eight years ago, or more than a full century after Einstein’s general relativity was first unleashed on the world. But way back in the 1970s, we received our first indirect evidence of the existence of gravitational waves: through the discovery and continued monitoring of the first known binary system consisting of two neutron stars. Known as the Hulse-Taylor binary, it was discovered by Russell Hulse and Joseph Taylor back in 1974. One of the binary stars is a millisecond pulsar, while the other is a neutron star whose pulses we can see.
Millisecond pulsars, as good luck would have it, are the best natural clocks in the Universe, and the pulsar in the Hulse-Taylor binary has a period of just 59 milliseconds. Regular variations of the arrival time of the pulses showed that there was a smooth, repetitive change that occurred every 7.75 hours, indicating the presence of a binary companion at very close distances: never more than 5 solar radii (or 3.4 million kilometers) away from each other. Over the span of many years, it was easy to see that the orbital period was slowly decreasing: consistent with the idea that gravitational waves were carrying energy away and causing the system to inspiral exactly as Einstein predicted. These neutron stars should complete their inspiral and merger after another 300 million years, so we’d have to look elsewhere in order to directly detect gravitational waves.
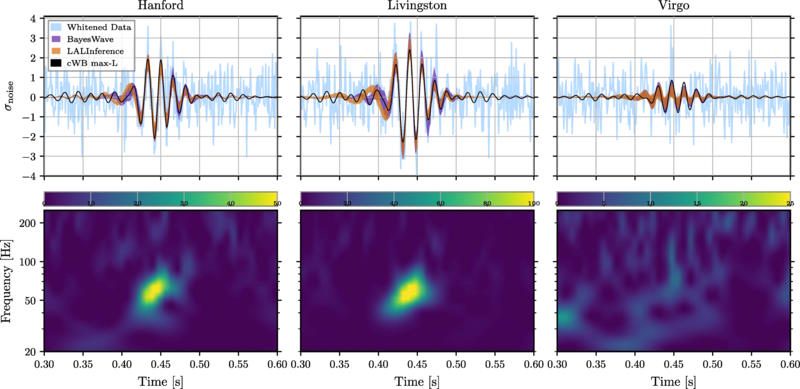
Direct detection at last
It was only with the advances brought along by constructing advanced LIGO — the Laser Interferometer Gravitational wave Observatory — that we became sensitive enough to the gravitational waves created during the most energetic phase of their emission (the inspiral that leads-up to the merger, plus the actual merger itself, plus the “ringdown” phase in the immediate aftermath) to detect them directly. While the Hulse-Taylor binary emits about ~2% of the Sun’s power (a little less than 1025 watts) in the form of gravitational waves, the first gravitational wave event ever seen, GW150914, peaked at more than 1049 watts, or more power than all the starlight from all the stars within the known, observable Universe.
Of course, that’s the total power emitted from the source in gravitational waves at its absolute peak. Much like starlight, gravitational waves are typically emitted in all directions, with gravitational radiation streaming outward in a spherical fashion from the source. There can be some collimation, or a “preferred direction” to the emission, but that typically only occurs under specific circumstances: dependent on the alignment of the spins of each black hole as well as the spin-orbit alignment as two objects come together and merge.
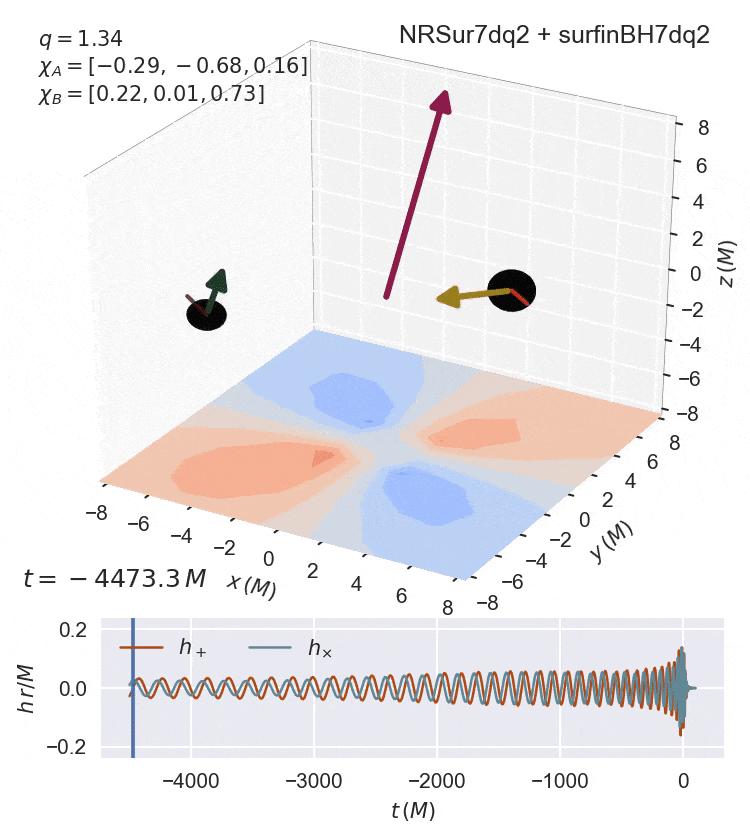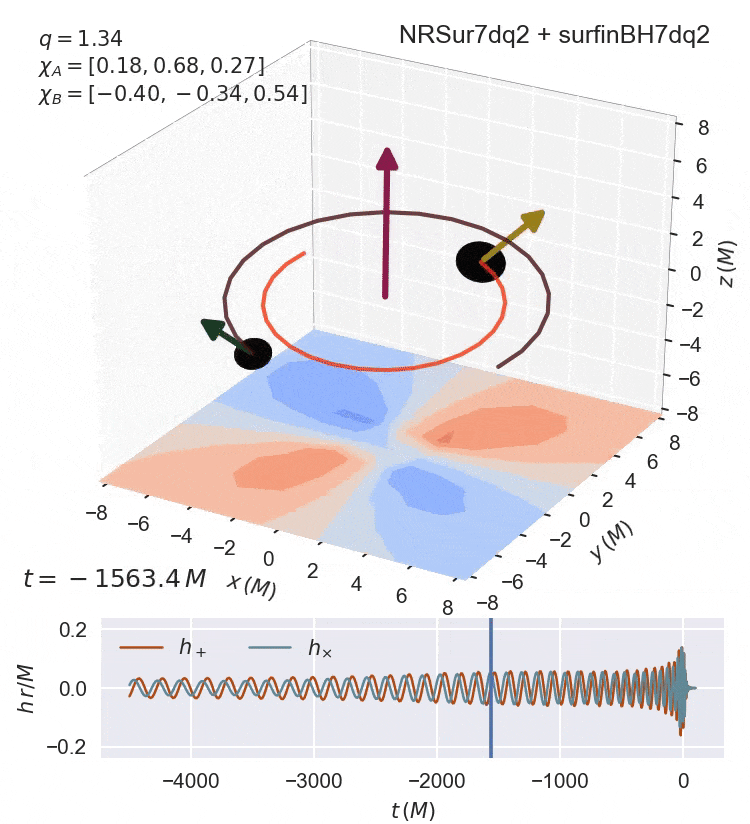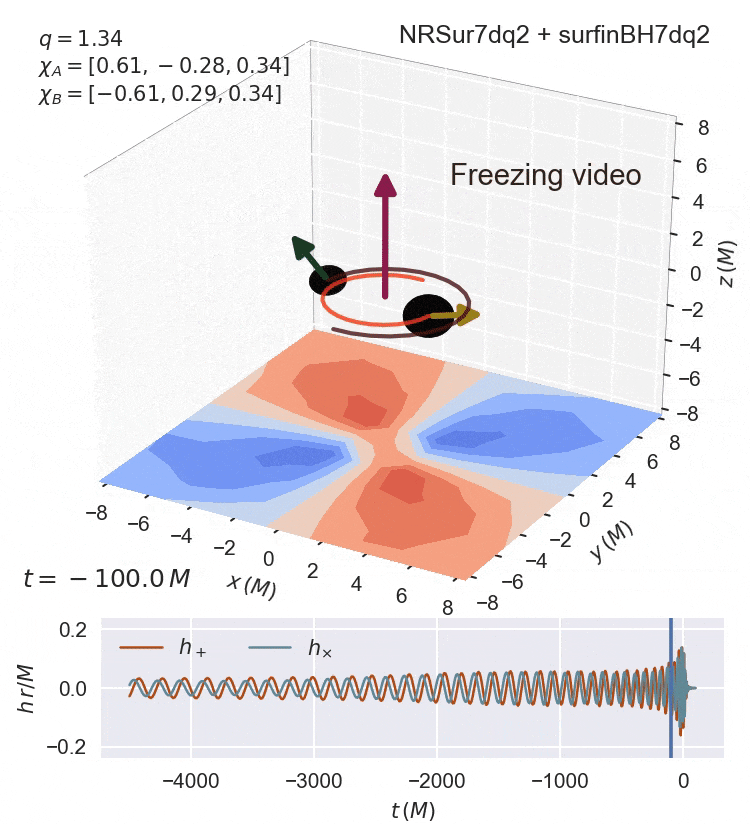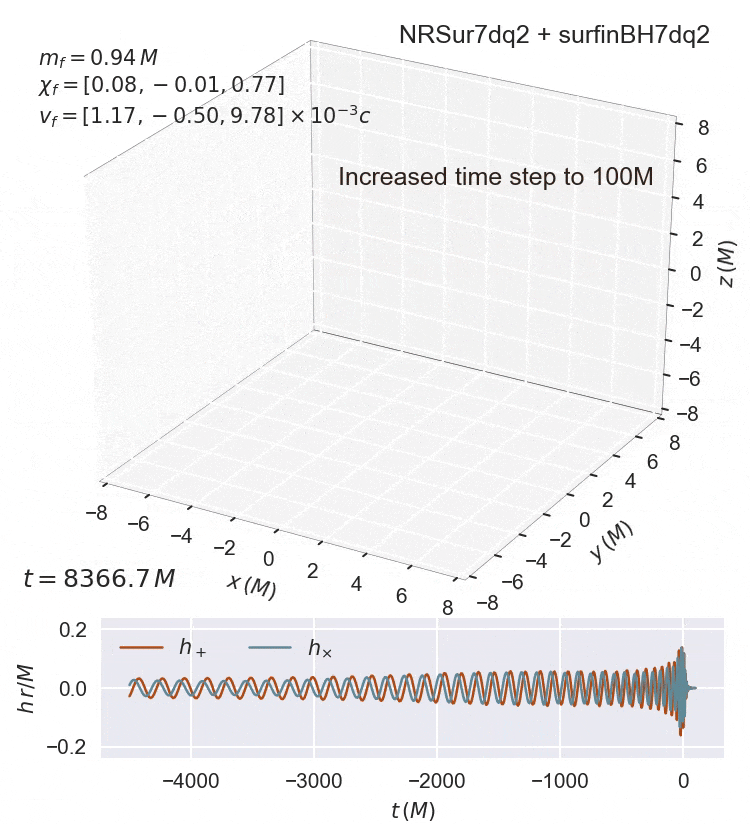
This directionality is minor, however, as even in the most severe cases we’ve studied, the departure from spherical gravitational wave emission is only a few percent at most.
Meanwhile, we can ask ourselves the question of how this Universe generated the most powerful gravitational wave signals of all. The strongest gravitational waves are generated by:
- the largest-mass objects,
- confined to the smallest volumes,
- that are in the final orbits of their inspiral phase, just before and at the moment of their mergers.
In our Universe, that corresponds to merging supermassive black holes. As these masses get closer and closer together during the inspiral phase, the period of orbit gets shorter, which corresponds to higher-frequency gravitational waves, and the amplitude of the signal increases, as more and more power gets generated at shorter distances.

Why do the waves stop?
However, all of a sudden, just as the power emitted by these inspiraling black holes reaches a peak, these gravitational waves cease to be emitted altogether. The two black holes have now passed within one another’s event horizon, and those ripples in spacetime face the same problem as light: they cannot escape from within the black hole’s event horizon. Once both masses, like two black holes (or a neutron star-black hole pair, or a neutron star-neutron star merger that creates a black hole), fall behind the event horizon, that’s the end of the game: no more gravitational waves generated from inside the event horizon can get out. All that escapes is gravitational radiation from what’s known as the ringdown phase: where the event horizon changes back into a shape that represents a single, final, post-merger object, as opposed to the two distinct objects from before the merger.
The problem, once again, with any gravitational wave is that it can only be emitted from outside a black hole’s event horizon, and never from the interior. This is a very, very big deal, because even if the (suspected) central singularities inside the black holes themselves are very small, the event horizons around these black holes are quite large. A black hole of one solar mass might have a singularity that’s smaller than the size of your fingernail, but its event horizon is several kilometers in diameter. And, on top of that, the more massive your black hole is, the larger its event horizon is as well.

Even though the most massive black holes emit the greatest amount of gravitational wave power and energy of any phenomena within the Universe when they merge, that energy is radiated from a large area: the surface area of the event horizon of the black hole itself. Moreover, that energy propagates outward in a spherical fashion: spreading out over time and becoming less dense. When black holes merge, despite all the energy they emit in the form of gravitational waves, there’s an upper limit to how much energy that can be: about ~10% of the mass of the smaller-mass black hole involved in the merger. With that little energy spread out over such a large region of space — larger than the black hole’s event horizon — collapse to a black hole from that state isn’t physically plausible.
That’s the big problem with event horizons: anything that falls inside of them cannot get out, including the gravitational waves that should be emitted during the most severe stage of the merger process. Once you’re behind that event horizon, you’re not escaping, even if you’re a gravitational wave. It’s also a big problem that the more massive your black hole is, sure, it can emit more energy and power during a merger, but its event horizon is also larger, causing the generated waves to be spread out over a larger surface area and only propagating outward from there.
The last possibility
The best scenario one can envision is to imagine that you have two (or more) separate black hole-black hole systems, with their spins and orbits aligned in such a way that they’ll produce collimated gravitational waves that preferentially come out in one direction, and that those multiple systems have their waves oriented so that they propagate directly toward one another. Yet, even in this scenario, the only way to get the waves to be energetic enough to create a black hole is to have these multiple mergers occur close enough together so that the waves can’t spread out.
As it turns out, the “closeness” required needs to be so severe that you’d never make a separate black hole from either of the two-or-more mergers that need to occur; they’d only be able to form within the event horizon of the post-merger black holes, which essentially prevents such a black hole from forming. The key takeaway is this: gravitational waves obey the same rules about the event horizon as light and particles do, in the sense that once they cross over to the inside of the event horizon, they can no longer be emitted, re-emerge, or “get out” in any way.
Someday, someone may figure out a very clever way to make perfectly collimated gravitational waves with a great enough amplitude to create a black hole from this type of energy alone. But based on the physics we know and the understanding of the Universe we have today, the answer to this particular Ask Ethan question, of whether gravitational waves could collapse into a black hole, seems to simply be, “no.”
Send in your Ask Ethan questions to startswithabang at gmail dot com!