Dimensional Reduction: the key to physics’ greatest mystery?

Could the secret to understanding gravity be held in reducing, not increasing, the number of dimensions?

This article is written by Sabine Hossenfelder. Sabine is a theoretical physicist specialized in quantum gravity and high energy physics. She also freelance writes about science.

“Dimension regulated the general scale of the work, so that the parts may all tell and be effective.” –Vitruvius

What if the Universe — and fundamentally, space itself — were like a pile of laundry?

Have one.
See this laundry pile? Looks just like our universe.
No?
Here, have another.
See it now? It’s got three dimensions and all.
But look again.
The shirts and towels? They’re not truly three-dimensional. They’re really crinkled and interlocked two-dimensional surfaces.
Wait.
These surfaces aren’t real, after all. It’s really one-dimensional yarn, knotted up tightly.
You ok?
Have another.
I see it clearly now. It’s everything at once, one-two-three dimensional. It just depends on how closely you look at it.
Amazing, don’t you think? What if our universe was just like that?

It doesn’t sound like a sober thought, but it’s got math behind it, so physicists think there might be something to it. Indeed the math has piled up lately. They call it “dimensional reduction,” the idea that space on short distances has fewer than three dimensions — and it might help physicists to quantize gravity.

We’ve gotten used to space with additional dimensions, rolled up so small (or compactified) that we can’t observe them. But how do you get rid of dimensions instead? To understand how it works we first have clarify what we mean by “dimension.”

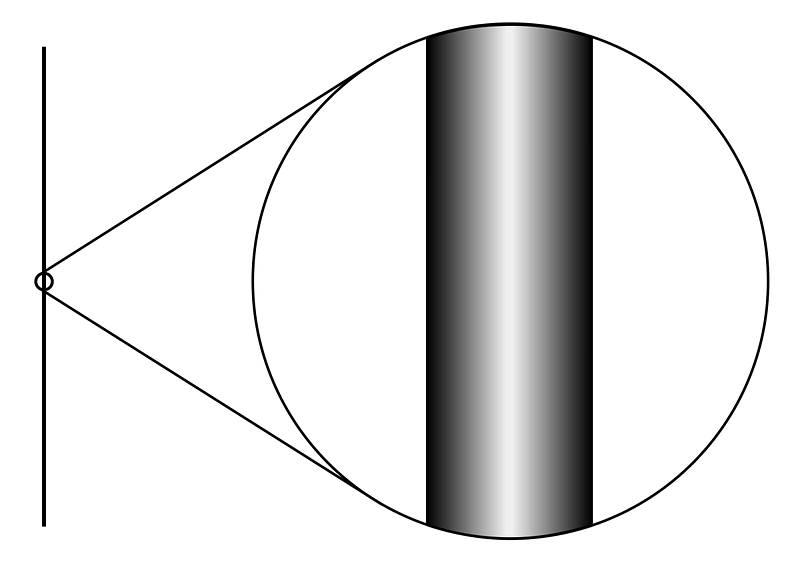
We normally think about dimensions of space by picturing a series of lines which spread from a point. How quickly the lines dilute with the distance from the point tells us the “Hausdorff dimension” of a space. The faster the lines diverge from each other with distance, the larger the Hausdorff dimension. If you speak through a pipe, for example, sound waves spread less and your voice carries farther. The pipe hence has a lower Hausdorff dimension than our normal 3-dimensional office cubicles. It’s the Hausdorff dimension that we colloquially refer to as just dimension.

For dimensional reduction, however, it is not the Hausdorff dimension which is relevant, but instead the “spectral dimension,” which is a slightly different concept. We can calculate it by first getting rid of the “time” in “space-time” and making it into space (period). We then place a random walker at one point and measure the probability that it returns to the same point during its walk. The smaller the average return probability, the higher the probability the walker gets lost, and the higher the number of spectral dimensions.

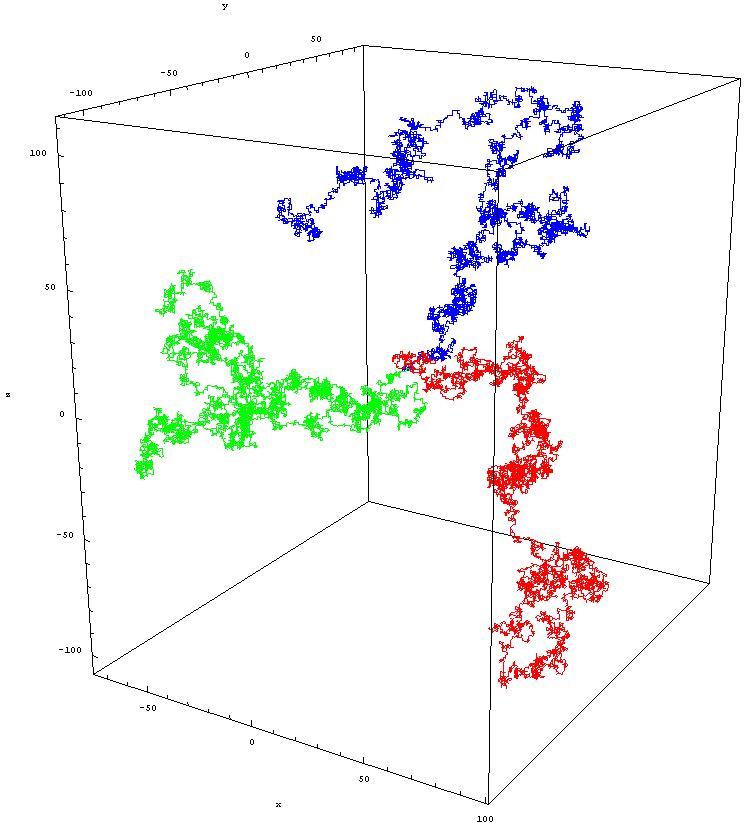
Normally, for a non-quantum space, both notions of dimension are identical. However, add quantum mechanics and the spectral dimension at short distances goes down from four to two. The return probability for short walks becomes larger than expected, and the walker is less likely to get lost — this is what physicists mean by “dimensional reduction.”

The spectral dimension is not necessarily an integer; it can take on any value. This value starts at 4 when quantum effects can be neglected, and decreases when the walker’s sensitivity to quantum effects at shortest distances increases. Physicists therefore also like to say that the spectral dimension “runs,” meaning its value depends on the resolution at which space-time is probed.

Dimensional reduction is an attractive idea because quantizing gravity is considerably easier in lower dimensions, where the infinities that plague traditional attempts to quantize gravity go away. A theory with a reduced number of dimensions at the shortest distances therefore has a much higher chance to remain consistent, and therefore to provide a meaningful theory for the quantum nature of space and time. Not so surprisingly, among physicists, dimensional reduction has received quite some attention lately.

This strange property of quantum-spaces was first found in Causal Dynamical Triangulation, an approach to quantum gravity that relies on approximating curved spaces by triangular patches. In this work, the researchers did a numerical simulation of a random walk in such a triangulized quantum-space, and found that the spectral dimension goes down from four to two. Or actually, to 1.80 ± 0.25, if you want to know precisely.

Instead of doing numerical simulations, it is also possible to study the spectral dimension mathematically, which has since been done in various other approaches. For this, physicists exploit that the behavior of the random walk is governed by a differential equation — the diffusion equation (a.k.a., the heat equation) — which depends on the curvature of space. In quantum gravity, spatial curvature has quantum fluctuations, so instead it’s the average curvature value which enters the diffusion equation. From the diffusion equation, one then calculates the return probability for the random walk.

Through this method, physicists have inferred the spectral dimension also in Asymptotically Safe Gravity, an approach to quantum gravity which relies on the resolution-dependence (the “running”) of quantum field theories. And they found the same drop as in Causal Dynamical Triangulations: from four to two spectral dimensions.

Another indication that dimensional reduction might be important comes from Loop Quantum Gravity, where the scaling of the area operator with length changes at short distances. In this case, is somewhat questionable whether the notion of curvature makes sense at all on short distances. Ignoring this philosophical conundrum, one can construct the diffusion equation anyway, and one finds that the spectral dimension –surprise — drops from four to two.

And finally, there is Horava-Lifshitz gravity, yet another modification of gravity which some believe helps with quantizing it. Here too, dimensional reduction, from four to two, has been found.

It is difficult to visualize what is happening with the dimensionality of space if it goes down continuously, rather than in discrete steps as in the example with the laundry pile. Perhaps a good way to picture it, as Calcagni, Eichhorn and Saueressig suggest, is to think of the quantum fluctuations of space-time as hindering a particle’s random walk, thereby slowing it down. It wouldn’t have to be that way, though. Quantum fluctuations could have also kicked the particle around wildly, thereby increasing the spectral dimension rather than decreasing it. But that’s not what the math tells us.

One shouldn’t take this picture too seriously though, because we’re talking about a random walk in space, not space-time, and so it’s not a real physical process. Turning time into space might seem strange, but it is a common mathematical simplification which is often used for calculations in quantum theory. Still, it makes it difficult to interpret what is happening physically.

I find it intriguing that several different approaches to quantum gravity share a behavior like this. Maybe it is a general property of quantum space-time? But then, there are many different types of random walks, and while these different approaches to quantum gravity share a similar scaling behavior for the spectral dimension, they differ in the type of random walk that produces this scaling. So maybe the similarities are only superficial.

And, of course, this idea has no observational evidence speaking for it. Maybe never will. But one day, I’m sure, all the math will click into place and everything will make perfect sense. Meanwhile, have another.

This post first appeared at Forbes, and is brought to you ad-free by our Patreon supporters. Comment on our forum, & buy our first book: Beyond The Galaxy!