Ask Ethan #57: How will Black Holes die?
The densest, most massive objects in the Universe will live an awfully long time, but not forever. Here’s what happens to them.
Image credit: Gemini Observatory/AURA illustration by Lynette Cook.
“Sit down before fact as a little child, be prepared to give up every preconceived notion, follow humbly wherever and to whatever abysses nature leads, or you shall learn nothing.” –T.H. Huxley
When you think of black holes, you probably think of these ultra-dense, incredibly massive regions of space from which nothing can escape. Not matter, not antimatter, not even light! You probably also think of how they continue to feed on whatever has the misfortune to run into them, even including dark matter. Yet at some point, every black hole in the Universe will not only cease to grow, but will eventually decay, shrinking and losing mass until it evaporates completely! For this week’s Ask Ethan, where we solicit your submissions, we take on Paweł Zuzelski’s inquiry, which asks:
I often see explanations of Hawking radiation along these lines: “pair of virtual particles appears on the event horizon. One particle falls into the hole, the other one escapes carrying some of hole’s mass away”. Usually there is a fine print saying this is a simplification. Indeed it must be an oversimplification — if one of the particles falls into the black hole, its mass should increase by the mass of the particle. Where is the catch?
This is an incredibly complicated topic, but one which we do actually understand. Let’s start, then, with talking about what empty space is like.
In general relativity, space and time are intricately related, forming the four dimensional fabric of spacetime. If you took all the particles in the Universe and moved them infinitely far away from the region we were in, if you took the fact that space was expanding out of the equation, if you took out all forms of radiation as well, and you took out any intrinsic curvature to space itself, you’d be well within your rights to claim that you had created flat, empty space.
Only, when you start considering that we live in a Universe where quantum field theory governs all the particles and their interactions, you have to recognize that even if there are no physical particles present, the physical fields that govern their interactions are still there. And one of the consequences of this is that what we think of as “flat, empty space” isn’t some constant quantity devoid of all energy. Instead, it’s better to think of flat, empty space as the quantum vacuum, where these quantum fields are everywhere.
You may be familiar with the idea that on quantum scales in the Universe, we find that there are inherent uncertainties when it comes to certain quantities. We can’t simultaneously know both the position and the momentum of a particle, and in fact the better we measure one of them, the greater the uncertainty that results in the other. This same uncertainty relation applies to energy and time as well, and this one is particularly relevant.
You see, if you’re looking at what you think is completely empty space, but you’re looking at it for one particular instant of time, you’ve got to remember that an instant is an infinitesimally small amount of time. Because of this uncertainty relation, there’s therefore a large uncertainty in the total amount of energy in your (even empty!) space at this time. This means that there could, in principle, be a number of particle/antiparticle pairs that exist for the briefest of instants at any given time, so long as they obey all the known conservation laws of the physical Universe.
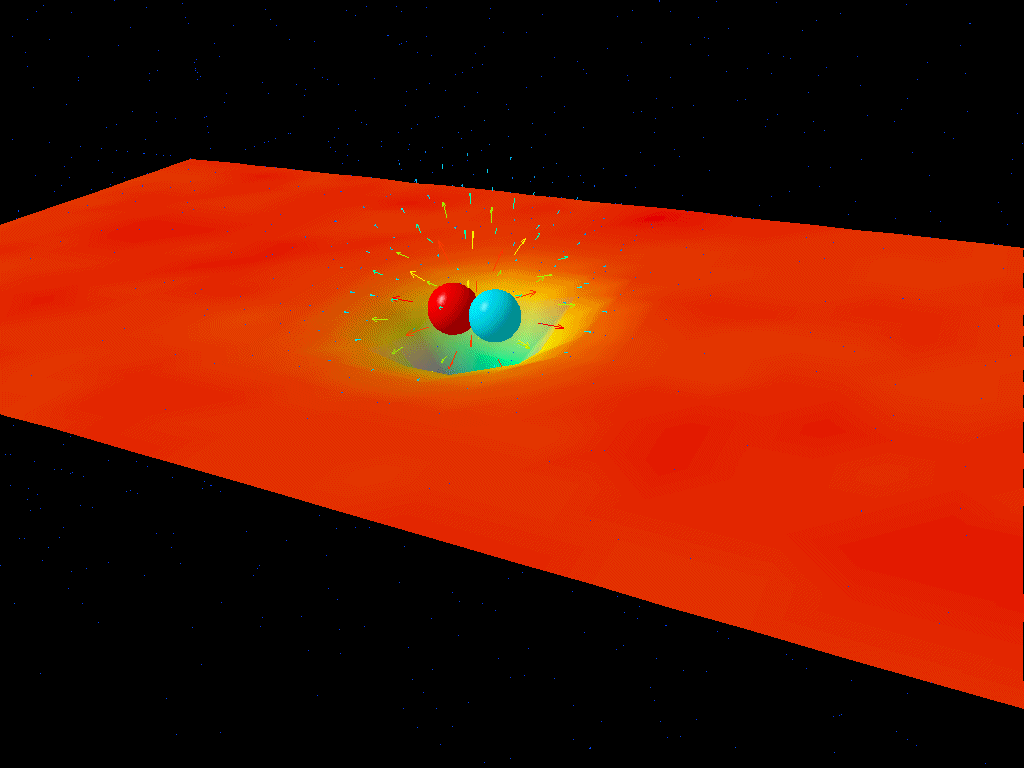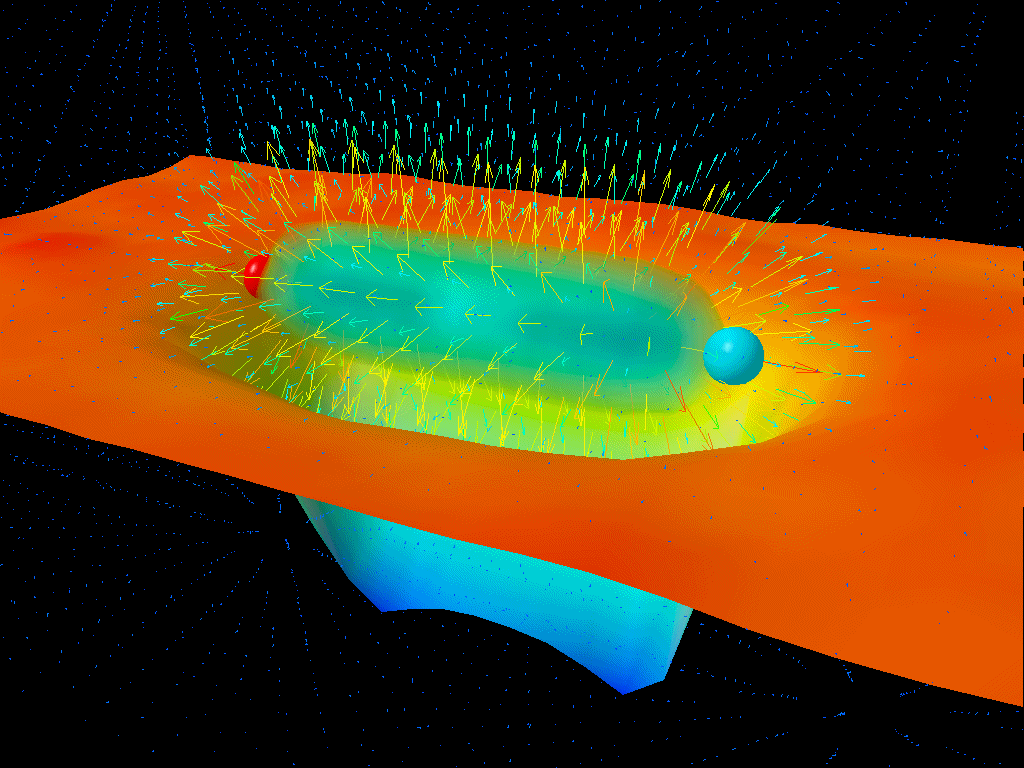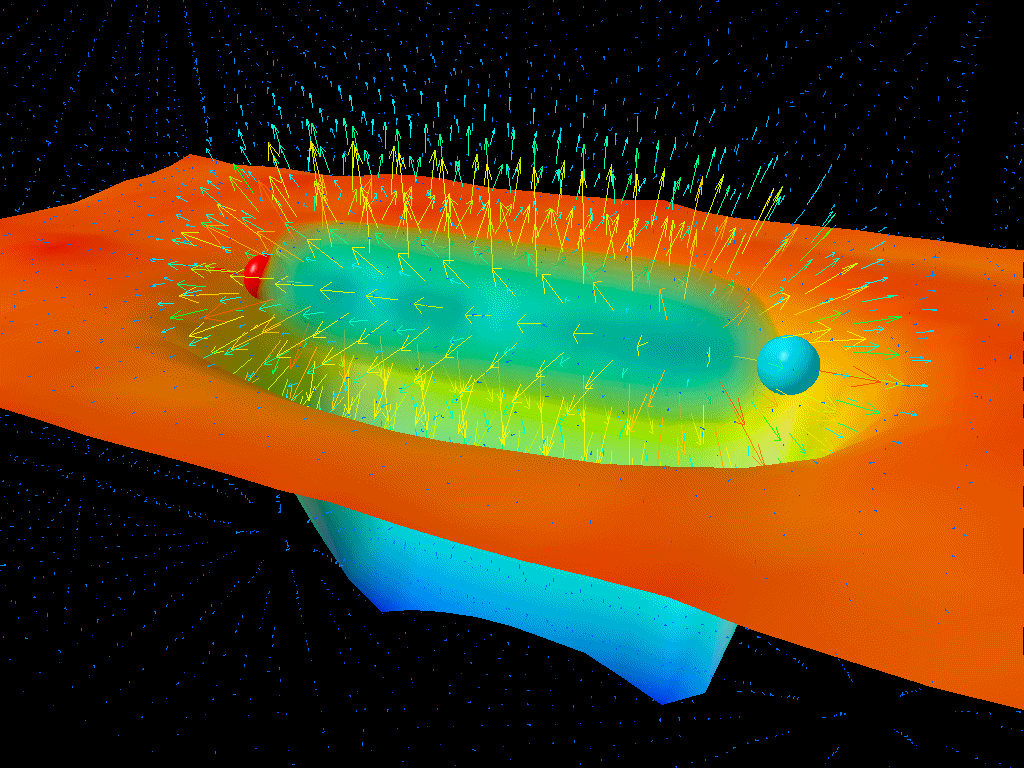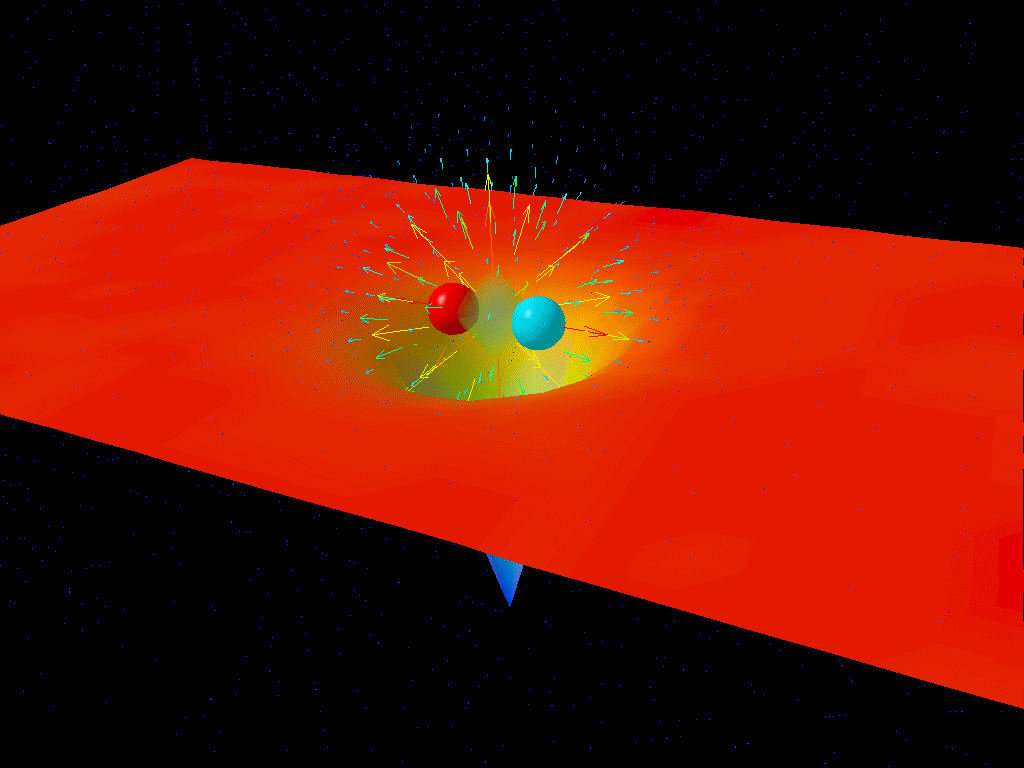
We often hear this described as “particle-antiparticle pairs popping in-and-out of the quantum vacuum,” and while that provides a nice visual, it isn’t really what’s happening. These aren’t real particles in the sense that, if you shoot a photon or an electron through that region of space, it’s never going to bounce off of this quantum vacuum particle. Instead, this gives us a window into the inherent “jittery-ness” of the quantum vacuum, and illustrates for us that there’s a reservoir of virtual particles that allows us to treat the energy inherent to empty space itself as the sum of all these virtual particles.
I’ll say that again because it’s important: there is an energy inherent to empty space itself, and if we think about all the quantum fluctuations inherent to that space and sum them up, that’s where the energy comes from.
Now, let’s go one step further. Let’s imagine, instead of space being completely flat and empty, let’s imagine that it’s still empty, but that it’s curved, meaning that there’s a gradient in the gravitational field of space.
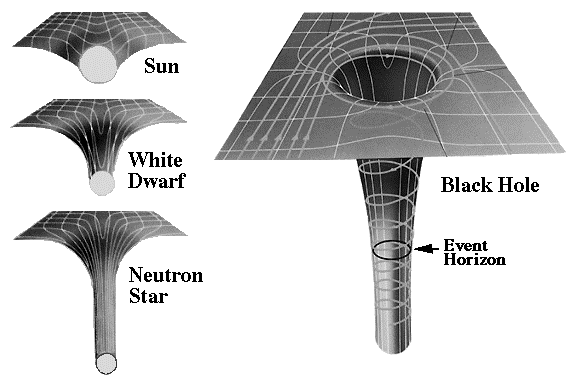
What are these quantum fluctuations going to look like, now? And in particular, if we allow space to be curved due to the presence of a black hole, what are those fluctuations going to look like inside and outside of the event horizon?
These are good questions to ask, and the most common picture you see is the (misleading) one below, which is pretty much the essence of what Paweł is asking about.
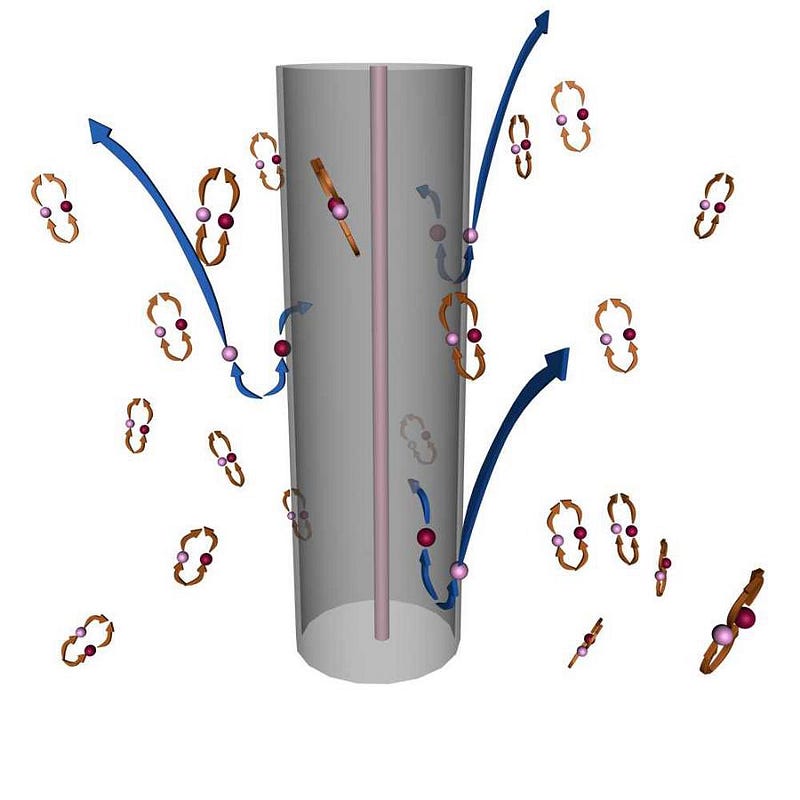
If you think of particle/antiparticle pairs as being “real” things, and if one escapes from the black hole’s event horizon and the other one falls in, then you’d expect to have just added energy to the Universe: half outside of the black hole and half to the mass of the black hole. But these pairs of particles and antiparticles aren’t real things, they’re only ways of visualizing (and calculating) the energy inherent to space itself.
The thing is, when your space is curved, remember we said there’s a gradient to the gravitational field. We do have these fluctuations that help us visualize the inherent energy to empty space, but there are going to be fluctuations that begin outside of the black hole’s event horizon that fall inside the event horizon before they can re-annihilate. But you can’t steal energy from empty space like that; something needs to happen to conserve it. So every time a virtual particle (or antiparticle) falls in, a real photon (or set of photons) needs to come out to compensate. And that real photon leaving the event horizon is how energy gets carried away from a black hole.

The way we naively looked at it earlier, of one set of a particle/antiparticle pair falling in with another escaping is too naive to be useful, since it isn’t particles or antiparticles that cause the decay of black holes, but rather photons following a blackbody spectrum.
A better picture (that I prefer) that’s still a little naive is to imagine that you have these quantum fluctuations, but that every time you have a pair of particle-antiparticles where one falls in, you have a corresponding particle-antiparticle pair where the other falls in. The particle/antiparticle pair on the outside annihilate, emitting real, energetic photons, while the ones that fall in take a corresponding amount of mass (via E = mc^2) away from the black hole.
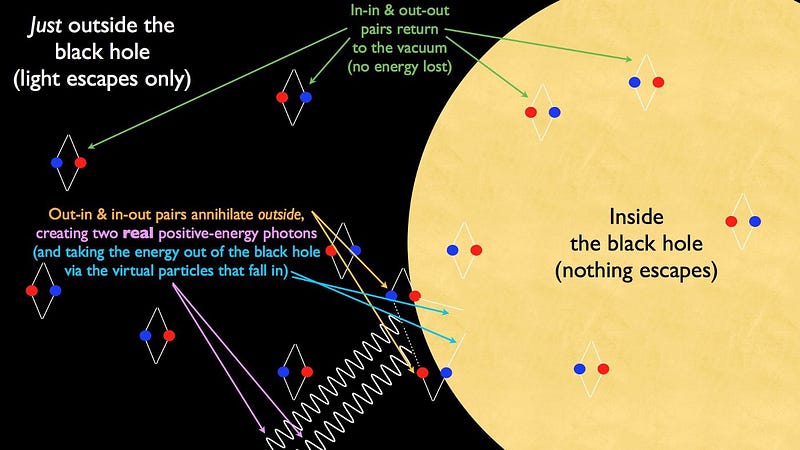
It’s still not a perfect analogy (because it’s still an analogy), but at least it’s photons leaving the event horizon of the black hole this time, which is exactly what Hawking radiation predicts. In fact — although you actually have to do the quantum field theory calculations in curved spacetime to find this out — Hawking radiation predicts that you’ll get a blackbody spectrum of photons with a temperature given by:
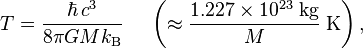
which is a temperature of less than one microKelvin for a black hole the mass of our Sun, less than one picoKelvin for the black hole at the center of our galaxy, and just a few tens of attoKelvins for the largest known black hole. These decay rates that this radiation corresponds to are so small that it means that black holes will continue to grow so long as they continue to absorb even one proton’s worth of material per present-age-of-the-Universe, which is estimated to occur for the next 10^20-some-odd years.
After that, black holes the mass of the Sun will finally start to lose more energy due to Hawking radiation (on average) than they’ll absorb, completely evaporating after ~10^67 years, and with the largest black holes in the Universe disappearing after around ~10^100 years. That may be far longer than the age of the Universe, but it’s still not forever. And the way it will decay is through the mechanism of photon emission via Hawking radiation.
In summary: empty space has a zero-point energy that isn’t zero, and in curved space, that causes a very low-energy spectrum of black-body radiation to be created right at the black hole’s event horizon. That radiation takes mass from the central black hole and causes the event horizon to slightly shrink over time. If you insist on thinking of the source of this radiation as particle/antiparticle pairs, please at least think of two pairs at a time, allowing the particle from one and the antiparticle of the other to annihilate, creating real photons that leave the black hole, and allowing the (virtual) pair that falls in to take the energy (or mass) away from the black hole itself.
And that’s how black holes will eventually die! Thanks for a great question, Paweł, and if you have questions or suggestions, send them in here. The next Ask Ethan column might be based on you!
Leave your comments at the Starts With A Bang forum on Scienceblogs!