All About Cosmic Inflation
What everyone should know about where our matter-and-radiation-filled Universe came from.
“I don’t think at this point we have any way of knowing where the laws of physics came from. We could hope that when we really understand the laws of physics that they will describe how the Universe came into existence.”
-Alan Guth
There’s a flurry of information coming out about the Big Bang and inflation, from bloggers, news outlets, science publications and scientists themselves. The wikipedia page on inflation is being rapidly updated as well, and misconceptions and misunderstandings are flying around, far outnumbering the rare sources that get the majority of the story right. In the aftermath of the release of the foundation-shaking results of the BICEP2 collaboration, there’s now a great opportunity for the world to understand exactly what we know about the origin of the Universe, how it developed, and — if the new discovery stands up to independent confirmation — what we will have learned.
Let’s start at the beginning.

In the early 20th century, our understanding of the Universe underwent a number of incredible and important revolutions. The slight deviations of the planet Mercury’s orbit from Isaac Newton’s predictions led Einstein to develop his general theory of relativity, which wound up predicting not only the observed orbital deviations, but many other things.
One of them was that mass actually caused spacetime to curve in a particular fashion, and that light that had to travel near a massive object would have its path bent as a result. This was the first new prediction of general relativity to be observationally confirmed, as stellar positions during a total solar eclipse appear shifted from when the (massive) Sun isn’t in their vicinity in the sky!

But while a theorist like Einstein was revolutionizing our understanding of gravitation, observers were revolutionizing our understanding of the most distant objects known to humanity. In particular, these “spiral nebulae” which could be seen through telescopes had some rather remarkable properties that we were just beginning to uncover.

The vast majority of these nebulae — now known to be galaxies not so unlike our own Milky Way — have very large redshifts, which either means they’re moving away from us very quickly, or that the space in between us and them is expanding. When Edwin Hubble successfully determined the distances to these galaxies in the 1920s, he found that the farther away a galaxy was from us, the larger its redshift was. This combination of the redshift data, Einstein’s relativity and the galactic distance scale led to the conclusion that the Universe was expanding, and that the distances between objects on the largest scales was increasing as the Universe aged.
There were a number of possible things this could mean for the Universe, but one of them — first proposed by Georges Lemaître and later expanded upon by George Gamow — was that the Universe began from a state of arbitrarily small size, high temperature and high density. It’s only the vast, cold and relatively empty place it is today because of the great amount of time that’s elapsed since its birth!
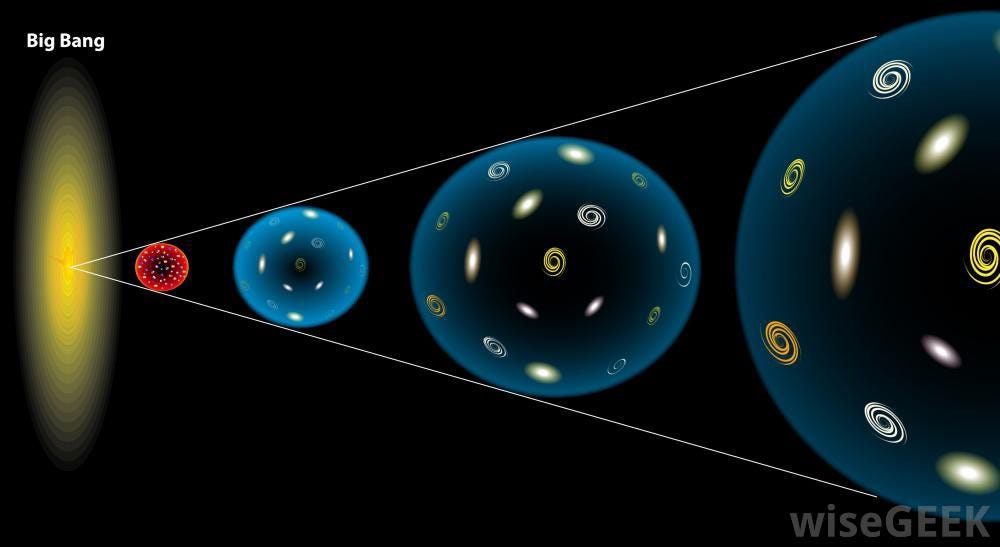
This idea is what’s known today as the original Big Bang theory. Let’s take a look at what this entails. Think about what our Universe is today: a vast, cosmic web of galaxies, with huge centralized clusters loosely connected by filaments, with vast cosmic voids in between them. The clusters, clumps and groups of galaxies that are gravitationally bound to one another will stay that way, but all the more distant ones are caught up in the expansion of the Universe, and will continue to get more distant as the Universe ages.
Only the galaxies within a few million light years of us are bound to us today; the vast majority of the rest are receding from us. But in the framework of the Big Bang, there’s a reason for this. The very fabric of space itself expands as time goes on, and that expansion rate is determined by the amount of matter and energy present in space, as well as the amount that space is intrinsically curved.
If we imagine the Universe further back in time, it was smaller, all the matter was closer together (and so it was denser), and — because the wavelength of light, which stretches with the expansion of the Universe, determines its temperature — the Universe was also hotter and more energetic in the distant past!
This means that we can, in principle, extrapolate backwards to as early a time as we want, and learn something about where our Universe came from. Because all the matter in our Universe today (that we can easily detect) is made up of atoms, and radiation above a certain energy will ionize atoms, there must have been a point in the Universe’s distant past — when things were so hot and so dense — that any neutral atoms that formed would have immediately been blasted apart back into nuclei and electrons!
But we can go even further back in time: there must have been a time where the radiation was so energetic that even atomic nuclei would have been blasted apart into protons and neutrons, and then even farther back when protons and neutrons would have been dissociated into quarks and gluons, and so on. As Lemaître himself originally suggested all the way back in 1927, the Universe could have originated from a “primeval atom” that was arbitrarily hot and dense, and possibly even infinitely so.
But it was Gamow and his collaborators who first began working out the details of this in the 1940s and 1950s. In particular, when the Universe finally did cool enough to form individual protons and neutrons, and then atomic nuclei, and then neutral atoms, certain signatures should be left over from those times. In particular, that last one — when it cooled enough to form neutral atoms — should mean that whatever radiation was left over from the early Universe at that moment should finally stop running into the ionized particles (mostly electrons) and should simply continue traveling through the Universe.
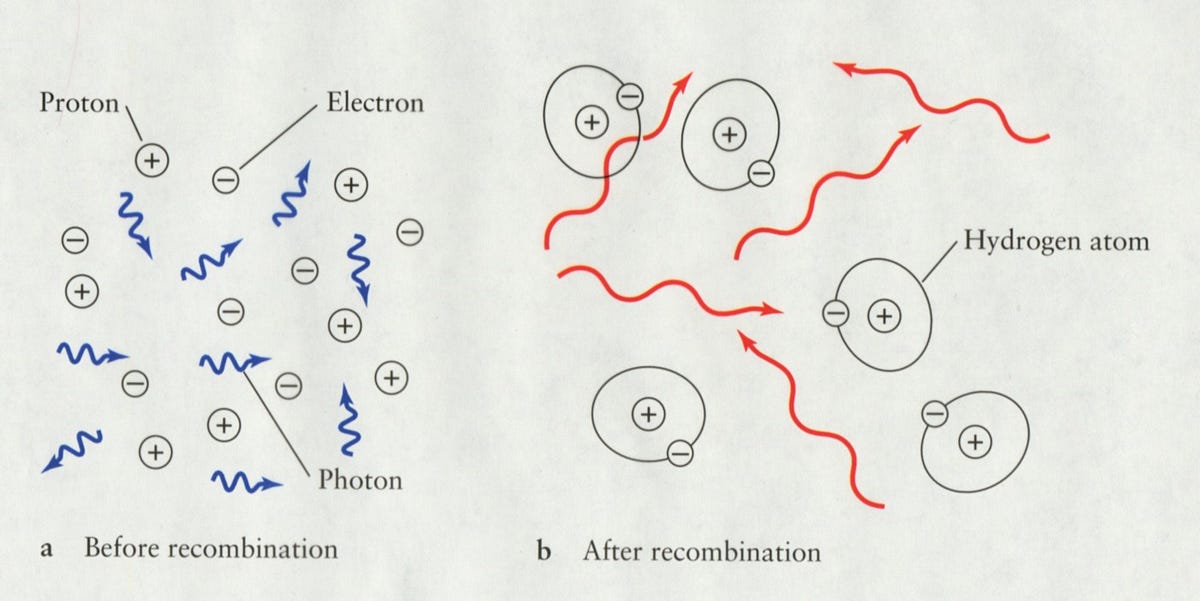
Its wavelength should increase (and it should become lower in energy) as the Universe expanded, and it should be just a few degrees above absolute zero by now. In particular, it should be approximately the same temperature in all directions, and it should appear everywhere on the sky. This relic of the “primeval fireball” should — if we look at the proper wavelengths of light — be visible everywhere we look in the Universe.
And in 1964, Arno Penzias and Robert Wilson discovered that leftover glow from the Big Bang, cementing it as the most accurate, predictively powerful theory describing the early Universe.
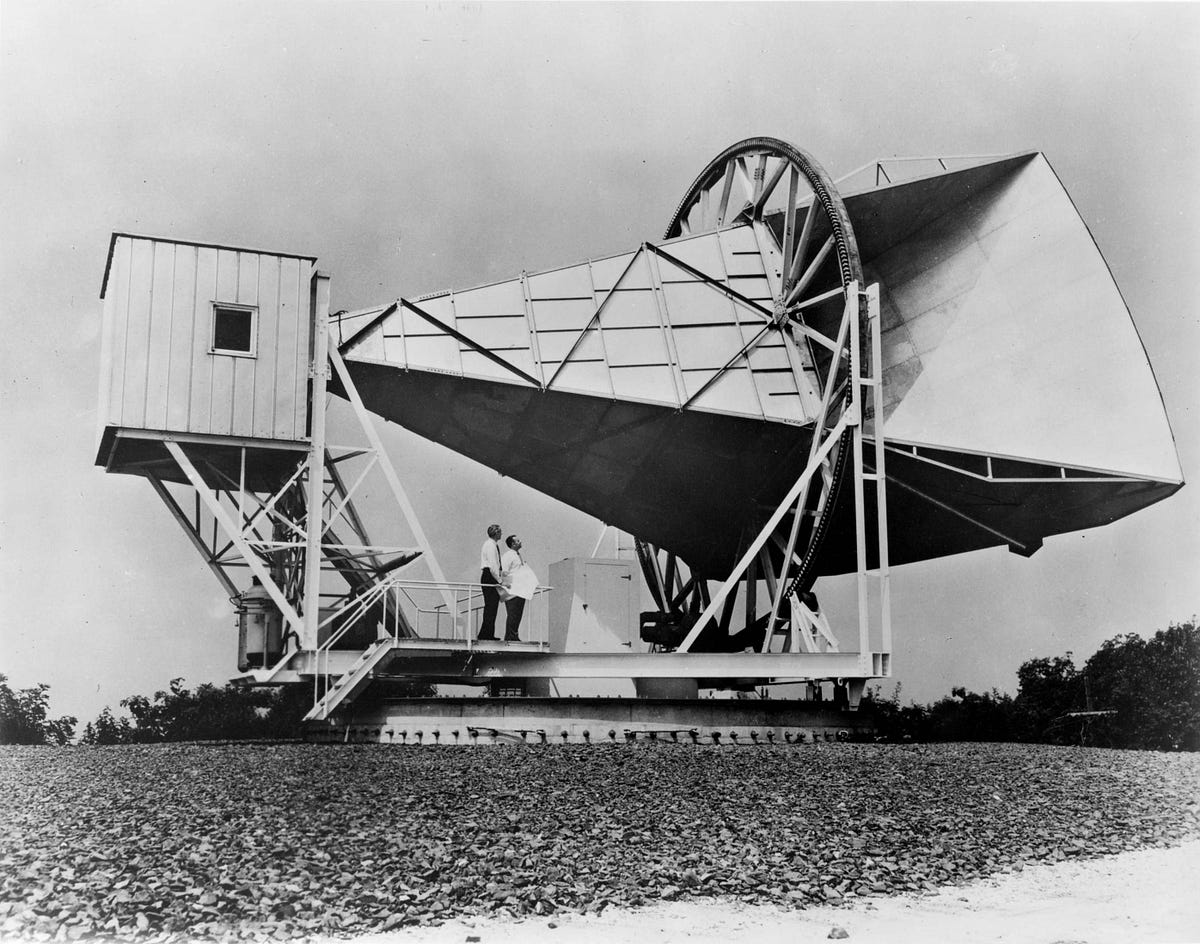
Subsequently, other confirmations of the Big Bang’s predictions came in as well: the lightest elements in the Universe — deuterium, helium-3, helium-4 and lithium-7 — were observed in the abundance predicted by nucleosynthesis in the early Universe. The way galaxies clustered and clumped together was consistent with a Universe that began more uniformly and then became more clustered as gravity had more time to group things together. The temperature of the very distant Universe was found to be warmer, consistent with a Universe that was still expanding and cooling. And the very distant, neutral atoms from before the Universe had an opportunity to form stars and galaxies in many locations was itself discovered: pristine gas left over from the Big Bang.
But there were some puzzles as well; some things that we observed that the Big Bang couldn’t explain.
For starters, if the Universe was, at some point in the past, at arbitrarily high energies, there should be all sorts of ultra-high energy relics left over from that time. Theoretical particles like magnetic monopoles, leftover signatures from grand unification, topological defects like cosmic strings and domain walls, etc. All of these should have left signatures in our observable Universe; particle signatures on small scales, and signatures in the large-scale structure of the Universe on bigger ones. Yet, when we look for these signatures, there are none.
Something was fishy. And yet there were more surprises.
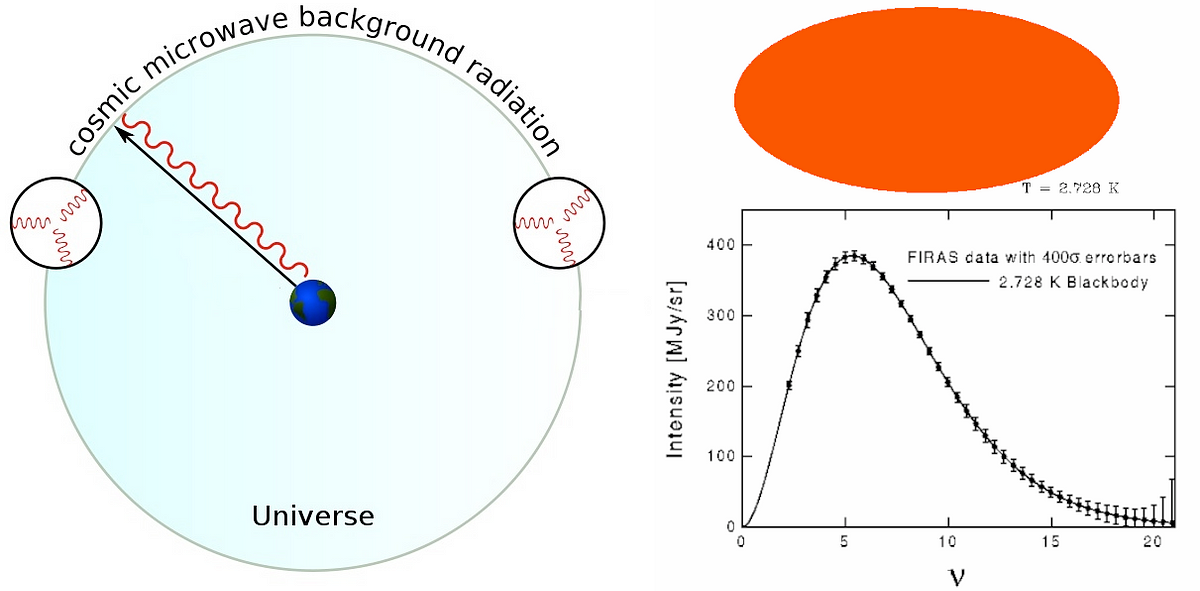
The leftover glow from the Big Bang was uniform. As in, really, really uniform; much more uniform than it had any right to be. This is unexpected for the following reason. If you turn on the heater in one corner of a room, the entire room will eventually heat up, but it will take some time. Why? Because the warmed air needs to exchange the heat energy it possesses with the cooler air elsewhere in the room, and that takes time and interactions. Until that exchange happens, we expect there to be a temperature gradient, and for there to be relatively warmer and colder regions.
Well, the Universe hasn’t had time for regions on opposite sides to interact or exchange any information, much less energy. There haven’t been interactions that should have brought it into thermal equilibrium, or a uniform-temperature state. What we would have expected is that some regions of space would be twice as warm (or cold) as others, but what we find is that space is of a uniform temperature to a few parts in 100,000.
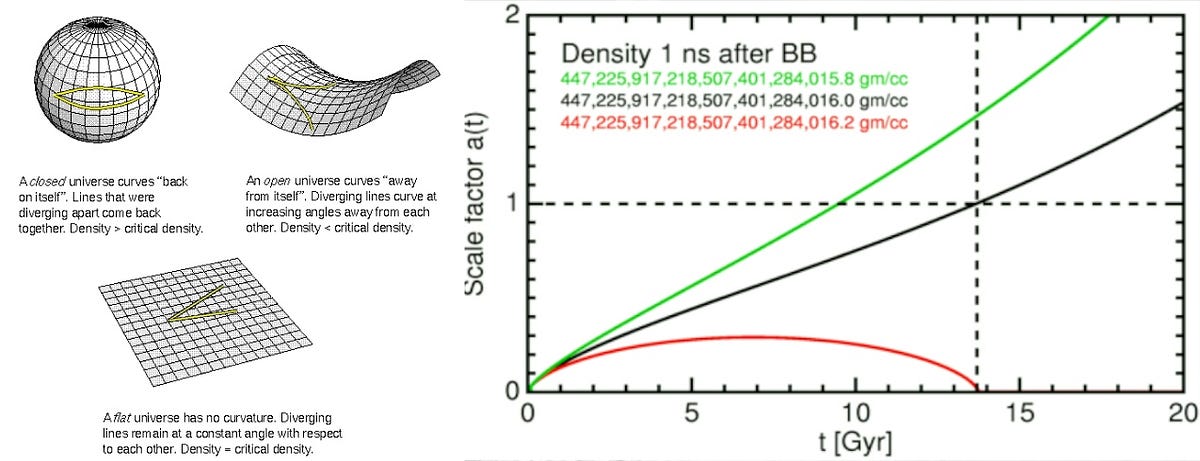
And finally, there was one more big one. The Universe’s expansion rate over time, remember, was determined by the matter and energy present, and also by the amount that space was intrinsically curved. And to the best of our measurements, the Universe appeared to not be curved at all. This isn’t necessarily a problem, but the level of fine-tuning in the Universe’s initial conditions required to achieve a result like this is phenomenal; the total energy density needed to be exactly the value it is to about one part in 10^28 in order to arrive at the curvature-free Universe we observe today.
Now it’s possible that “this is just the way the Universe is” and there’s no further explanation, but that’s not how science progresses! The way we learn more about the Universe is we ask ourselves whether there’s a theoretical phenomenon that could account for this behavior, and if so, what are the other observational or experimental consequences and predictions of such a theory?

You must understand that these problems and puzzles are only difficulties if you insist that we extrapolate back to those arbitrary high energies and temperatures. If, instead, we allow for the possibility that we can’t extrapolate back to the highest energies and temperatures and densities and smallest scales possible, but instead theorize that something else happened to cause and set up the hot, dense, expanding, matter-and-radiation-filled Universe, we can not only solve these problems, but figure out what came before the Big Bang framework is applicable.
And that’s exactly what the theory of cosmological inflation says. It says that prior to the Universe being described by the matter-and-radiation-filled, expanding state we have today, it went through a period where there was practically no matter or radiation, and instead the Universe was dominated by energy inherent to space itself, and expanded exponentially!
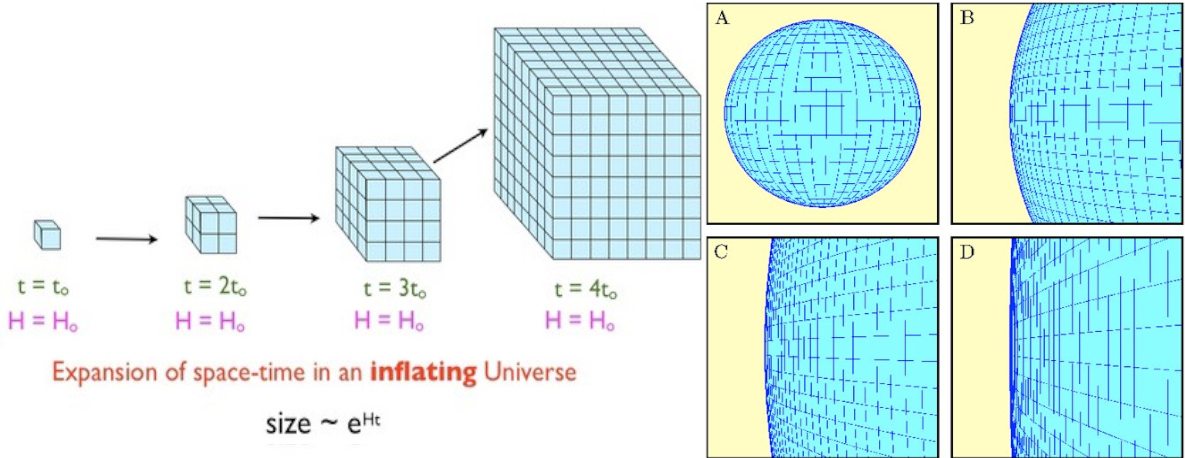
This means that the region of space that today consists of what we call “our Universe” — of which our observable Universe is just a tiny part — was once contained in an arbitrarily small region of space. Whatever matter or radiation pre-existed in that region was inflated away; exponential expansion stretches the Universe so that no two particles should ever meet.
If there were any high-energy, high-temperature particles, topological defects, or other curiosities, inflation pushed them out so that — at most — there would be one contained in the entire observable Universe. If there were regions of space that had different temperature properties, they are now separated by at least trillions of light years, and if the Universe had any intrinsic curvature to it, it was stretched by inflation to be indistinguishable from “flat” today.
In other words, inflation solves all of the aforementioned problems! But, can it solve those problems while still:
- reproducing all of the appropriate initial conditions of the Big Bang,
- being framed in a way that’s mathematically and physically consistent with all known existing physics, and finally (and most importantly),
- making new, testable predictions about what we should see in the Universe?
The answer is “yes” to all three, but it took a while to get there. What follows is going to be detail-oriented, but you deserve the details. Here we go!(And if you want to skip the details, look for this symbol: ☆★☆)
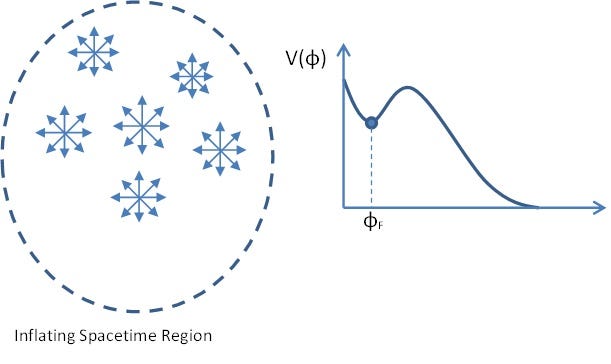
Alan Guth’s initial formulation was to treat inflation as a quantum scalar field, which is the simplest type of field that’s consistent with all the physics and mathematics of the Universe. It’s an outstanding choice, because it allows you to explore the possibilities of what can happen without the messy (or at least, messier) physics of more complicated physical systems. (You can come up with models of multi-field inflation, quantum-gravity-inspired inflation, string theory inflation, etc., but you don’t learn anything new by doing so.)
Guth suggested a field like the one above, where spacetime started out in that false minimum; being high above the bottom of where your zero-point-energy lies means your space is undergoing the rapid, exponential expansion required by inflation. But inflation can’t last forever or our Universe wouldn’t be here! So he hypothesized that — since it’s a quantum field — it can undergo quantum tunneling, and enter the stable non-inflating state via a standard, quantum process.
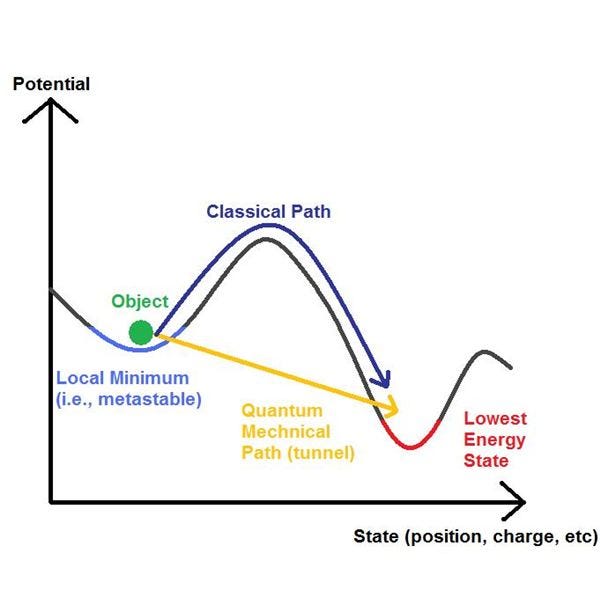
It’s a pretty good attempt, especially since this was the very first paper ever written about inflation! Unfortunately, this would have resulted in an empty Universe, where all the energy of that empty space was transferred into the walls of our “bubble” of space where inflation ends. Since all the space around our bubble would still be inflating, we’d never find another bubble, and therefore we’d never get our observable Universe out. In other words, inflation — in this first model — would never have ended properly to give us our Universe with the Big Bang in it.
We needed a graceful exit to that inflationary state, and that was discovered independently by Andrei Linde and by the team of Paul Steinhardt and Andy Albrecht.
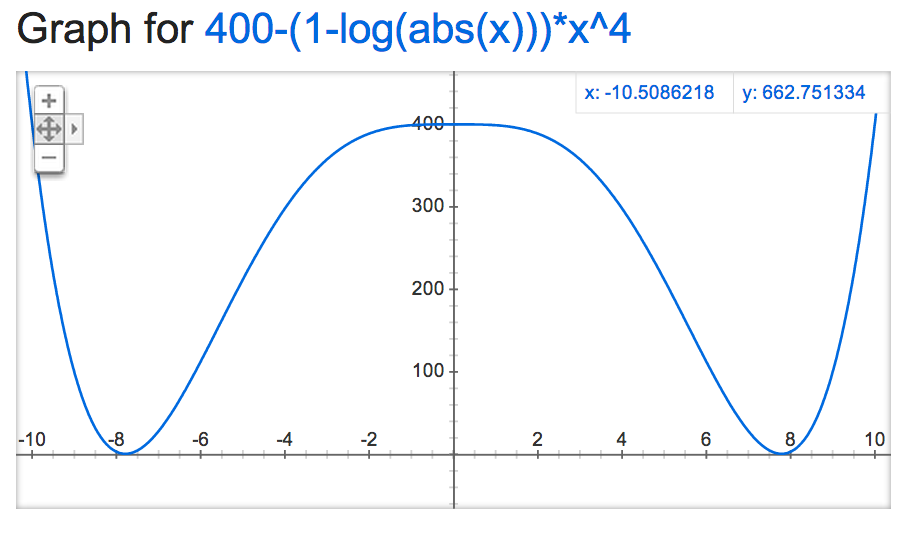
Instead of having a potential that required tunneling, you could have a potential where you were atop a very (but not perfectly) flat hill. While you remained atop that hill — or off of the bottom in general — your Universe was inflating, but as you eventually rolled down into the minimum, inflation ends everywhere, gradually, converting all of that “energy of empty space” into matter-and-radiation.
That’s the hot Big Bang! This solution became known as “new inflation” (and Guth’s original model became known as “old inflation”), and reproduced all the known conditions of the early Universe while simultaneously solving all of the problems with an arbitrarily hot, dense and small Universe. Whenever anyone says that the Big Bang comes before inflation, they are very likely missing this important part of the story!
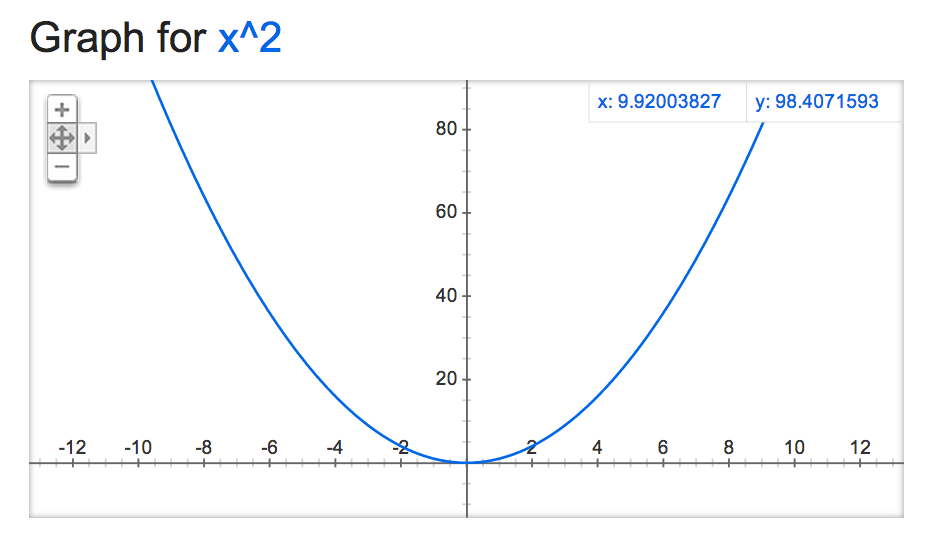
There’s also another way to have a successful round of inflation in the early Universe, and this one doesn’t necessarily rely on starting at an unstable place on a particularly flat scalar field potential. Instead, you can make the assumption that a variety of initial field values are likely, and assume whatever potential you like. There are only a few conditions necessary — given a scalar field — for inflation to occur, and a wide variety of potentials can work. Even the humble parabola, above, will work just fine, so long as you assume these chaotic initial conditions, and allow the field to not necessarily start at the center, but anyplace.
As time goes forward, the regions which wind up inflating the most, which is the regions farthest away from the center in this example, will very quickly encompass the overwhelming majority of the Universe. Andrei Linde, who was one of the discoverers of “new inflation,” also discovered this version of inflation with chaotic initial conditions — known as chaotic inflation — and ushered in an era where we realized that a huge variety of inflationary potentials could give rise to a Universe like ours.
So, which of the inflationary models that we can think up will be correct? In order to discriminate between them, we needed to figure out what observable phenomena would be connected to these potentials. If this were a classical field, and all you were was a ball that rolled down a hill, nothing interesting would happen. You’d inflate while you were high up away from the zero-point, and then inflation would end as you rolled down to the bottom.
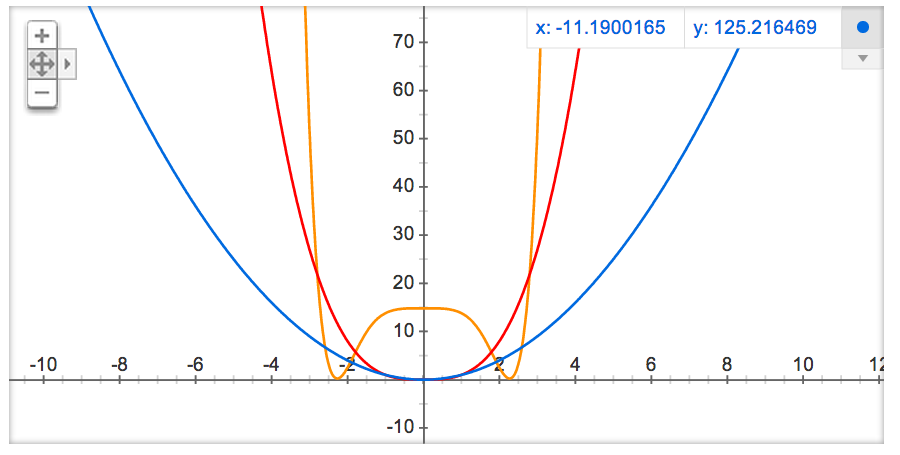
But because this is a quantum field, it exists in (and couples to) spacetime, which means it produces quantum fluctuations! These fluctuations translate into new predictions! Specifically, inflation produces scalar fluctuations, which leads to tiny variations in density across various scales in the Universe, and also tensor fluctuations, which leads to gravitational waves. As inflation nears its end — during the last few fractions of a second before reheating and the Big Bang — the fluctuations produced at that time get stretched across what is today our observable Universe.
But how do those fluctuations get produced?
You can draw any curve (or potential) you like that leads to inflation, and then look at two things at the location on the curve near the end of inflation:
- What is the slope of the curve near the end of inflation?
- How quickly is that slope changing at that location?
If the slope were perfectly flat and unchanging, you’d get a perfectly scale-invariant spectrum of density fluctuations, and no gravitational waves. Both the slope and how its changing contribute to the spectrum of density fluctuations (the flatter both of them are, the closer the spectrum is to scale invariant), and the faster the slope is changing, the larger the gravitational waves are. We actually got our first look at the data on density fluctuations from the COBE satellite in the 1990s, and here were the results.
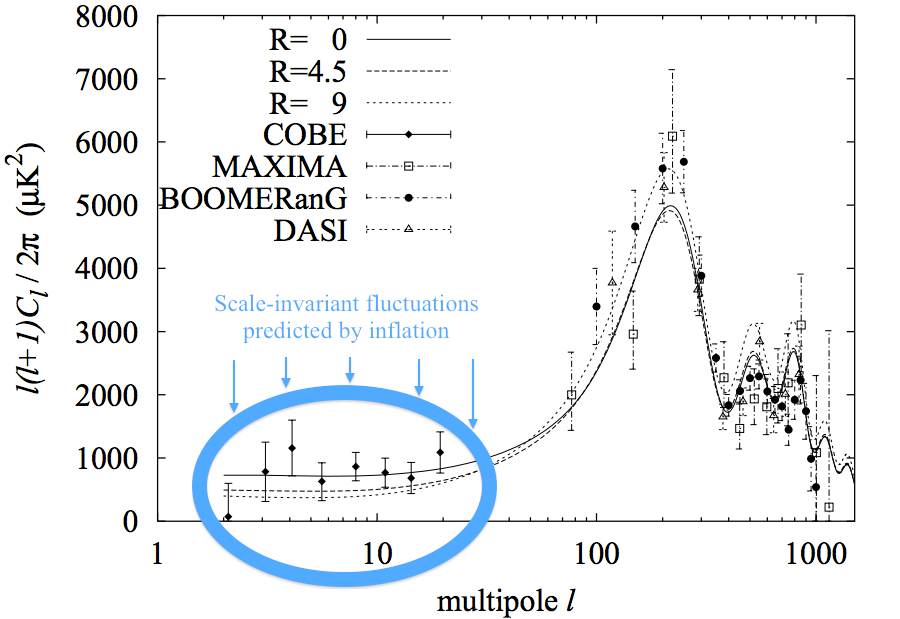
It’s very close to scale invariant — which means the best-fit curve on the graph above is very close to being perfectly flat before it starts its upswing — but not quite! In other words, this was consistent with a number of models of inflation, including both the “new inflation” model but also with a number of Linde’s chaotic models, including the simple parabola.
But if we could detect the signature of gravitational waves, that would be thing that allowed us to tell different models apart! In particular, the ratio of gravitational wave perturbations to density perturbations — something that we simply call r in cosmology — is the big differentiator between many of these models.
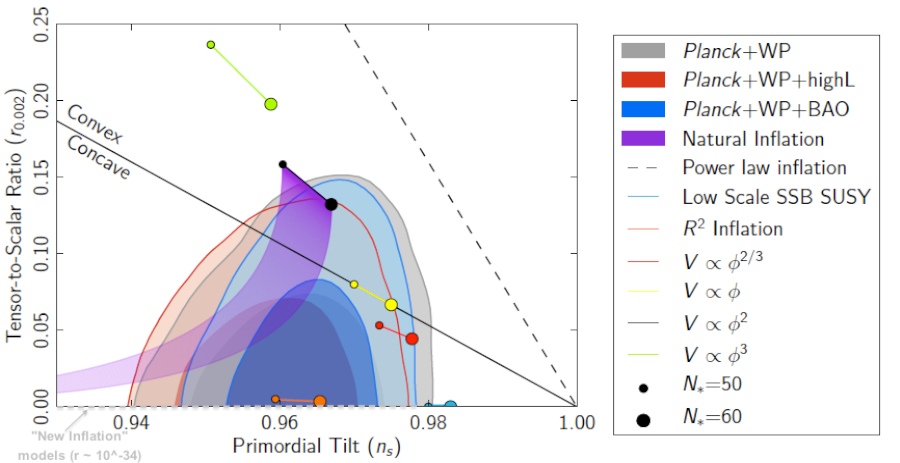
After the first major results from the Planck satellite were released, it looked like the “new inflation” models were favored, as the non-detection of gravitational waves combined with that almost scale invariant spectrum (where n_s = 1 would be perfectly scale invariant) would favor models of new inflation. Linde’s parabola, by the way, is the black barbell in the graph above.
(☆★☆ — If you wanted to skip the details on inflation, welcome back!)
But Planck doesn’t have their polarization data done yet, and polarization is where the gravitational wave signature best shows up.
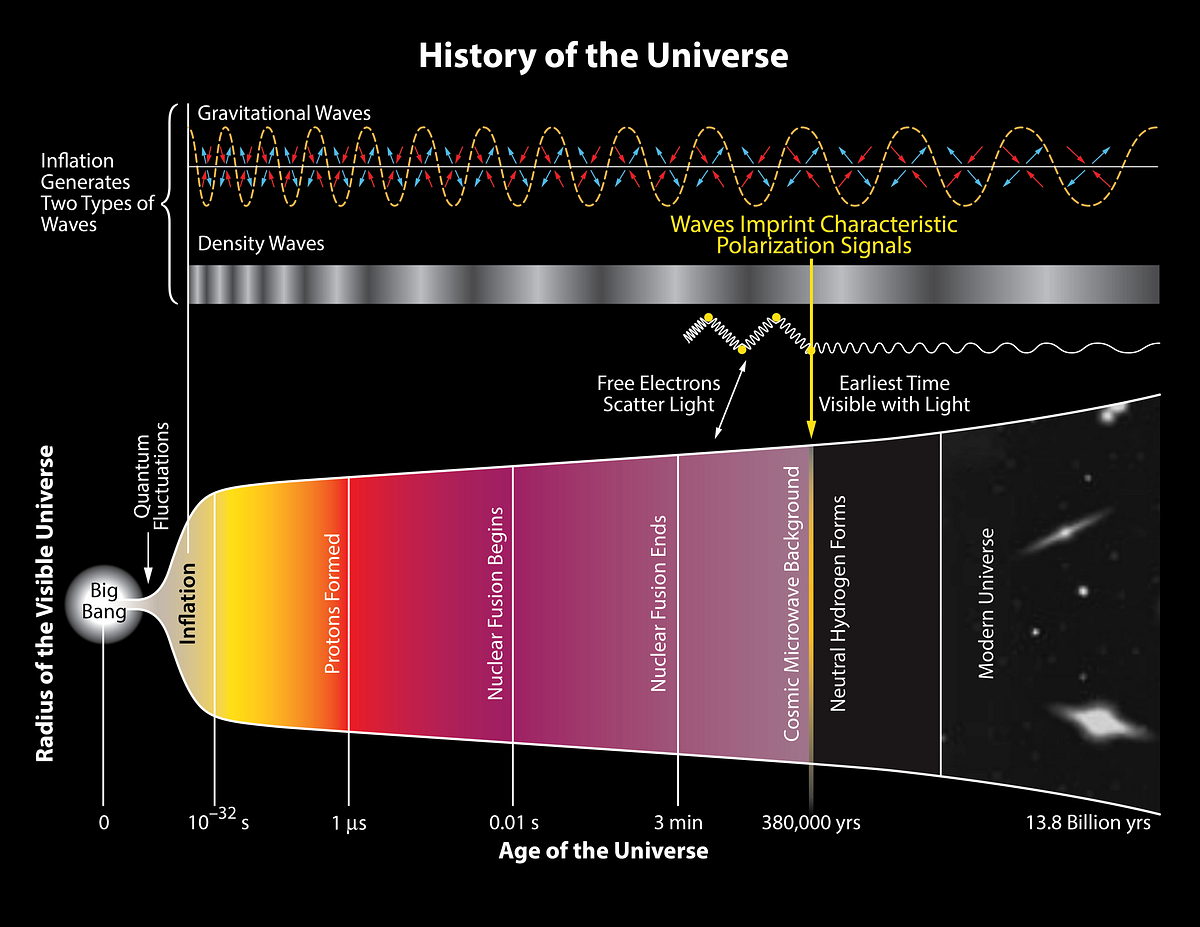
Note that this diagram messes up the Big Bang coming after inflation on their timeline of events in the Universe.
But there are other experiments that are all competing to measure exactly that: the polarization data that could give us a window into whether there were gravitational waves produced during inflation! Those gravitational waves — if they exist — would be imprinted on the B-mode polarization signature of the cosmic microwave background, which itself is the leftover glow from the Big Bang!
Well, to date, there were only null results reported. But the BICEP2 collaboration — after checking their results for over a year — finally released the first claimed detection of B-mode polarization in the Cosmic Microwave Background!
Although it’s very, very important to check this independently (and there should be many checks coming over the next two years), here’s what they found.
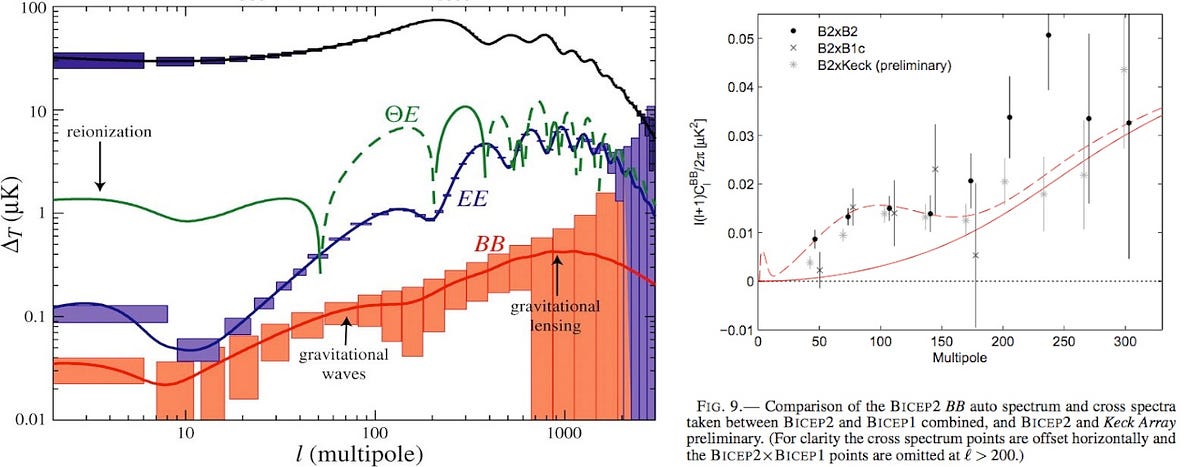
And if we look at the overall, best-fit data from the BICEP2 collaboration, what do we find?
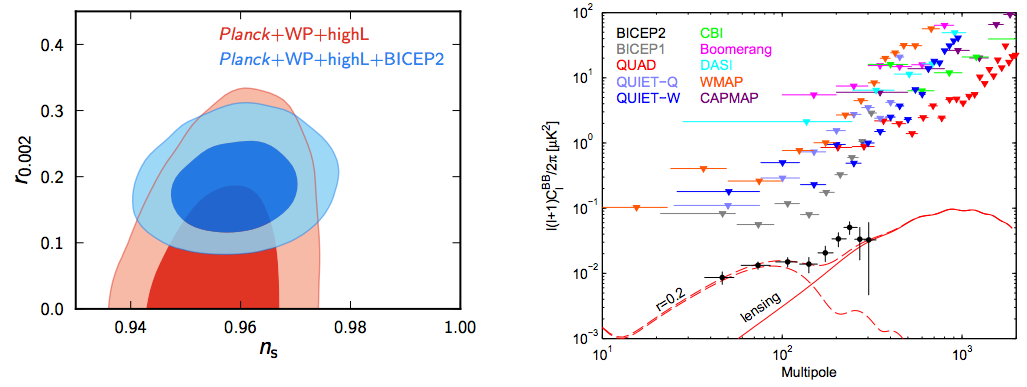
We find that r, the tensor-to-scalar ratio, the ratio of gravitational waves from inflation to density fluctuations from inflation, is large, as in, around 0.2, and that the fit is pretty good, although on smaller angular scales (at larger values of l, or multipole number) there is some unexplained deviation. But it’s an amazing result, and if it’s substantiated, it’s the discovery of the century (so far) for cosmology!
So if this result holds up, what does it mean?
It means that not only can we be even more sure that there was a period of cosmic inflation preceding the Big Bang, it means we can begin to tell what kind of inflation we had. It means we can start constructing more accurate and more sophisticated models, and learn how this period of exponential expansion ended, and gave rise to our hot, dense, expanding Universe. It means that Guth, Linde, and probably the principal investigator of the BICEP2 collaboration are in line for Nobel Prizes.
And it means that we should be building LISA — the Laser Interferometer Space Antenna — to detect these waves directly. Because even though this is a great moment for science and for cosmology, it’s also the beginning of a new era in our understanding of the Universe: one with gravitational waves left over from before the Big Bang!
Have a comment? Head over to the Starts With A Bang forum at Scienceblogs!