Black hole myth busted: they don’t suck anything in

- Black holes are the densest objects in the Universe, with at least several Suns worth of mass collected in a region that’s so small, even objects moving at the speed of light can’t escape from it.
- Although these objects exert a tremendous gravitational force, they don’t “suck matter in” any differently than neutron stars, white dwarfs, stars, or planets do: they just gravitate normally.
- Despite the common picture of black holes sucking everything in from their surroundings, that’s not how they work at all. Black holes don’t suck; that’s just the most common myth about them.
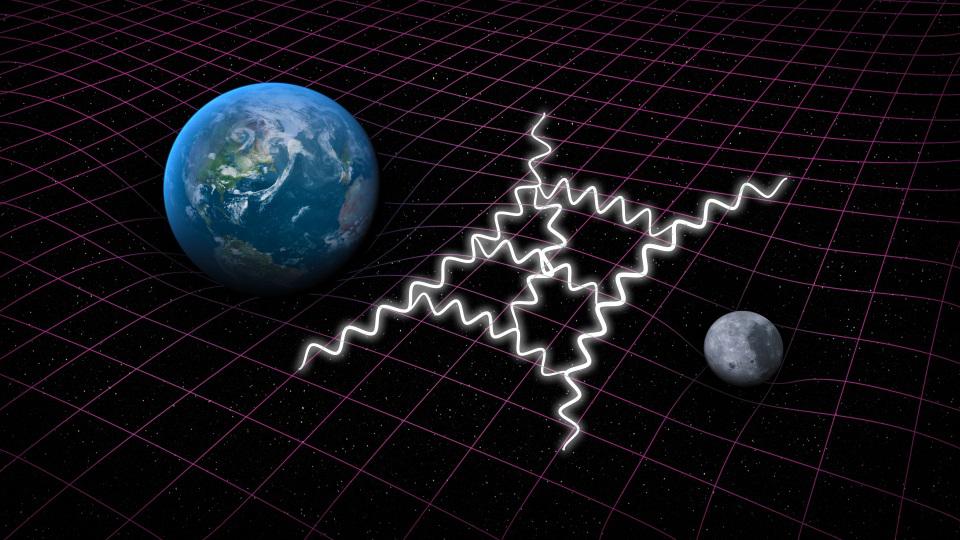
Black holes are some of the strangest, most wondrous objects in all the Universe. With huge amounts of mass concentrated into an extremely small volume, their interiors inevitably collapse down to singularities, surrounded by event horizons from which nothing — not even light — can escape. In terms of ranking objects by density, black holes are the densest objects known to exist within the entire Universe. Whenever anything passes too close in the vicinity of a black hole, the forces from the black hole will tear it apart into its constituent particles. If any particles of matter, antimatter, or radiation ever cross over the event horizon, those quanta will simply fall down into the central singularity, where they cause the black hole to grow by adding to its total mass.
These properties about black holes are all true. But if you ask people to tell you their conception of a black hole — including what it is and what it does — you’ll often find that there’s an idea associated with black holes that’s absolute fiction: that black holes suck any surrounding matter into them. This couldn’t be further from the truth, and completely misrepresents how gravity works. The biggest myth about black holes is that they suck. Here’s the scientific truth behind how they work instead.
Both in principle and in practice, there are many different ways to create a black hole. You can create one by having a large, massive star go supernova, where the sufficiently massive central core of that star implodes and forms a black hole. You can witness two neutron stars merge, where if they cross a specific mass threshold (where their total mass exceeds about 2.5-2.7 solar masses) the post-merger object that results will create a newly-formed black hole. Or you could have a large collection of matter — a supermassive star or converging streams of cold, contracting gas — collapse down directly to a black hole with no associated cataclysmic event.
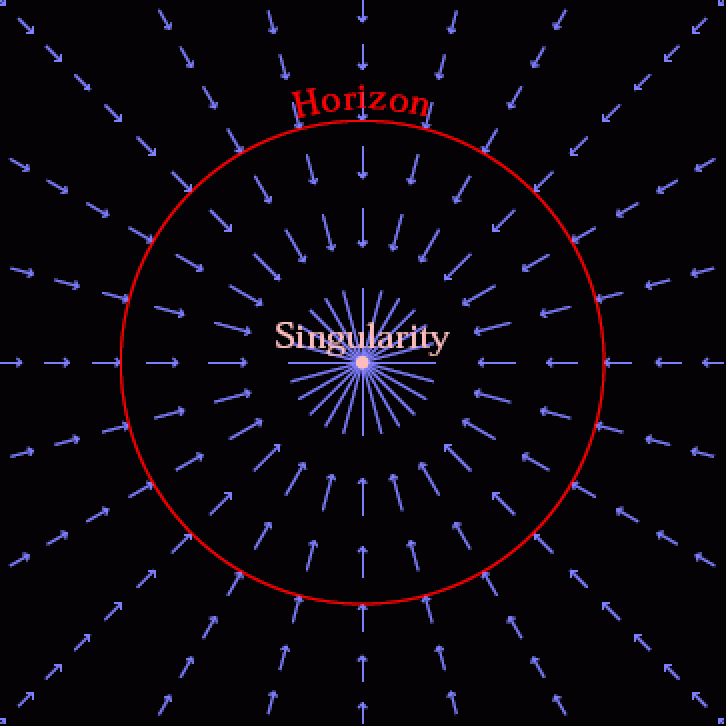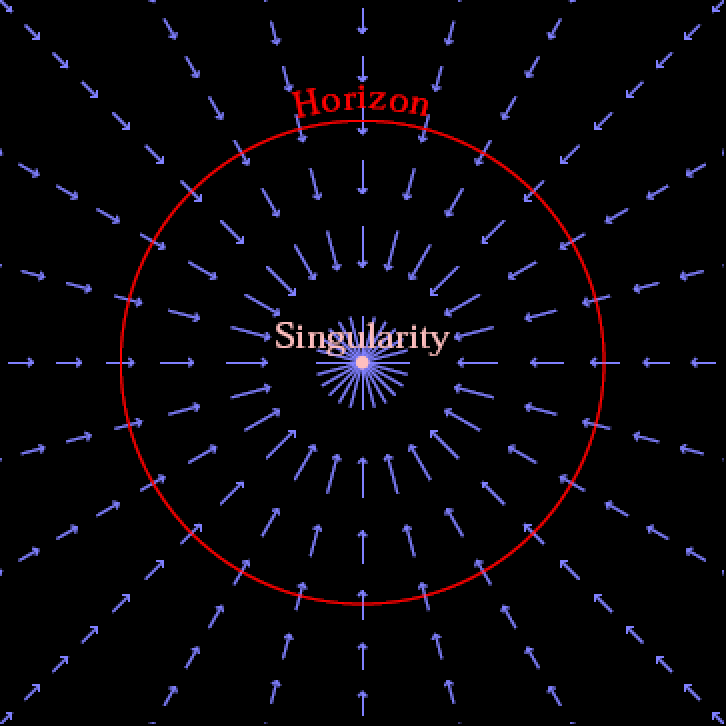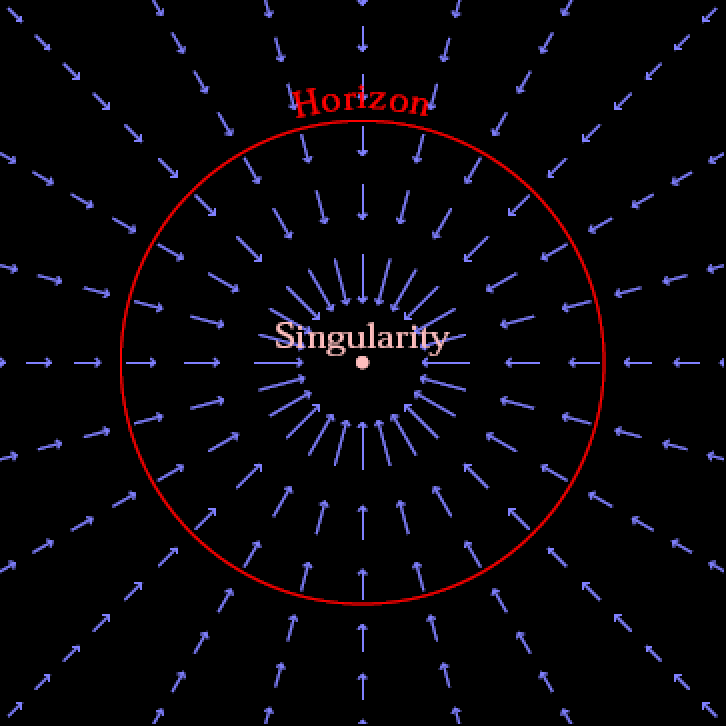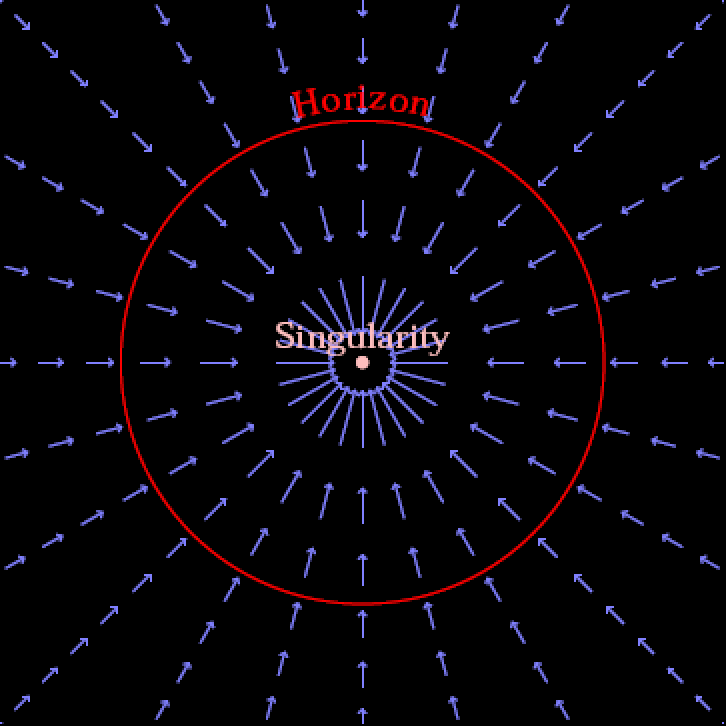
Once you’ve accumulated enough mass within a concentrated enough volume of space, an event horizon will form in a region surrounding that mass. From outside the event horizon, you can still escape if you move away from the black hole at speeds that are at or below the speed of light: speeds that are physically possible for objects to attain. Yet, if you were located within the event horizon, then even if you were moving at the speed of light in a vacuum, the ultimate cosmic speed limit, any path you could take would still lead you inevitably toward the central singularity. There is no escape from within the event horizon of a black hole; once you cross over to the interior, you’re doomed to encounter the central singularity.

For objects that remain outside of the black hole’s event horizon, however, there’s still plenty of trouble brewing. Because black holes are such massive objects but also such compact objects, when you get close to one, you start to experience a significant set of tidal forces acting on you. Most of us aren’t very familiar with the notion of tidal forces except in one instance: the tidal forces arising from the Moon interacting with the Earth.
Most of us don’t think about where our tides come from very often: the fact that, at the continental boundaries with the oceans, the oceans appear to rise (when the tide comes in) and fall (when the tides go out) twice per day. For the most part, they’re due to the presence of our Moon (and, to a lesser extent, partly due to the Sun as well).
On average, it’s not a bad approximation to treat the Moon as a point mass and the Earth as a point mass, separated by the relatively large distance of 380,000 kilometers or so. But when you fold in a slightly deeper layer to reality — the fact that the Earth isn’t a point, but an object that occupies a real, substantial volume — you’ll realize that the components of the Earth that face the Moon are actually closer to the Moon than the parts of Earth that face away from the Moon, whose components will be farther away. The parts of Earth that are closer to the Moon will experience a greater gravitational attraction to the Moon than average; the parts that are more distant than average will experience a lesser attraction than average.
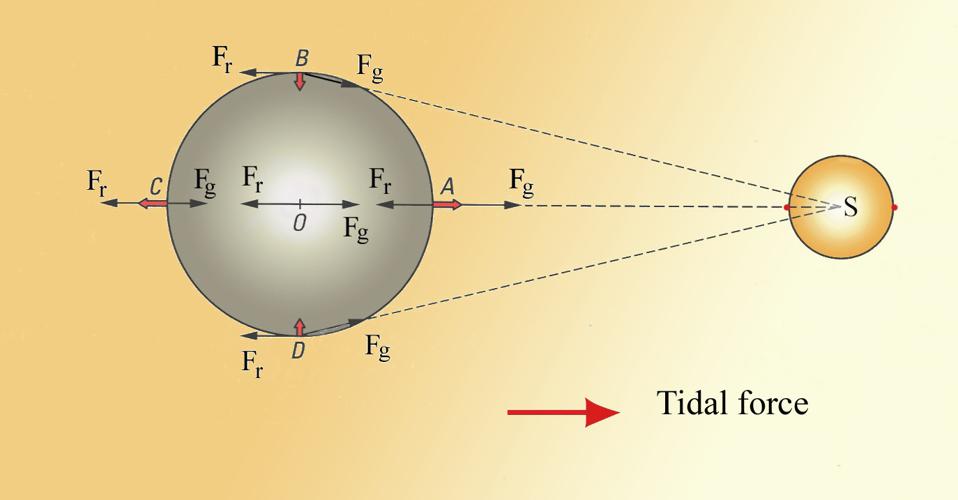
In addition to having “closer” and “farther” parts, we have to remember that the Earth isn’t a one-dimensional object that’s lined up with the imaginary line connecting the Earth to the Moon, but rather is a fully three-dimensional spheroid. If we consider the Earth as a three-dimensional entity, we have to realize that there are both “top” and “bottom” areas of the Earth (from the Moon’s point of view) as well as “left“ and “right“ areas of the Earth, and those areas will get pulled inward, toward the center of the Earth, relative to the portions of Earth that are located closer to the middle of that imaginary line connecting the Earth to the Moon.
What we can do to better visualize this is to consider the gravitational force exerted by the Moon on every point on the Earth, and then to subtract out the average force (i.e., the force on the center of the Earth) that the Moon exerts, gravitationally. This shows us how all the various points on the surface experience the external forces from the Moon differently. The “force lines” that result map out the relative forces an object experiences, and explain why objects that experience tides get:
- stretched along the direction of the “average” force,
- while also getting compressed in all directions that are perpendicular to the direction of that “average” force.

You already know that the closer you get to an object, the larger the gravitational force on you gets. Someone here on the surface of the Earth accelerates down toward the Earth’s center at 9.8 m/s² (32 ft/s²). If you were twice as far away, such as in orbit around the Earth at a distance of ~6371 km (one Earth radius) from the surface, the gravitational force on you would only be one-quarter of what it is on our planet’s surface, and you’d accelerate down toward the center of the Earth at just 2.45 m/s² (8 ft/s²).
You might then assume that the closer you get to a massive object, the larger these tidal forces would become. That’s correct, but the tidal forces get larger even faster than the gravitational force does: if you halved the Earth-Moon distance, the tidal forces wouldn’t be four times as strong, but eight times as strong!
Because black holes are both extremely massive and extremely compact, they are the objects capable of generating the largest-known tidal forces in the Universe. This is why, as you approach a black hole, you find yourself getting “spaghettified,” or stretched into a thin, noodle-like shape: due to the extreme tidal forces. It’s easy to see how this phenomenon leads to the misconception that black holes would suck you in: the closer you get to one, the stronger the attractive force of gravity gets and the stronger the tidal forces that work to tear you apart get as well.

Still, the idea that you’ll get sucked into a black hole isn’t based in reality; it’s solely a misconception about how forces (and black holes) work. It’s true that every single particle that makes up any object near a black hole is going to be affected by the black hole’s gravity. However, those particles are still subject to the same laws of physics as all other objects, including the laws of physics that arise from the gravitational curvature of spacetime generated by general relativity.
In the context of general relativity, the fabric of space is curved and bent by the presence of all forms of energy, including mass. It’s also true that black holes offer the greatest concentrations of mass found anywhere throughout the Universe. But what you might not intuit is this: it’s also true that the density of any mass that curves space is completely irrelevant for how space is curved, so long as your object is outside of the physical mass that’s curving space itself.
Consider this fact: if you were to replace the Sun with
- a white dwarf,
- a neutron star,
- or a black hole,
of the same exact mass as the Sun itself (one solar mass), the gravitational force that acted on the Earth would be no different from the force exerted by the modern-day Sun. It’s only the total mass that curves the space around you; density has practically nothing to do with it.

From a distance, a black hole behaves no differently from any other mass in the Universe. At large distances, it’s only mass that matters; how that mass is distributed has negligible effects on both gravitational and tidal forces. It’s only when you get extremely close to an object — within only a few Schwarzschild radii of it, or close to its event horizon — that you start noticing how a black hole departs from other masses, as well as how spacetime’s curvature departs from the predictions of Newtonian gravity. Even nearby a black hole, however, you’ll find that the black hole simply acts as an attractor, where objects that approach it make the same orbits they normally would around a mass: a circle, ellipse, parabola or hyperbola, depending on their speed and trajectory, to a very good approximation.
Because of the substantial tidal forces that arise from traveling so close to a large mass, approaching objects frequently get torn apart by a black hole’s gravity, creating a class of events known to astronomers as TDEs: tidal disruption events. Matter that gets torn apart by a black hole often winds up being accreted around it: in the form of an accretion disk. Within these disks, there might be additional effects present: magnetic fields, friction, and heating among them. Some of the matter that orbits the black hole, given these additional interactions, is likely to be slowed down and eventually swallowed by the black hole, even as the overwhelming majority of that matter still escapes: accelerated away from the black hole and funneled into outgoing jets.

It simply isn’t true that black holes create a “sucking” effect on any objects within the Universe at all. The fact of the matter is that black holes aren’t sucking anything in; there’s no force that a black hole exerts that a normal object (like a moon, planet, or star) doesn’t also exert. In the end, it’s all just gravity: the average gravitational force exerted on an object’s center-of-mass and the tidal forces that stretch, compress, and tear apart the object around its center-of-mass. The biggest difference between black holes and other massive objects is that black holes are:
- denser than most objects,
- occupy a much smaller volume of space than other objects,
- and are capable of being far more massive than any other single object in the Universe.
Saturn might be just fine orbiting our Sun as it is today: with the Sun’s mass and at the distance of Saturn from the Sun, it should remain stable for as long as the Sun fuses hydrogen into helium in its core: for many billions of years to come. However, if you were to replace the Sun with the black hole at the center of the Milky Way — a black hole that’s some 4,000,000 times as massive as the Sun — the tidal forces would destroy Saturn completely. Saturn would first be broken up into an enormous ring, where it would soon become part of the black hole’s accretion disk.

Credit: X-ray: NASA/CXC/CfA/R.Kraft et al Radio: NSF/VLA/Univ. of Hertfordshire/M.Hardcastle et al. Optical: ESO/VLT/ISAAC/M.Rejkuba et al.
Given enough friction, heating, and acceleration in the presence of the gravitational, electric, and magnetic fields that all the matter generates, eventually, a portion of that mass would fall inside and get swallowed, while the remainder would be funneled into bipolar jets that were emitted away from the black hole, perpendicular to Saturn’s original orbital plane.
This is the only context in which black holes even appear to suck matter in: as they absorb matter that undergoes gravitational infall due to the black hole’s mass, small size, and the close proximity of an infalling object to its event horizon. The combination of tidal forces and the matter already present around the black hole can indeed tear external objects apart, but it’s only a fraction of the torn-apart particles that will experience enough of a drag force to be funneled into the accretion disk and, potentially, into the black hole itself.
Even in this scenario, black holes are known to be messy eaters; the overwhelming majority of matter that passes close to a black hole will be spit back out and ejected in some form or another. It’s only the small portion of matter that falls inside the event horizon that ever causes black holes to grow and gain in overall mass.

Credit: University of Warwick/Mark A. Garlick
If we replaced any mass in the Universe with an equivalent-mass black hole, and we went further and removed all the frictional material (like accretion disks) around it, we’d find that very little mass would wind up entering the black hole. None of it would get “sucked in”, and only basic gravitational infall would lead to any mass entering the black hole at all. The only resistive “frictional effect” a particle would experience, i.e., an effect that changed its motion relative to standard gravitational infall, would be due to the emission of gravitational radiation (gravitational waves) as it moves through the curved spacetime generated by the black hole.
In terms of inevitably falling into a black hole, it’s only the material that formed interior to three times the event horizon radius — interior to what’s known as the innermost stable circular orbit (ISCO) in general relativity — that would inexorably get sucked into it. Compared to what actually falls into the event horizon in our physical reality, the purported “sucking” effects are nowhere to be found. In the end, we have only the force of gravity, and the curved spacetime that would result from the presence of these masses, affecting the evolution of objects located in space at all. The idea that black holes suck anything in is arguably the biggest myth about black holes of all. They grow due to gravitation, and nothing more. In this Universe, that’s more than enough to account for all the phenomena we observe.