Throwback Thursday: Fun Facts for Pi Day

This March 14th, stump your friends with these amazing facts about the world’s favorite transcendental number!

“So here we have π squared, which an engineer would call ‘10.’” –Frank King

Today, March 14th, is known tongue-in-cheek as Pi Day here in the United States, as 3.14 (since we write the month first) are the first three well-known digits to the famed number, π. As you know, it’s the ratio of a perfect circle’s circumference to its diameter.

It’s also practically impossible to calculate exactly, because it’s impossible to represent π as a fraction. (You may remember that’s part of the definition of an irrational number.) But that doesn’t mean we haven’t tried to get arbitrarily close!

The easiest way to try is to either inscribe or circumscribe a regular polygon around a circle of radius 1, and calculate the polygon’s area. The more sides you make, the closer you’ll get.

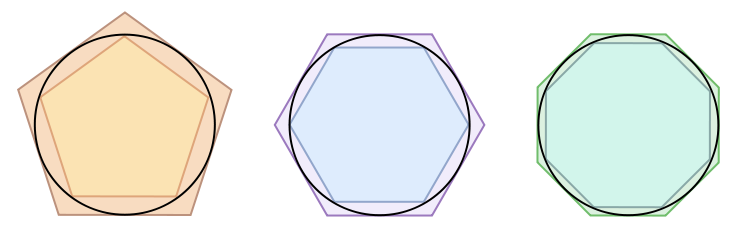
Archimedes, who discovered the fraction 22/7 (which is why Pi Day is July 22 in Europe), took the equivalent of a 96-sided polygon to do this, and found that π was between 220/70 and 224/71, which is not bad for over two thousand years ago!

But it’s hardly the most impressive approximation for π from the ancient world. That honor goes to the Chinese mathematician, Zu Chongzhi.

He discovered — in the 5th Century — the approximation Milü, which is 355/113. Which is equal to, for those of you at home, 3.1415929… meaning you have to go to the eighth digit to see the difference between this number and π, and that the difference is only 0.0000002667, or 8.49 millionths of a percent.

In fact, if we look at the best fractional approximations of π…

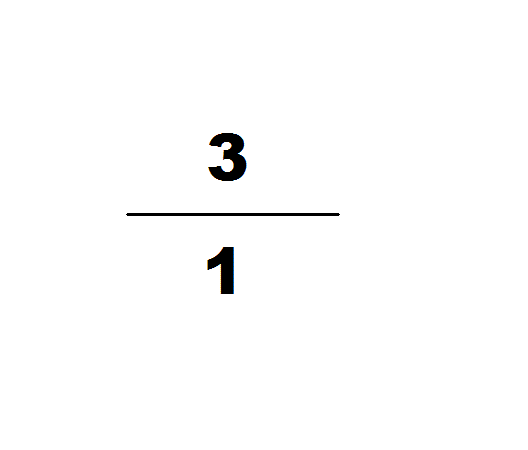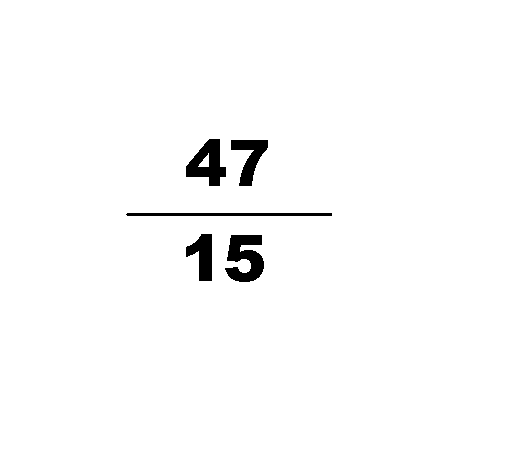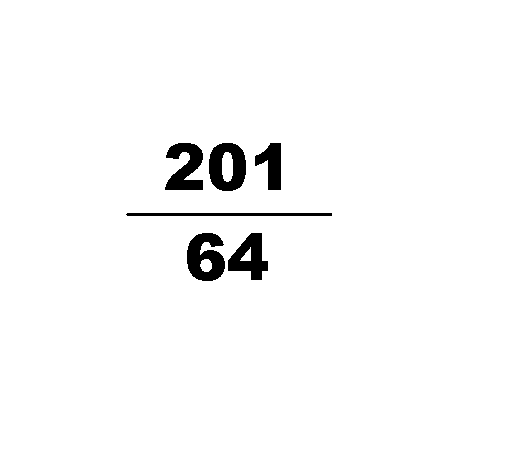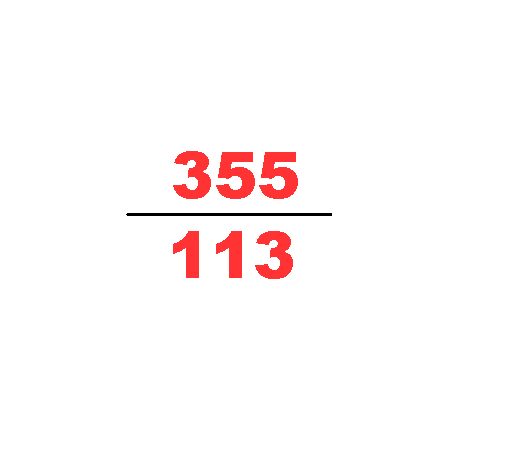
you won’t find a better one until 52163/16604! (Exclamation point, not factorial!) And at that, 52163/16604 is barely better; it differs from π by 0.0000002662, or 8.47 millionths of a percent.

That was the world’s best approximation for π for something like 900 years, until this guy came along. Pretty impressive!

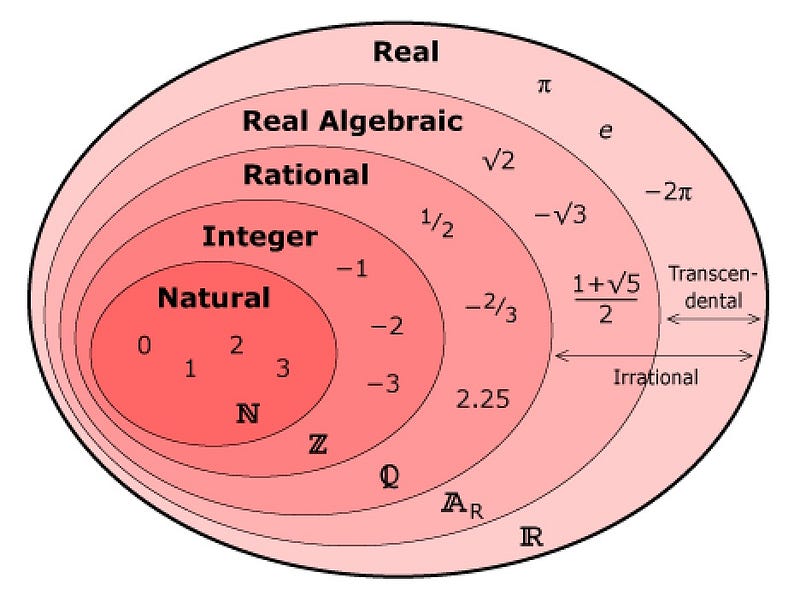
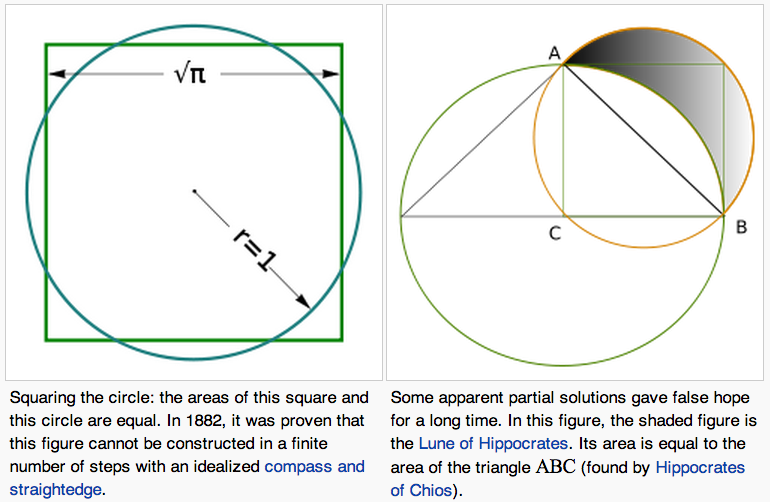
In fact, not only is π irrational, like √3, but you can’t even write down a polynomial equation that has π as a solution, which makes it not just irrational but also transcendental! (On the other hand, √3 is expressable as a solution to a polynomial equation, like “x^2 – 3 = 0.”) This means that one of the most famous math puzzles in history — to create a square with the same area as a circle using only a compass and straightedge — is fundamentally impossible!

But what if you wanted to calculate π, but wanted to do as little math as possible? No geometry, just basic counting and four-function mathematics? Well, if you can play darts, you can do it!

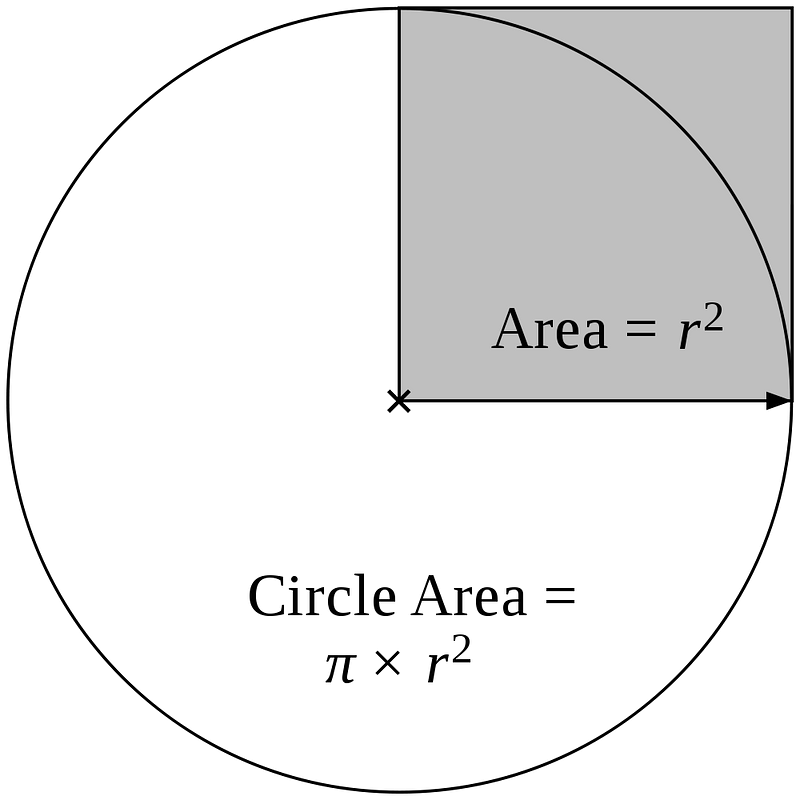
It will only get you to π very slowly, but throwing darts (randomly) at a circle with a square of area equal to the circle’s radius will allow you to calculate π! How so? Count the darts that land in the circle, divide by the number of darts that land in the grey square, and that’s how you calculate π. (For those of you who write a computer program that can do this, congratulations, you’ve just written your first monte carlo simulation!)

But let’s say you wanted to be more efficient, but you wanted to get to π with arbitrary accuracy, given enough time. Have I got a fun method for you: you can represent it as a continued fraction, and the farther you continue it, the more accurate you’ll get!

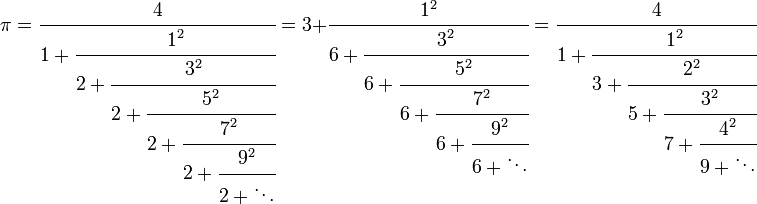
For example, here’s the results from the first few terms; not bad!

But there are some fun things about π that only appear if you’re willing to calculate very deeply. For example, here are the first 1,000 digits of π, and pay particular notice to the consecutive digits that appear.

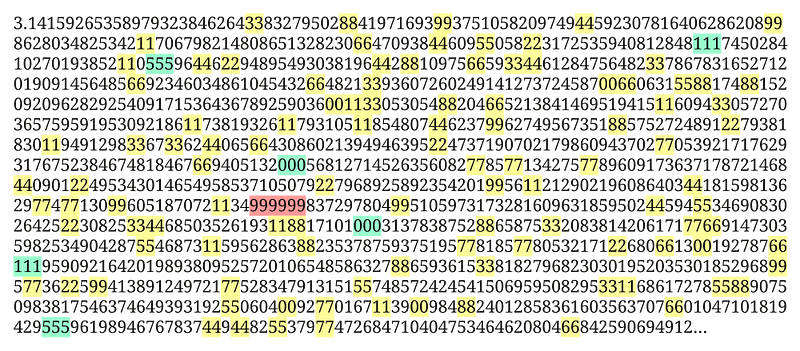
If you calculate it out, you’ll discover that 762 digits in, you arrive at a string of six 9s in a row, something incredibly unlikely to occur, and known as the Feynman Point. (Because Richard Feynman noted if he could memorize π to that point, he could say “nine-nine-nine-nine-nine-nine and so on…”)

In fact, you won’t find a string of seven digits in a row until you’ve written out nearly two million digits of π! But if you think that’s a fun fact, I’ve got a doozy for you. Try calculating this: the natural logarithm of the number 262,537,412,640,768,744, and dividing it by the square root of 163. What do you get?

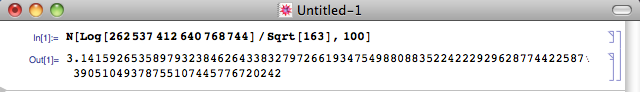
Unbelievably, you get almost π, but not quite! You see, it’s equal to π for the first 31 digits, but the 32nd digit is different! There’s a very intricate mathematical reason why this is so, but it’s also useful as a fun coincidence!

Pi Day is also a special day for anyone interested in astronomy and space! Four famous astronomy and space heroes have their birthday on Pi Day; can you name them all from their pictures? (Okay, the first one is easy.)

And finally, for the astronomy fans out there, there’s a famous star cluster in the night sky that looks an awful lot like π; take a look at Messier 38!

And with that said — whether you celebrate with math, confections, or a little of both — I hope you enjoy your π day!

(And — spoilers — your birthday boys are, from left-to-right, Albert Einstein, Apollo 8 Commander Frank Borman, Astronomer Giovanni Schiaparelli, and last-man-on-the-Moon Gene Cernan.)

A version of this post appeared at the old Starts With A Bang blog on Scienceblogs; check out our forum there now and leave a comment if you like!