To understand chaos theory, play a game of Plinko

- Chaos theory stems from the observations that given a complex enough system, its time-evolution will be unpredictable if you wait long enough, no matter how precisely you know the laws and initial conditions.
- Although it was never designed for the application, the simple game of Plinko, made famous by The Price Is Right, provides a perfect illustration of the idea of mathematical chaos.
- No matter how accurately you place two Plinko chips, one after the other, you simply cannot count on achieving the same outcome time after time.
Of all the pricing games on the iconic television show The Price Is Right, perhaps the most exciting of all is Plinko. Contestants play an initial pricing game to obtain up to 5 round, flat disks — known as Plinko chips — which they then press flat against a pegboard wherever they choose, releasing it whenever they like. One-at-a-time, the Plinko chips cascade down the board, bouncing off of the pegs and moving horizontally as well as vertically, until they emerge at the bottom of the board, landing in one of the prize (or no prize) slots.
Quite notably, contestants who drop a chip that happens to land in the maximum prize slot, always found in the direct center of the board, often try to repeat the exact same drop with whatever remaining disks they possess. Despite their best efforts, however, and the fact that the initial positioning of the disks might be virtually identical, the ultimate paths the disks wind up traversing are almost never identical. Surprisingly, this game is a perfect illustration of chaos theory and helps explain the second law of thermodynamics in understandable terms. Here’s the science behind it.

At a fundamental level, the Universe is quantum mechanical in nature, full of an inherent indeterminism and uncertainty. If you take a particle like an electron, you might think to ask questions like:
- Where is this electron?
- How fast and in what direction is this electron moving?
- And if I look away right now and look back one second later, where will the electron be?
They’re all reasonable questions, and we’d expect that they’d all have definitive answers.
But what actually transpires is so bizarre that it’s enormously unsettling, even to physicists who’ve spent their lifetimes studying it. If you make a measurement to precisely answer “Where is this electron?” you become more uncertain about its momentum: how fast and in what direction it moves. If you measure the momentum instead, you become more uncertain about its position. And because you need to know both momentum and position to predict where it will arrive with any certainty in the future, you can only predict a probability distribution for its future position. You’ll need a measurement at that future time to determine where it actually is.

Perhaps for Plinko, however, this quantum mechanical weirdness shouldn’t matter. Quantum physics might have a fundamental indeterminism and uncertainty inherent to it, but for large-scale, macroscopic systems, Newtonian physics ought to be perfectly sufficient. Unlike the quantum mechanical equations that govern reality at a fundamental level, Newtonian physics is completely deterministic.
According to Newton’s laws of motion — which can all be derived from F = ma (force equals mass times acceleration) — if you know the initial conditions, like position and momentum, you should be able to know exactly where your object is and what motion it will possess at any point in the future. The equation F = ma tells you what happens a moment later, and once that moment has elapsed, that same equation tells you what happens after the next moment has passed.
Any object for which quantum effects can be neglected obeys these rules, and Newtonian physics tells us how that object will continuously evolve over time.
However, even with perfectly deterministic equations, there’s a limit to how well we can predict a Newtonian system. If this surprises you, know that you’re not alone; most of the leading physicists who worked on Newtonian systems thought that there would be no such limit at all. In 1814, mathematician Pierre Laplace wrote a treatise entitled, “A philosophical essay on probabilities,” where he predicted that once we gained enough information to determine the state of the Universe at any moment in time, we could successfully use the laws of physics to predict the entire future of everything absolutely: with no uncertainty at all. In Laplace’s own words:
“An intellect which at a certain moment would know all forces that set nature in motion, and all positions of all items of which nature is composed, if this intellect were also vast enough to submit these data to analysis, it would embrace in a single formula the movements of the greatest bodies of the universe and those of the tiniest atom; for such an intellect nothing would be uncertain and the future just like the past would be present before its eyes.”
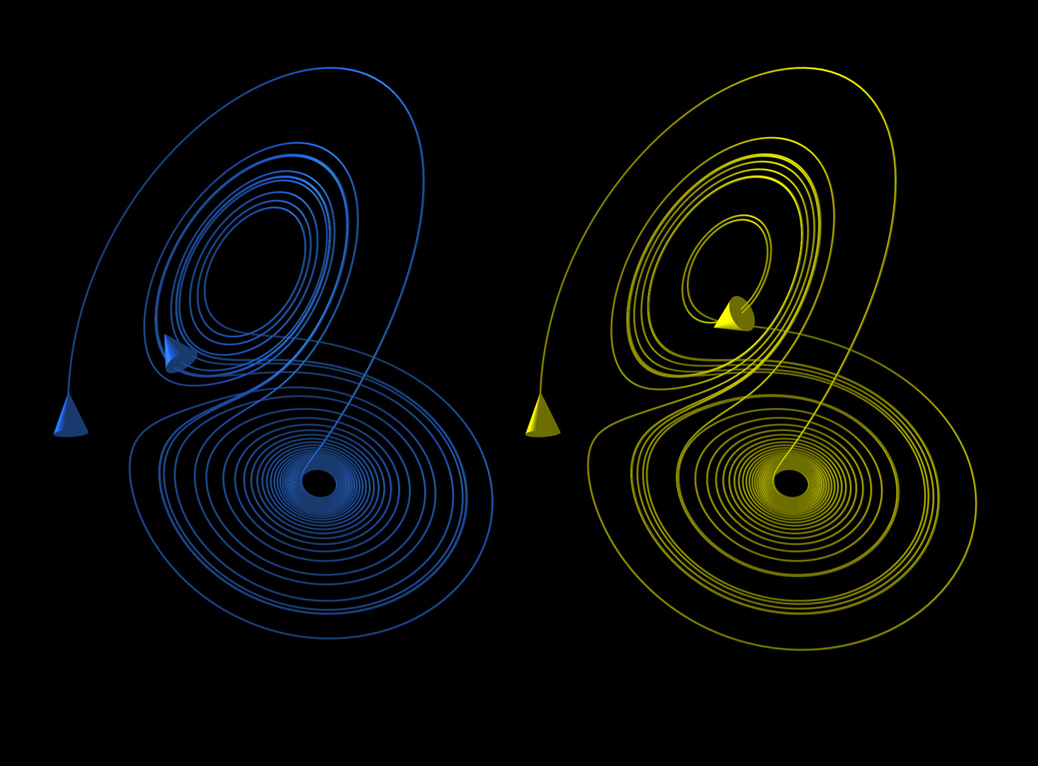
And yet, the need to invoke probabilities in making predictions about the future doesn’t necessarily stem from either ignorance (imperfect knowledge about the Universe) or from quantum phenomena (like Heisenberg’s uncertainty principle), but rather arises as a cause of the classical phenomenon: chaos. No matter how well you know the initial conditions of your system, deterministic equations — like Newton’s laws of motion — don’t always lead to a deterministic Universe.
This was first discovered back in the early 1960s, when Edward Lorenz, a meteorology professor at MIT, attempted to use a mainframe computer to help arrive at an accurate weather forecast. By using what he believed was a solid weather model, a complete set of measurable data (temperature, pressure, wind conditions, etc.), and an arbitrarily powerful computer, he attempted to predict weather conditions far into the future. He constructed a set of equations, programmed them into his computer, and waited for the results.
Then he re-entered the data, and ran the program for longer.

Surprisingly, the second time he ran the program, the results diverged at one point by a very slight amount, and then diverged thereafter very quickly. The two systems, beyond that point, behaved as though they were entirely unrelated to one another, with their conditions evolving chaotically with respect to one another.
Eventually, Lorenz found the culprit: when Lorenz re-entered the data the second time, he used the computer’s printout from the first run for the input parameters, which was rounded off after a finite number of decimal places. That tiny difference in initial conditions might have only corresponded to the width of an atom or less, but that was enough to dramatically alter the outcome, particularly if you time-evolved your system far enough into the future.
Small, imperceptible differences in the initial conditions led to dramatically different outcomes, a phenomenon colloquially known as the Butterfly Effect. Even in completely deterministic systems, chaos arises.

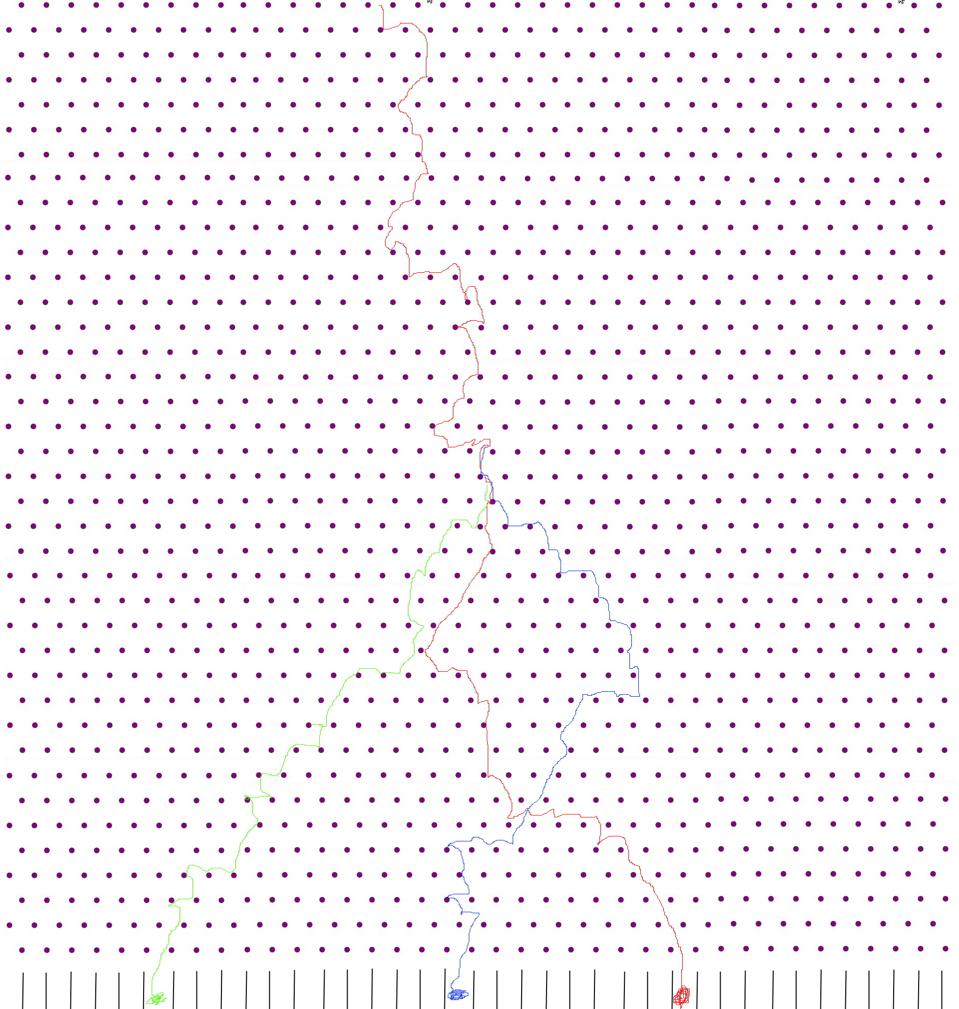
All of this brings us back to the Plinko board. Although there are many version of the game available, including at amusement parks and casinos, they’re all based on the idea of a Galton Board, where objects bounce one way or the other down an obstacle-filled ramp. The actual board used on The Price Is Right has somewhere around 13–14 different vertical levels of “pegs” for each Plinko chip to potentially bounce off of. If you’re aiming for the central spot, there are a lot of strategies you can employ, including:
- starting in the center and aiming for a drop that will keep the chip in the center,
- starting on a side and aiming for a drop that will bounce the chip towards the center by the time it reaches the bottom,
- or starting near the center, and aiming for a drop that will move farther away from the center before returning to the center.
Every time your chip hits a peg on the way down, it has the potential to knock you one-or-more spaces to either side, but every interaction is purely classical: governed by Newton’s deterministic laws. If you could stumble upon a path that caused your chip to land exactly where you desired, then in theory, if you could recreate the initial conditions precisely enough — down to the micron, the nanometer, or even the atom — perhaps, even with 13 or 14 bounces, you might wind up with an identical-enough outcome, winning the big prize as a result.
But if you were to expand your Plinko board, the effects of chaos would become unavoidable. If the board were longer and had dozens, hundreds, thousands, or even millions of rows, you’d quickly run into a situation where even two drops that were identical to within the Planck length — the fundamental quantum limit at which distances make sense in our Universe — you’d start to see the behavior of two dropped Plinko chips diverging after a certain point.
In addition, widening the Plinko board allows for a greater number of possible outcomes, causing the distribution of final states to be greatly spread out. Put simply, the longer and wider the Plinko board is, the greater the odds of not only unequal outcomes, but of having unequal outcomes that display an enormous-magnitude difference between two dropped Plinko chips.
This doesn’t just apply to Plinko, of course, but to any system with a large number of interactions: either discrete (like collisions) or continuous (such as from multiple gravitational forces acting simultaneously). If you take a system of air molecules where one side of a box is hot and the other side is cold, and you remove a divider between them, collisions between those molecules will spontaneously occur, causing the particles to exchange energy and momenta. Even in a small box, there would be more than 1020 particles; in short order, the entire box will have the same temperature, and will never separate into a “hot side” and a “cold side” again.
Even in space, just three point masses is enough to fundamentally introduce chaos. Three massive black holes, bound within distances the scale of the planets in our Solar System, will evolve chaotically no matter how precisely their initial conditions are replicated. The fact that there’s a cutoff in how small distances can get and still make sense — again, the Planck length — ensures that arbitrary accuracies on long-enough timescales can never be ensured.
The key takeaway of chaos is this: even when your equations are perfectly deterministic, you cannot know the initial conditions to arbitrary sensitivities. Even placing a Plinko chip on the board and releasing it with down-to-the-atom precision won’t be enough, with a large enough Plinko board, to guarantee that multiple chips would ever take identical paths. In fact, with a sufficiently large board, you can all but guarantee that no matter how many Plinko chips you dropped, you’d never arrive at two truly identical paths. Eventually, they’d all diverge.
Minuscule variations — the presence of air molecules moving from the host’s announcing, temperature variations arising from the contestant’s breath, vibrations from the studio audience propagating into the pegs, etc. — introduce enough uncertainty so that, far enough down the line, these systems are effectively impossible to predict. Along with quantum randomness, this effective classical randomness prevents us from knowing the outcome of a complex system, no matter how much initial information we possess. As physicist Paul Halpern so eloquently put it, “God plays dice in more ways than one.”