8 lessons on lifelong learning from an astrophysicist

Although most of us are fascinated with astrophysics — the science of what naturally forms all throughout the entirety of the cosmos — very few of us actually embark down the path of becoming an astrophysicist. To get there, one must not only master a large suite of mathematical tools, but also learn how to obtain solutions when faced with difficult problems. Sometimes, we run up against fundamental limitations:

• limits of what data or information can be obtained,
• limits to the applicability of our theories,
• or even limits to how well a problem can be solved, even in principle.

As physicists, we don’t get to control or dictate the laws of nature; we can only approximate reality as closely as possible in our attempts to describe nature.

Still, there is a particular set of skills that one must develop in order to be competent as an astrophysicist. While most fret about the difficulty of the math involved in solving such problems — from algebra to calculus to differential equations to even more advanced topics — it turns out that math skills can be practiced and gained just like any other skill: painting, swimming, or playing the piano. If you want to get good at painting, you must paint; if you want to get good at swimming, you must swim; and if you want to get good at solving math problems, you simply practice solving them. But along the way to becoming an astrophysicist, especially once you’ve acquired those necessary skills, a series of deeper insights emerges. Here are eight key lessons that apply near-universally to lifelong learners everywhere.

From 1845 to 1846, British astronomer John Couch Adams, working on the problem of Uranus’ orbit, proposed at least 5 and likely 6 potential locations for a hypothetical new planet, but detection remained elusive due to mistakes on both the theorist’s and the observers’ ends. Urbain Le Verrier, meanwhile, made his first and only prediction in 1846, which led to a near-immediate observational discovery when his letter arrived at the Berlin Observatory.

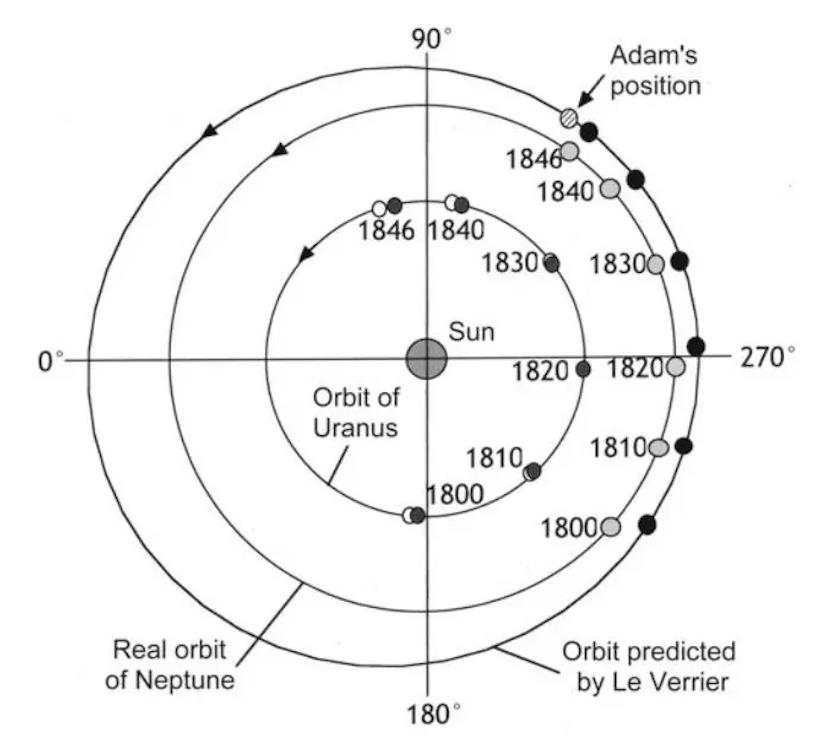
1.) The most critical step in problem-solving is setting up the problem correctly. One of the first things you learn, when faced with a problem in physics or astronomy, is how to systematically approach it. You begin by identifying what is known and what you need to know, and then, based on the relationships that exist between those various quantities, you attempt to devise a step-by-step plan to arrive at an answer or solution to what needs to be known. While most people — particularly those who are intimidated by physics or mathematics — fret about the execution of that step-by-step plan, including the more mathematically intensive aspects of those steps, the truth is that those are skill-based steps. If you make an error, it’s not the end of the world; plenty of competent colleagues can help catch and remedy it.

However, if you’ve set up the problem incorrectly, your entire approach will be wrong. No matter how perfectly you execute your steps from that point onward, you’ll only arrive at solutions to a different (and most likely irrelevant) problem. This means that identifying:

• what you know,
• what you need to know,
• what the key relationships between those quantities are,
• and determining how to figure out a solution (including by taking measurements or collecting data to fill in any important “missing pieces” along the way),

are the most critical aspects of arriving at a potential solution. Sure, you (or someone) actually does have to solve the problem at some point, but figuring out what problem you actually need to solve, as well as how you’ll solve it, are essential steps that no level of mathematical adeptness on its own can replace.

This animation shows the flight trajectory of the Europa Clipper mission, including its flyby of Mars in early 2025, its flyby of Earth in late 2026, and its encounter and eventual gravitational capture by the Jovian system in early 2030. Despite the radiation of Jupiter, the Europa Clipper’s highly elliptical orbit will enable several dozen close encounters with Europa before the spacecraft becomes non-operational.

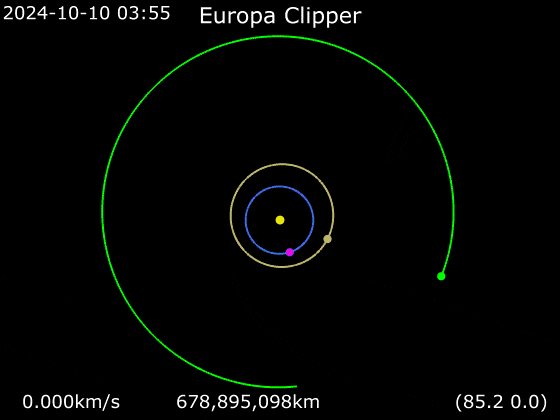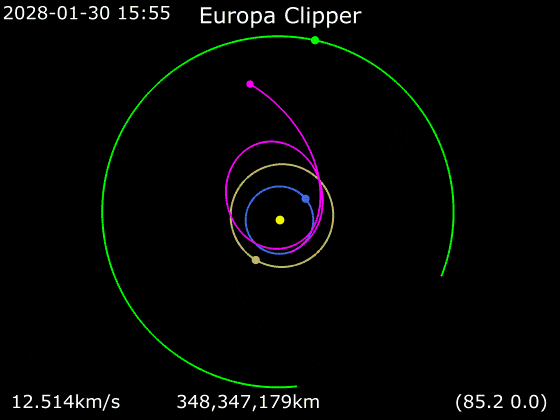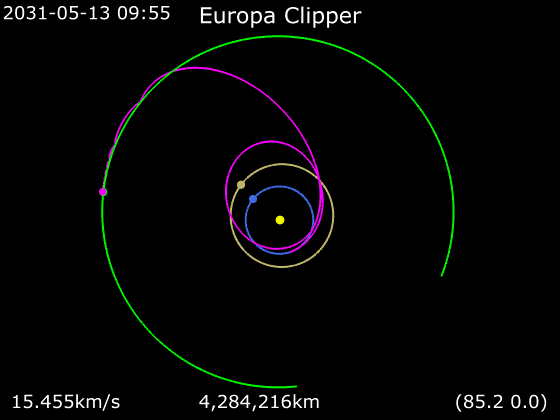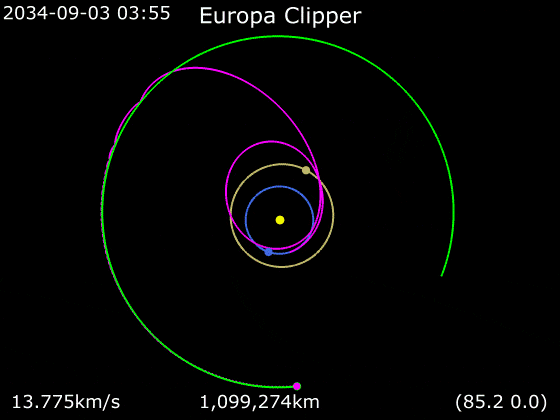
2.) A sufficiently close approximation is often just as good as an exact result. As humanity explores the Solar System, one of our most important tools is the ability to send spacecraft to whatever world or body we’re interested in: to investigate what we’re studying up close, or in situ. We’ve been sending spacecraft to the Moon, Venus, and Mars since the 1960s. Since the dawn of the 21st century, we’ve flown past Pluto, come closer to the Sun than ever before, and have missions on their way to explore the icy moons of Jupiter. Our best theory of gravity has been around since 1915: Einstein’s general relativity, since well before any human-created object broke free of the bonds of Earth’s gravity.

And yet, when we perform the theoretical calculations needed to tell these spacecraft where they need to be at what time so that they’ll wind up on the proper trajectories toward their ultimate destination, we don’t use general relativity at all. We simply use the equations of Newtonian gravity, despite the fact that we know it’s only a coarse approximation of Einstein’s more general, more rigorous formulation of gravity. The reasons why are simple and straightforward:

• it’s easier to solve problems using Newton’s equations than Einstein’s equations,
• and that Newton’s equations yield answers that are good enough approximations of reality that our missions are no less successful because we used them instead of the more difficult Einstein equations.

Knowing when you have to devote the resources to solving a much harder version of a problem, as well as when you can save yourself those efforts, is a key lesson that applies to much more than astrophysics.

The Variable Star RS Puppis, with its light echoes shining through the interstellar clouds. Variable stars come in many varieties; one of them, Cepheid variables, can be measured both within our own galaxy and in galaxies up to 50–60 million light years away, with all Cepheid variables exhibiting a relationship between their period, or how long it takes them to reach maximum brightness again after fading, and their luminosity, or how intrinsically bright they are.

3.) Keep an eye out for the underlying relationships between things you can observe or measure. Around the dawn of the 20th century, astronomy was a very male-dominated field, and women who were interested in the endeavor were relegated to data processing roles: such as sorting stars into various categories based on their properties. One of those women was Henrietta Leavitt, who noticed that a certain class of prominently bright, blue-colored stars (Cepheids) would fade and brighten periodically over time. The brighter the star was, the faster the variation; the fainter the star was, the slower the variation. This relationship became known as the period-luminosity relationship, or as it’s sometimes called in more modern times, Leavitt’s Law.

Even though it isn’t clear, even today, why this underlying relationship exists, it’s played an important role in determining the distance to galaxies other than our own. Edwin Hubble used this relationship to measure the distance to Andromeda and other nearby galaxies, discovering the expanding Universe as a result. Today, Cepheids are used as a reliable distance indicator: an invaluable tool in helping construct the cosmic distance ladder. Knowing how various measurable, observable quantities are related can be a vital piece of information in problem-solving, even if you don’t understand everything about the nature of the relationship itself.

A mural of the Einstein field equations, with an illustration of light bending around the eclipsed Sun: the key observations that first validated general relativity four years after it was first theoretically put forth: back in 1919. The Einstein tensor is shown decomposed, at left, into the Ricci tensor and Ricci scalar, with the cosmological constant term added in after that. If that constant weren’t included, an expanding (or collapsing) Universe would have been an inevitable consequence.

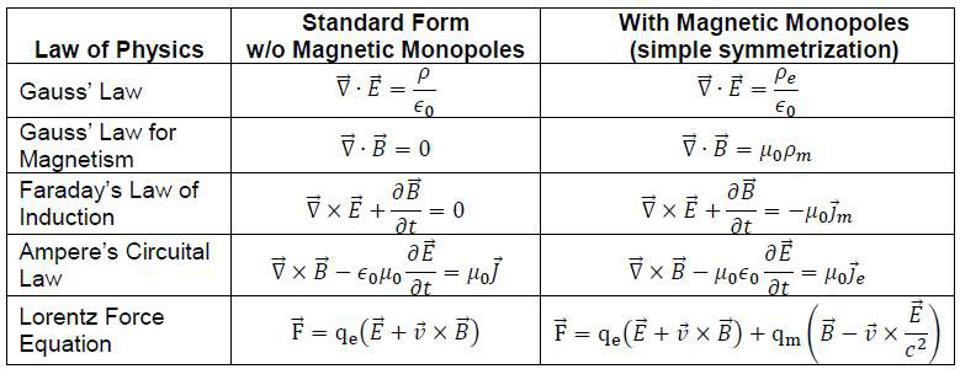
4.) Always be aware that your expertise in a subject, no matter how deep it runs, is limited. This is one of the most humbling lessons that comes along with any scientific endeavor: after years or even decades of study, even after becoming a world expert in your specific field, you must always recognize that there are going to be others that know more about aspects of your field than you do. There’s always someone who has specialized in aspects of what you do or what you study that has greater expertise than you do, whom you can learn from and gain knowledge from if you’re willing to listen to what they have to say.

This level of humility is not easy to glean; we are all too often victims of our own overconfidence in ourselves and what we know. Recognizing where your expertise ends and knowing when to seek additional information in order to better understand and solve a problem you’re faced with has been a hallmark of scientific advances throughout history, from Halley asking Newton about the orbits of periodic comets to Einstein asking his friend Marcel Grossman for help with the mathematics of curved spacetime. If Einstein was able to recognize the limits of his own expertise and was capable of seeking help when he needed additional skills that he himself lacked, perhaps we, too, can be humble enough to admit when we require additional information to properly address the puzzles we’re facing.

It’s possible to write down a variety of equations, like Maxwell’s equations, that describe the Universe. We can write them down in a variety of ways, but only by comparing their predictions with physical observations can we draw any conclusion about their validity. It’s why the version of Maxwell’s equations with magnetic monopoles (right) doesn’t correspond to reality, while the ones without (left) do. The displacement current term (the second term in the fourth equation) arose from Maxwell’s attempt to fix the incompleteness in electricity and magnetism that existed prior to his work: an important theoretical leap that turned out to accurately reflect reality.

5.) Knowing where to find the information you need is more important than memorizing it. There are certain pieces of information we keep in our heads all the time, because we use that information frequently and need to recall it at a moment’s notice. Other pieces of information, however, are either difficult to remember, obscure, or are only needed occasionally. Astrophysicists might remember the speed of light in a vacuum regularly, but rarely recall details such as the speed of light through an acrylic medium or the speed of sound at an altitude of 16 kilometers (10 miles) above sea level. This isn’t something that one should remedy through the memorization of an ever-increasing suite of facts, values, and equations but rather simply by knowing where to go to access that relevant information whenever it’s needed.

While physics students may often be chided for using the wrong formula or inserting an incorrect value into an equation, the solution isn’t to memorize everything you’ll ever need and store it in your head; very few of us have brains that are capable of that type of perfect recall every time. Instead, knowing where that relevant information can be found, and being able to access it when the necessary circumstances arise, is a far more useful set of skills for nearly all applications. Most problems that most humans face are not game show-like in nature, requiring an instant, perfect answer to a relatively trivial matter. Knowing how to find and access whatever information might be relevant to a class of problems you’re likely to encounter, however, is a skill that should be cultivated by almost everyone.

This diagram should be read from the upper right, following the arrows, to show the decay chain (and the mean lifetime of each step) of the unstable element uranium-238. Although the longest step is the first one, the end product, of lead-206, is only reached several hundreds of thousands of years after the first step in the decay chain takes place. Nevertheless, over 99.9% of the U-238 that has decayed since Earth first formed is now in the form of lead-206.

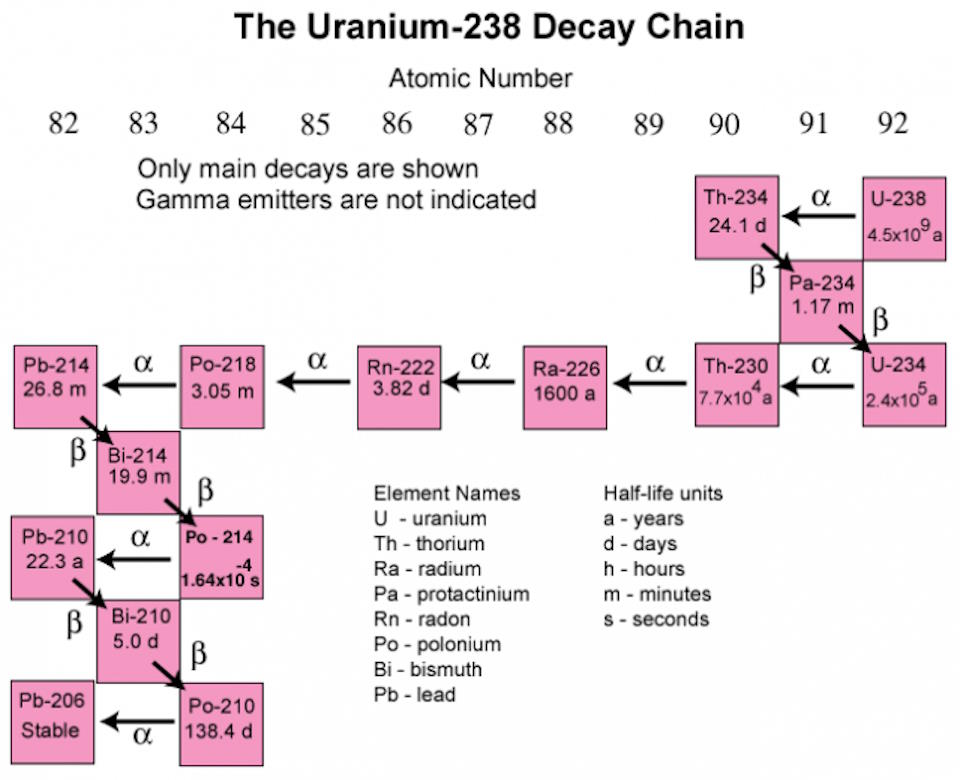
6.) Knowing which aspects of a puzzle are extraneous can keep you from succumbing to distractions. If you begin with a sample of uranium, one of the heaviest naturally occurring elements in the Universe, it won’t remain as uranium forever. The most common isotope of uranium has 92 protons and 146 neutrons, and is known as uranium-238. With a half-life of 4.5 billion years, roughly 50% of the uranium-238 that was formed at the same time as planet Earth (4.5 billion years ago) is still present on our world today.

What form does the other 50% possess today?

This might seem like an extremely difficult problem, as uranium-238 doesn’t just decay to thorium-234 after 4.5 billion years. Thorium-234 is also unstable, and will decay to protactinium-234 with its own half-life. Protactinium-234 is similarly unstable, as is its decay product: uranium-234. Uranium-234 decays to thorium-230, which decays to radon-226, and in fact there are a total of fourteen decay steps that take place before one arrives at a truly stable end result: lead-206. Some of those decay steps take only microseconds, while others take minutes, days, years, or even millennia.

And yet, if you answer “the other 50% is lead-206,” you’ll be more than 99.9% correct; less than 0.1% of the initial uranium-238 exists in the form of all other 13 possible “steps” in the decay chain combined. Rather than a 14-step calculation, simply knowing that, for this particular problem, only one step is important and relevant can save you an enormous amount of time and effort. This has widespread applications to a variety of problems we face: not only about radioactive decay.

If the Earth were perfectly flat and the acceleration, everywhere, were straight down, all projectiles would make a parabola. But for real projectiles (exaggerated, at right), the acceleration is always toward Earth’s center, which means the trajectory must be a portion of an ellipse, rather than a parabola.

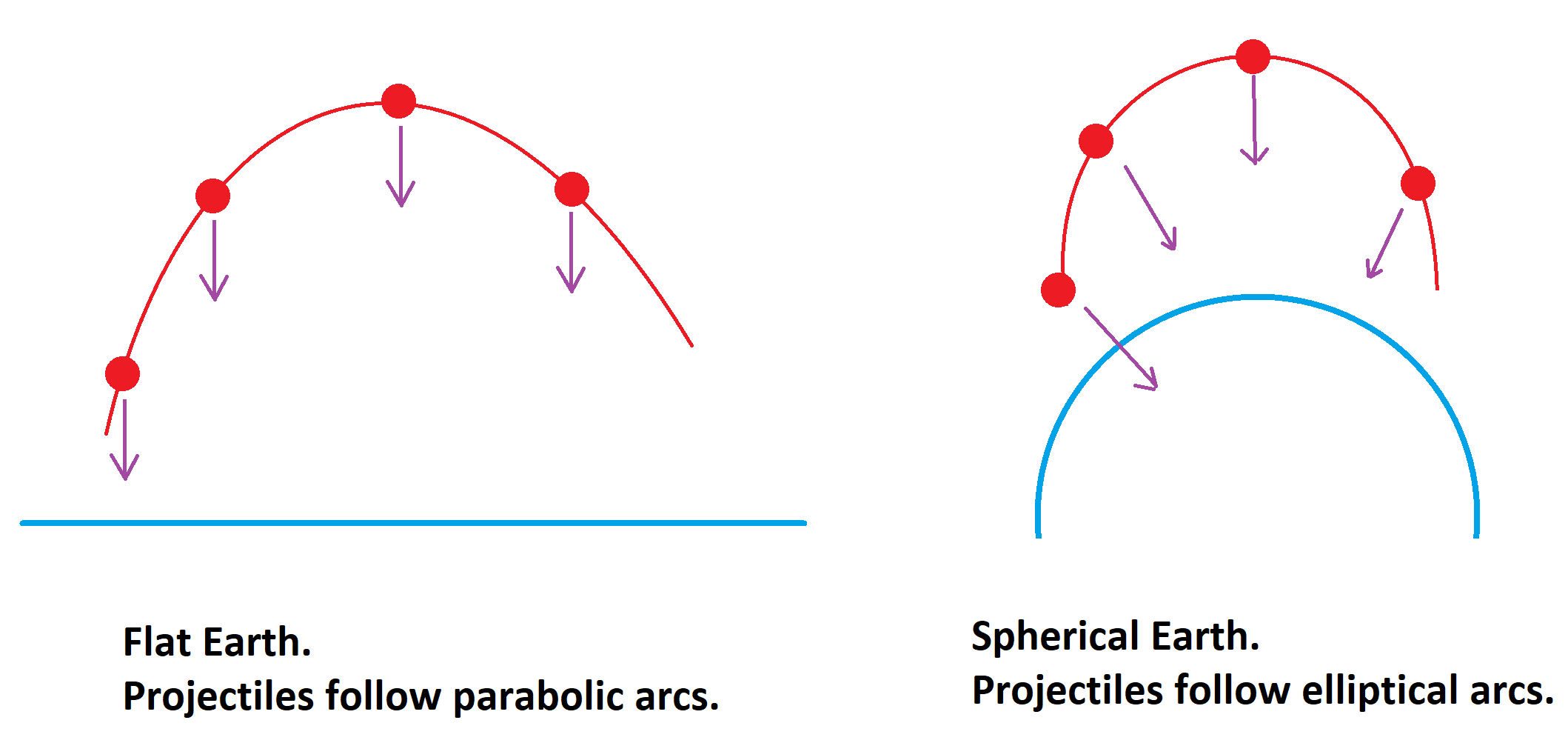
7.) There’s almost always a deeper layer to reality than our current understanding indicates. When most of us begin studying physics, we start with mechanics: projectiles in free-fall and balls rolling down ramps. We solve problems using simple algebra, which is totally sufficient for those applications. Later on, we learn that position, velocity, and acceleration aren’t just related to one another, but are aspects of a differential equation: where the same formula describes how an object’s motion changes from one instant to the next. Even more deeply, the quantum nature of reality teaches us that quantities such as “position” can never even be measured exactly, but that there’s a fundamental amount of uncertainty that’s always inherent to it.

This is the flipside to the previous lesson. While ignoring extraneous pieces of information that aren’t relevant to the problem in question can save you time and effort, uncovering deeper relationships between various aspects of whatever system you’re studying can lead to the ability to predict phenomena that would otherwise be surprising and mysterious. Oftentimes, the order is reversed, and it’s the appearance of surprising and mysterious phenomena that leads us forward to search for a deeper, hitherto undiscovered set of relationships between quantities we’re familiar with. One must always remember that our perception of reality is not reality itself, but only our best approximation of it.

There is a positive, non-zero energy inherent to quantum fields themselves: including the radiation field from electrodynamics, the chromodynamic field from the strong nuclear force, and the weak field from the weak nuclear force. Although these manifest, in our calculations, as virtual particles which appear in Feynman diagrams, they have physically real effects, such as the Lamb shift and the Schwinger effect. The total energy inherent to space can never be zero, or pathological consequences arise.

8.) When things don’t add up, be prepared to re-examine your underlying assumptions. This happens more often than you might think: we have a theory for how something ought to work, we perform the key experiments or make the key measurements, and what we find differs from what we thought we’d find. The big question is always, “Why?” Why did our predictions fail to match what occurred? Was there a flaw with our experiments or measurements? Did we make a calculational mistake when it comes to our predictions? Although sometimes the fault lies with something we’ve done, very often there’s a flaw in the underlying assumptions that we made when either performing our experiments or making our predictions.

Perhaps we’ve ignored something that is relevant to the problem we’re asking. Perhaps we extrapolated the expected behavior of a system to outside its range of demonstrated validity. Perhaps our model that we’re using is oversimplified, and a more complex one is required. Or, perhaps the laws that we expected to govern the system need to be modified for this particular system, and by extension, for others as well. The most important aspect of science is this: the world can be understood, but our understanding of it at any moment in time is only provisional and is always subject to revision. If our data and our predictions fail to match, it’s often the assumptions that underlie our thinking that are flawed, and questioning them is part of how we find solutions to otherwise intractable problems.

This portion of the CEERS survey contains a wide variety of galaxies found at all distances, from nearby to more than 30 billion light-years away, as imaged by JWST’s NIRCam instrument. Some galaxies appear to have grown so massive, so quickly, that simulations couldn’t account for them. However, a new study finds that some of those early galaxies are in fact much less massive than they first appeared, and are brightness-enhanced by both bursty star-formation and from the light produced from active supermassive black holes within them.

There’s a piece of advice I got from a mathematics professor back when I was in my first year of college. At the beginning of a course on differential equations, he cautioned us, “Most of the differential equations that exist cannot be solved. And most of the differential equations that can be solved cannot be solved by you.” This underscored two vital aspects to learning.

• Not every problem you’ll face is a problem you can solve exactly, but rather is something you can only make a best attempt at for your particular needs.
• However, if you do need a solution, you had better be prepared not merely to make the best attempt you can muster, but also to be resourceful in accessing all previous work that has been done on the topic; the answer you seek may be beyond your capabilities, but may not be beyond the capabilities of everyone who’s ever faced it.

When most of us think of learning, we think about knowledge that we gain through experience, study, or practice. But learning itself is so much more: it’s an art, it’s a skill, and it can only take place if you have the ability to recognize when what you already know is insufficient to tell the full, comprehensive story of the puzzle you’re faced with. These eight lessons, even if you yourself aren’t an astrophysicist at all, can still help everyone become a better learner.