What Is The Smallest Possible Distance In The Universe?

The Planck length is a lot smaller than anything we’ve ever accessed. But is it a true limit?

If you wanted to understand how our Universe operates, you’d have to examine it at a fundamental level. Macroscopic objects are made up of particles, which can only themselves be detected by going to subatomic scales. To examine the Universe’s properties, you must to look at the smallest constituents on the smallest possible scales. Only by understanding how they behave at this fundamental level can we hope to understand how they join together to create the human-scale Universe we’re familiar with.

But you can’t extrapolate what we know about even the small-scale Universe to arbitrarily small distance scales. If we decide to go down to below about 10^-35 meters ⁠ — the Planck distance scale ⁠ — our conventional laws of physics only give nonsense for answers. Here’s the story of why, below a certain length scale, we cannot say anything physically meaningful.

Imagine, if you like, one of the classic problems of quantum physics: the particle-in-a-box. Imagine any particle you like, and imagine that it’s somehow confined to a certain small volume of space. Now, in this quantum game of peek-a-boo, we’re going to ask the most straightforward question you can imagine: “where is this particle?”

You can make a measurement to determine the particle’s position, and that measurement will give you an answer. But there will be an inherent uncertainty associated with that measurement, where the uncertainty is caused by the quantum effects of nature.

How large is that uncertainty? It’s related to both ħ and L, where ħ is Planck’s constant and L is the size of the box.

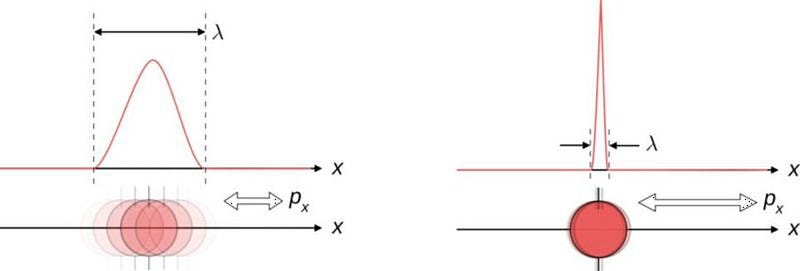
For most of the experiments we perform, Planck’s constant is small compared to any actual distance scale we’re capable of probing, and so when we examine the uncertainty we get — related to both ħ and L — we’ll see a small inherent uncertainty.

But what if L is small? What if L is so small that, relative to ħ, it’s either comparably sized or even smaller?

This is where you can see the problem start to arise. These quantum corrections that occur in nature don’t simply arise because there’s the main, classical effect, and then there are quantum corrections of order ~ħ that arise. There are corrections of all orders: ~ħ, ~ħ², ~ħ³, and so on. There’s a certain length scale, known as the Planck length, where if you reach it, the higher-order terms (which we usually ignore) become just as important as, or even more important than, the quantum corrections we normally apply.

What is that critical length scale, then? The Planck scale was first put forth by physicist Max Planck more than 100 years ago. Planck took the three constants of nature:

1. G, the gravitational constant of Newton’s and Einstein’s theories of gravity,
2. ħ, Planck’s constant, or the fundamental quantum constant of nature, and
3. c, the speed of light in a vacuum,

and realized that you could combine them in different ways to get a single value for mass, another value for time, and another value for distance. These three quantities are known as the Planck mass (which comes out to about 22 micrograms), the Planck time (around 10^-43 seconds), and the Planck length (about 10^-35 meters). If you put a particle in a box that’s the Planck length or smaller, the uncertainty in its position becomes greater than the size of the box.

But there’s a lot more to the story than that. Imagine you had a particle of a certain mass. If you compressed that mass down into a small enough volume, you’d get a black hole, just like you would for any mass. If you took the Planck mass — which is defined by the combination of those three constants in the form of √(ħc/G) — and asked that question, what sort of answer would you get?

You’d find that the volume of space you needed that mass to occupy would be a sphere whose Schwarzschild radius is double the Planck length. If you asked how long it would take to cross from one end of the black hole to the other, the length of time is four times the Planck time. It’s no coincidence that these quantities are related; that’s unsurprising. But what might be surprising is what it implies when you start asking questions about the Universe at those tiny distance and time scales.

In order to measure anything at the Planck scale, you’d need a particle with sufficiently high energy to probe it. The energy of a particle corresponds to a wavelength (either a photon wavelength for light or a de Broglie wavelength for matter), and to get down to Planck lengths, you need a particle at the Planck energy: ~10¹⁹ GeV, or approximately a quadrillion times greater than the maximum LHC energy.

If you had a particle that actually achieved that energy, its momentum would be so large that the energy-momentum uncertainty would render that particle indistinguishable from a black hole. This is truly the scale at which our laws of physics break down.

When you examine the situation in greater detail, it only gets worse. If you start thinking about quantum fluctuations inherent to space (or spacetime) itself, you’ll recall there’s also an energy-time uncertainty relation. The smaller the distance scale, the smaller the corresponding timescale, which implies a larger energy uncertainty.

At the Planck distance scale, this implies the appearance of black holes and quantum-scale wormholes, which we cannot investigate. If you performed higher-energy collisions, you’d simply create larger mass (and larger size) black holes, which would then evaporate via Hawking radiation.

You might argue that, perhaps, this is why we need quantum gravity. That when you take the quantum rules we know and apply them to the law of gravity we know, this is simply highlighting a fundamental incompatibility between quantum physics and General Relativity. But it’s not so simple.

Energy is energy, and we know it causes space to curve. If you start attempting to perform quantum field theory calculations at or near the Planck scale, you no longer know what type of spacetime to perform your calculations in. Even in quantum electrodynamics or quantum chromodynamics, we can treat the background spacetime where these particles exist to be flat. Even around a black hole, we can use a known spatial geometry. But at these ultra-intense energy, the curvature of space is unknown. We cannot calculate anything meaningful.

At energies that are sufficiently high, or (equivalently) at sufficiently small distances or short times, our current laws of physics break down. The background curvature of space that we use to perform quantum calculations is unreliable, and the uncertainty relation ensures that our uncertainty is larger in magnitude than any prediction we can make. The physics that we know can no longer be applied, and that’s what we mean when we say that “the laws of physics break down.”

But there might be a way out of this conundrum. There’s an idea that’s been floating around for a long time — since Heisenberg, actually — that could provide a solution: perhaps there’s a fundamentally minimal length scale to space itself.

Of course, a finite, minimum length scale would create its own set of problems. In Einstein’s theory of relativity, you can put down an imaginary ruler, anywhere, and it will appear to shorten based on the speed at which you move relative to it. If space were discrete and had a minimum length scale, different observers — i.e., people moving at different velocities — would now measure a different fundamental length scale from one another!

That strongly suggests there would be a “privileged” frame of reference, where one particular velocity through space would have the maximum possible length, while all others would be shorter. This implies that something that we currently think is fundamental, like Lorentz invariance or locality, must be wrong. Similarly, discretized time poses big problems for General Relativity.

Still, there may actually be a way to test whether there is a smallest length scale or not. Three years before he died, physicist Jacob Bekenstein put forth a brilliant idea for an experiment. If you pass a single photon through a crystal, you’ll cause it to move by a slight amount.

Because photons can be tuned in energy (continuously) and crystals can be very massive compared to a photon’s momentum, we could detect whether the crystal moves in discrete “steps” or continuously. With low-enough energy photons, if space is quantized, the crystal would either move a single quantum step or not at all.

At present, there is no way to predict what’s going to happen on distance scales that are smaller than about 10^-35 meters, nor on timescales that are smaller than about 10^-43 seconds. These values are set by the fundamental constants that govern our Universe. In the context of General Relativity and quantum physics, we can go no farther than these limits without getting nonsense out of our equations in return for our troubles.

It may yet be the case that a quantum theory of gravity will reveal properties of our Universe beyond these limits, or that some fundamental paradigm shifts concerning the nature of space and time could show us a new path forward. If we base our calculations on what we know today, however, there’s no way to go below the Planck scale in terms of distance or time. There may be a revolution coming on this front, but the signposts have yet to show us where it will occur.