How many fundamental constants does it take to define our Universe?

And even with them all in place, what do we still not know?
“The joy of life consists in the exercise of one’s energies, continual growth, constant change, the enjoyment of every new experience. To stop means simply to die. The eternal mistake of mankind is to set up an attainable ideal.” –Aleister Crowley
When we think about our Universe at a fundamental level, we think about all the particles in it and all the forces and interactions that occur between them. If you can describe those forces, interactions and particle properties, you have everything you need to reproduce our Universe, or at least a Universe virtually indistinguishable from our own, in its entirety. Because if you know the laws of physics — gravitation, quantum mechanics, electromagnetism, the nuclear forces, etc. — all you need are the relationships that tell you “by how much,” and so long as you start with the same initial conditions, you’ll wind up with a Universe with the same structures from atoms to galaxy clusters, the same processes from electron transitions to stellar explosions, the same periodic table of elements, and the same chemical combinations from hydrogen gas to proteins and hydrocarbon chains, among a great number of other similarities.

When you encounter the question of “how much,” you probably think of the force of gravity being determined by a universal gravitational constant, G, and of the “energy of a particle” being determined by its rest mass, such as the mass of an electron, me. You think of the speed of light, c, and for quantum mechanics, Planck’s constant, ħ. But physicists don’t like to use these constants when we describe the Universe, because these constants have arbitrary dimensions and units to them.
But there’s no inherent importance to a unit like a meter, a kilogram or a second; in fact there’s no reason at all to force ourselves to define things like “mass” or “time” or “distance” when it comes to the Universe. If we give the right dimensionless constants (without meters, kilograms, seconds or any other “dimensions” in them) that describe the Universe, we should naturally get out our Universe itself. This includes things like the masses of the particles, the strengths of their interactions, the speed limit of the Universe and even the fundamental properties of spacetime itself!
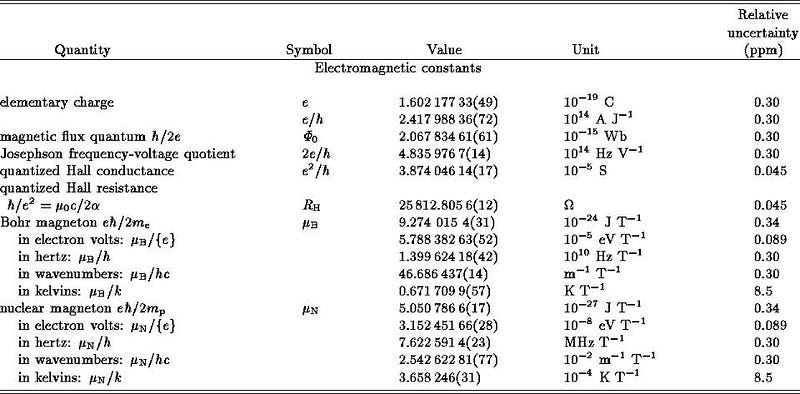
As it turns out, it takes 26 dimensionless constants to describe the Universe as simply and completely as possible, which is quite a small number. Even at that, they don’t give us everything, because there are some important things that are fundamentally still unknown about our Universe. Here’s what the constants we need are.
1.) The fine-structure constant, or the strength of the electromagnetic interaction. In terms of some of the physical constants we’re more familiar with, this is a ratio of the elementary charge (of, say, an electron) squared to Planck’s constant and the speed of light. But if you put these constants together, you get a dimensionless number! At the energies currently present in our Universe, this number comes out to ≈ 1/137.036, although the strength of this interaction increases as the energy of the interacting particles rise.
2.) The strong coupling constant, which defines the strength of the force that holds protons and neutrons together. Although the way the strong force works is very different from the electromagnetic force or gravity, the strength of this interaction can still be parametrized by a single coupling constant. This constant of our Universe, too, like the electromagnetic one, changes strength with energy.
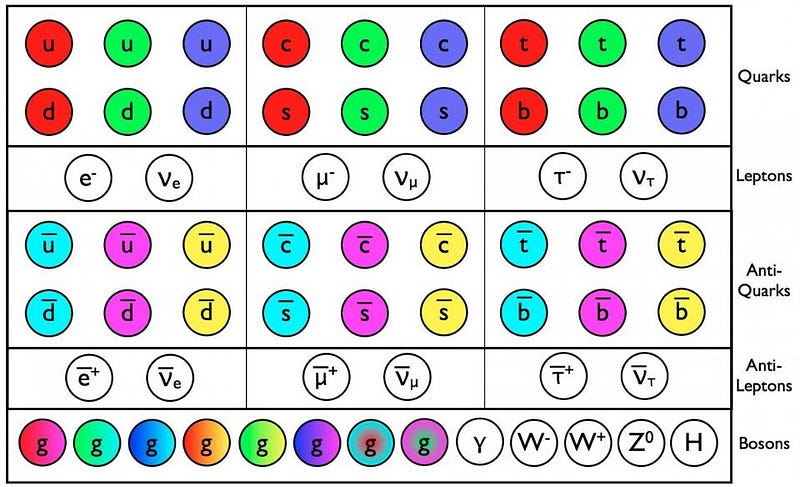
3–17.) This one is a bit of a disappointment. We have fifteen particles in the Standard Model: the six quarks, six leptons, the W, Z, and the Higgs boson, that all have a rest mass. While it’s true that their antiparticles all have identical rest masses, we were hoping that there would’ve been some relationship, pattern, or more fundamental theory that gave rise to these masses with fewer parameters than the fifteen we need: one for each non-zero rest mass. Alas, it takes fifteen constants to describe these masses, with the lone good news that we can scale these parameters to be relative to the gravitational constant, G, to wind up with 15 dimensionless parameters that have no need for a separate descriptor of the gravitational force’s strength.
18–21.) The quark mixing parameters. We have six different types of quarks, and because there are two subsets of three that all have the same quantum numbers as one another, they can mix together. If you’ve ever heard of the weak nuclear force, radioactive decay or CP-violation, these four parameters — all of which must be (and have been) measured — are required to describe them.
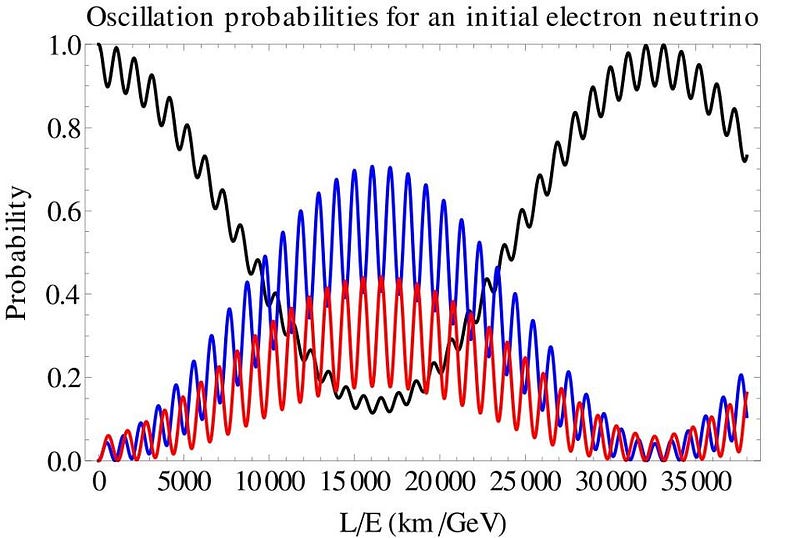
22–25.) The neutrino mixing parameters. Similar to the quark sector, there are four parameters that detail how neutrinos mix with one another, given that the three types of neutrino species all have the same quantum number. The solar neutrino problem — where the neutrinos emitted by the Sun weren’t arriving here on Earth — was one of the 20th century’s biggest conundrums, finally solved when we realized that neutrinos had very small but non-zero masses, mixed together, and oscillated from one type into another. The quark mixing is described by three angles and one CP-violating complex phase, and the neutrino mixing is described in the same way. While all four parameters have already been determined for the quarks, the CP-violating phase for the neutrinos is not yet measured.
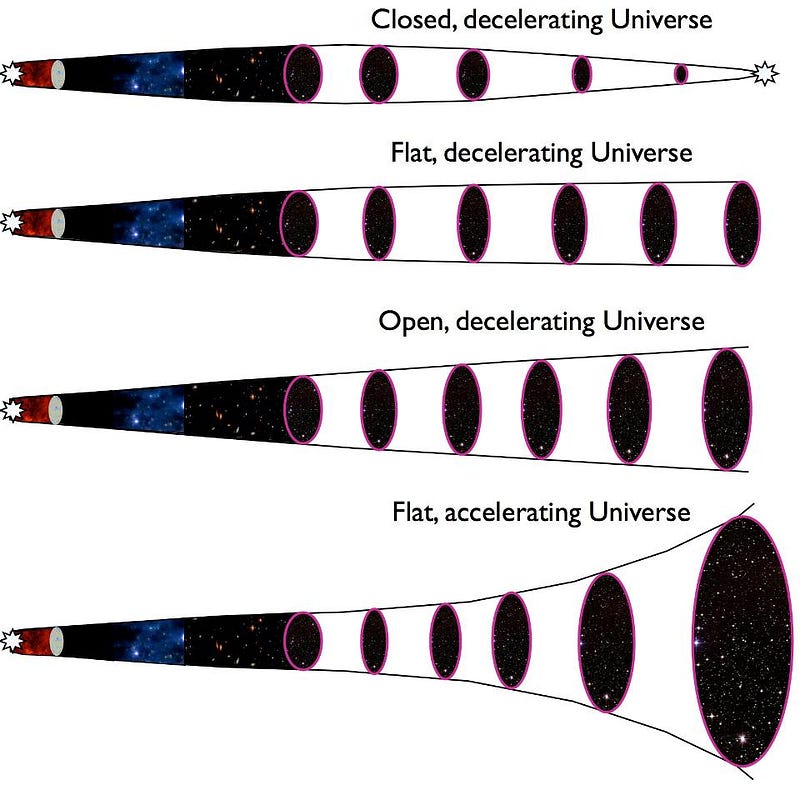
26.) The cosmological constant. You may have heard that the Universe’s expansion is accelerating due to dark energy, and this requires yet one more parameter — a cosmological constant — to describe the amount of that acceleration. Dark energy could yet turn out to be more complex than being a constant, in which case it may need more parameters as well, and hence the number may be greater than 26.
If you give me the laws of physics and these 26 constants, I can throw these into a computer and tell it to simulate my Universe. And quite remarkably, what I get out looks pretty much indistinguishable from the Universe we have today, from the smallest, subatomic scales all the way up to the largest, cosmic ones.
But even with this, there are still four puzzles that will likely require at least some additional constants to resolve. These are:
- The problem of the matter-antimatter asymmetry. The entirety of our observable Universe is made up dominantly of matter and not antimatter, yet we do not fully understand why this is so, or why our Universe has the amount of matter it does. This problem — the problem of baryogenesis — is one of the great unsolved problems in theoretical physics, and may require one (or more) new fundamental constants to describe its solution.
- The problem of cosmic inflation. This is the phase of the Universe that preceded and set up the Big Bang has made many new predictions that have been verified observationally, but isn’t included in this description. Very likely, when we more fully understand what this is, additional parameters will have to be added to this set of constants.
- The problem of dark matter. Given that it almost definitely consists of at least one (and maybe more) new type of massive particle, it stands to reason that more new parameters — potentially even more than one for each new particle type — will need to be added.
- The problem of strong CP-violation. We see CP-violation in the weak nuclear interactions and expect it in the neutrino sector, but we have yet to find it in the strong interactions, even though it is not forbidden. If it exists, there should be more parameters; if it doesn’t, there is likely an additional parameter related to the process that restricts it.

Our Universe is an intricate, amazing place, and yet our greatest hopes of a unified theory — a theory of everything — ought to decrease the number of fundamental constants we need. But the more we learn about the Universe, the more parameters we’re learning it takes to fully describe it. While it’s important to recognize where we are and what it takes, today, to describe the entirety of what’s known, it’s also important to keep searching for a more complete paradigm that not only gives us everything the Universe has to give us, but makes it as simple as possible.
Right now, unfortunately, anything simpler than what we’ve put forth here is too simple to work. Our Universe may not be as elegant as we hoped for after all.
This post first appeared at Forbes, and is brought to you ad-free by our Patreon supporters. Comment on our forum, & buy our first book: Beyond The Galaxy!